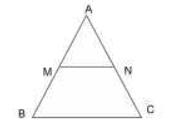
In △ABC, MN||BC, the area
of quadrilateral MBCN=130 sqcm. If AN : NC = 4:5, then the area of △MAN is:
त्रिभुज ABC में, MN||BC है तथा चतुर्भुज MBCN का क्षेत्रफल 130 वर्ग सेमी है | यदि AN : NC = 4 : 5 है, तो त्रिभुज MAN का क्षेत्रफल ज्ञात करें |
45cm2
65cm2
40cm2
32cm2
The correct Answer is:D
Step by step video, text & image solution for In triangle ABC, MN||BC, the area of quadrilateral MBCN=130 sqcm. If AN : NC = 4:5, then the area of triangle MAN is: त्रिभुज ABC में, MN||BC है तथा चतुर्भुज MBCN का क्षेत्रफल 130 वर्ग सेमी है | यदि AN : NC = 4 : 5 है, तो त्रिभुज MAN का क्षेत्रफल ज्ञात करें | by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Similar Questions
In ΔABC, MN||BC, the area of quadrilateral MBCN = 130 sq.cm. If AN : NC = 4:5. then the area of ΔMAN is :
 View Solution
View Solution△ABC is similar to △DEF. The area of △ABC is 100cm2 and the area of △DEF is 49cm2. If the altitude of △ABC=5cm, then the corresponding altitude of △DEF is:
त्रिभुज ABC त्रिभुज DEF के समरूप है | त्रिभुज ABC का क्षेत्रफल 100 वर्ग सेमी है तथा त्रिभुज DEF का क्षेत्रफल 49 वर्ग सेमी है | यदि त्रिभुज ABC की ऊंचाई - 5 सेमी है, तो त्रिभुज DEF की संगत ऊंचाई ज्ञात करें |View Solution
Knowledge Check
- Question 1 - Select One
In △ABC, MN||BC, the area of quadrilateral MBCN=130 sqcm. If AN : NC = 4:5, then the area of △MAN is:
त्रिभुज ABC में, MN||BC है तथा चतुर्भुज MBCN का क्षेत्रफल 130 वर्ग सेमी है | यदि AN : NC = 4 : 5 है, तो त्रिभुज MAN का क्षेत्रफल ज्ञात करें | A45cm2B65cm2C40cm2D32cm2
A45cm2B65cm2C40cm2D32cm2
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If the area of triangle ABC is 136cm2, then the area of triangle BDE is equal to:
ABC और BDE दो समबाहु त्रिभुज हैं जेसे D, BC का मध्य-बिंदु है। यदि त्रिभुज ABC का क्षेत्रफल 136cm2, है, तो त्रिभुज BDE का क्षेत्रफल ज्ञात करेView SolutionIf the height of an equilateral triangle is 12 cm, then what is the area of the triangle?
यदि एक समबाह त्रिभुज की ऊंचाई 12 सेमी है, तो त्रिभुज का क्षेत्रफल कितना है?View SolutionIf the area of an equilateral triangle is 36√3cm2, then the perimeter of the triangle is:
यदि एक समबाहु त्रिभुज का क्षेत्रफल 36√3 वर्ग सेमी है, तो इस त्रिभुज का परिमाप कितना होगा ?View SolutionIn triangle ABC AD, BE, CF are medians. If the area of the triangle DGC is 20cm2 , then the area of triangle AGF + the area of triangle BGF is equal to:
त्रिभुज ABC में, AD, BE और CF मध्यरेखा हैं और G त्रिभुज का केन्द्रक है। यदि त्रिभुज DGC का क्षेत्रफल 20cm2 है, तो त्रिभुज AGF का क्षेत्रफल+ त्रिभुज BGF का क्षेत्रफल किसके बराबर है:View SolutionIn △ABC ,D and E are the points on sides AB and AC, respectively, such that DE || BC. If DE : BC is 3 : 5, then (Area of △ADE): (Area of quadrilateral DECB) is:
त्रिभुज ABC में, D तथा E भुजा AB और AC पर स्थित ऐसे बिंदु हैं कि DE || BC है | यदि DE : BC= 3 : 5, है, तो (△ADE का क्षेत्रफल ): ( DECB का क्षेत्रफल ) ज्ञात करें |View SolutionA circle is inscribed in △ABC, touching AB at P, BC at Q and AC at R. If AR=5 cm, RC= 6 cm and AB=12 cm, then perimeter of △ABC is:
एक वृत्त किसी त्रिभुज ABC में समाहित है जो AB को P पर, BC को Q पर तथा AC को R पर स्पर्श करता है | यदि AR =5 सेमी और AB = 12 सेमी है, तो त्रिभुज ABC का परिमाप ज्ञात करें |View SolutionIn a △ABC, D and E are two points on the sides AB and BC , respectively such that AD : DB = 2: 3 and DE || AC. If the area of △ADE is equal to 18 square cm, then what is the area ( In square cm) of △ABC ?
किसी त्रिभुज ABC में, D और E क्रमश: भुजा AB और BC पर स्थित बिंदु हैं जो इस प्रकार हैं कि AD : DB = 2: 3 है तथा 9DE || AC है | यदि त्रिभुज ADE का क्षेत्रफल 18 वर्ग सेमी के बराबर है, तो त्रिभुज ABC का क्षेत्रफल ( वर्ग सेमी में ) ज्ञात करेंView Solution
