If x1andx2 are the means of two distributions such that x1<x2and¯x is the mean of the combined distribution, then
¯x<¯x1
¯x>¯x2
¯x=¯x1+¯x22
¯x1<¯x<¯x2
The correct Answer is:D
To solve the given problem, we need to analyze the relationship between the means of two distributions, x1 and x2, and the mean of the combined distribution, denoted as ¯x.
1. Understanding the Given Information:
We are given that x1<x2 and that x1 is the mean of the combined distribution. This means that the combined mean ¯x is equal to x1.
2. Expressing the Combined Mean:
The combined mean ¯x of two distributions with means x1 and x2 can be expressed as:
¯x=x1+x22
Since we know ¯x=x1, we can set up the equation:
x1=x1+x22
3. Multiplying Both Sides by 2:
To eliminate the fraction, we multiply both sides of the equation by 2:
2x1=x1+x2
4. Rearranging the Equation:
Now, we can rearrange the equation to isolate x2:
2x1−x1=x2
Simplifying this gives:
x2=x1
5. Analyzing the Inequality:
However, we were given that x1<x2. This means that our earlier assumption must be checked. Since x1 is the mean of the combined distribution, it must be less than the mean of the second distribution x2.
6. Final Conclusion:
Thus, we can conclude that:
x1<x2
This confirms that x1 is less than x2, and since x1 is the mean of the combined distribution, it is consistent with the information provided.
Final Result:
The relationship we derived is:
x1<x2
We are given that x1<x2 and that x1 is the mean of the combined distribution. This means that the combined mean ¯x is equal to x1.
The combined mean ¯x of two distributions with means x1 and x2 can be expressed as:
¯x=x1+x22
Since we know ¯x=x1, we can set up the equation:
x1=x1+x22
To eliminate the fraction, we multiply both sides of the equation by 2:
2x1=x1+x2
Now, we can rearrange the equation to isolate x2:
2x1−x1=x2
Simplifying this gives:
x2=x1
However, we were given that x1<x2. This means that our earlier assumption must be checked. Since x1 is the mean of the combined distribution, it must be less than the mean of the second distribution x2.
Thus, we can conclude that:
x1<x2
This confirms that x1 is less than x2, and since x1 is the mean of the combined distribution, it is consistent with the information provided.
The relationship we derived is:
x1<x2
Topper's Solved these Questions
Similar Questions
If ¯¯¯X1and¯¯¯X2 are the means of two series such that ¯¯¯X1<¯¯¯X2and¯¯¯X is the mean of the combined series, then
View SolutionIf (x)1and(x)2 are the means of two distributions such that (x)1<(x)2andx is the mean of the combined distribution then:- (a) (x)<(x)1 (b) (x)>(x)2 (c) x=(x)1+(x)22 (d) (x)1<(x)<(x)2
View Solution
Knowledge Check
If ¯¯¯¯x2 and¯¯¯¯x2 are the means of two distributions such that ¯¯¯¯x1<¯¯¯¯x2and¯x is the mean of the combined distriubtion, then
A¯x<¯¯¯¯x1B¯x>¯¯¯¯x2C¯x=¯¯¯¯x1+¯¯¯¯x22D¯¯¯¯x1<¯x<¯¯¯¯x2Which one of the following statements is/are correct?
A¯x<¯y<¯zB¯x<¯y<¯zC¯z=¯x<¯y2D¯x<¯z<¯yIfoverline(x_1)andoverline(x_2)aremeansoftwodistributionssuchtˆoverline(x-1) lt overline(x_2)andoverlinex is distribution, then
A¯x<¯¯¯¯x1B¯x>¯¯¯¯x2C¯x=¯¯¯¯x1+¯¯¯¯x22D¯¯¯¯x1<¯x<¯¯¯¯x2
Find the mean of the following distribution: x: 4 6 9 10 15 f: 5 10 10 7 8
View SolutionIf a random variable X has a poisson distribution such that P(X=1)=P(X=2), its mean and variance are
View SolutionIf the mean of the set of numbers x1,x2,...xn is ¯x, then the mean of the numbers xi+2i, 1≤i≤n is
View SolutionIf the mean of the distribution
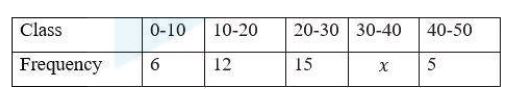
is 24.6, then the value of x is
View SolutionIf the mean of a set of observations x1,x2,…,xn is ¯¯¯X, then the mean of the observations xi+2i,i=1,2,...,n is
View Solution
