If sinx=cosy where x and y are acute angle then what is the relation between x and y ?
x−y=π2
x+y=3π2
x+y=π2
x+y=π4
The correct Answer is:C
To solve the problem where sinx=cosy and x and y are acute angles, we can follow these steps:
Step 1: Use the co-function identity
We know from trigonometric identities that:
sinx=cos(π2−x)
This means that if sinx=cosy, we can express cosy in terms of sine:
cosy=sin(π2−y)
Step 2: Set the equations equal
Since sinx=cosy, we can rewrite it using the co-function identity:
sinx=sin(π2−y)
Step 3: Use the property of sine function
For two angles A and B, if sinA=sinB, then:
A=BorA=π−B
Applying this to our equation:
x=π2−y(since both angles are acute)
Step 4: Rearranging the equation
From the equation x=π2−y, we can rearrange it:
x+y=π2
Conclusion
Thus, the relation between x and y is:
x+y=π2
We know from trigonometric identities that:
sinx=cos(π2−x)
This means that if sinx=cosy, we can express cosy in terms of sine:
cosy=sin(π2−y)
Since sinx=cosy, we can rewrite it using the co-function identity:
sinx=sin(π2−y)
For two angles A and B, if sinA=sinB, then:
A=BorA=π−B
Applying this to our equation:
x=π2−y(since both angles are acute)
From the equation x=π2−y, we can rearrange it:
x+y=π2
Thus, the relation between x and y is:
x+y=π2
Topper's Solved these Questions
- TRIGONOMETRIC RATIO OF SPECIFIC ANGLESBook:LUCENT PUBLICATIONChapter:TRIGONOMETRIC RATIO OF SPECIFIC ANGLESExercise:Exercise 10B
Explore 10 Videos
- TRIGONOMETRIC RATIO OF SPECIFIC ANGLESBook:LUCENT PUBLICATIONChapter:TRIGONOMETRIC RATIO OF SPECIFIC ANGLESExercise:Exercise 10B
Explore 10 Videos
- QUADRILATERAL Book:LUCENT PUBLICATIONChapter:QUADRILATERAL Exercise:Exercise 7B
Explore 6 Videos
Similar Questions
Knowledge Check
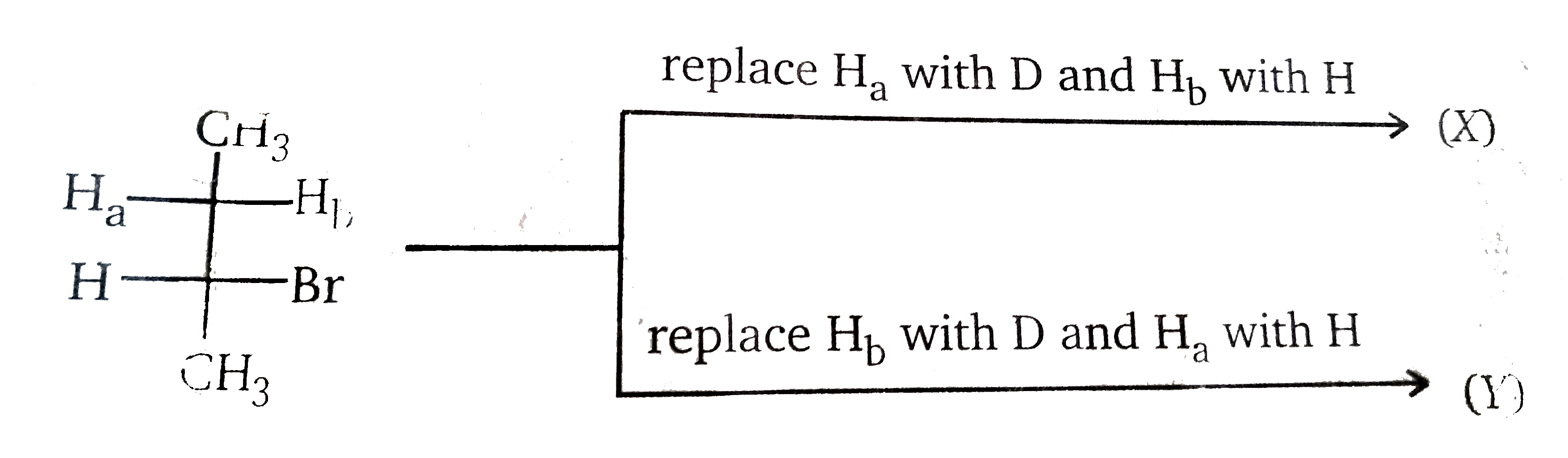
Relation between (X) and (Y) is :AwnantiomersBdiastereomersCE and Z isomerDconstitutional isomerIf sinx−cosx=1, where 'x' is an acute angle ,the value of (sinx+cosx) is :
A0B1C12D2What is the angle between the lines x+y=1 and x−y=1 ?
Aπ6Bπ4Cπ3Dπ2
If sinx+cosy=1 ,x=300 and y is an acute angle. Find the value of y
View SolutionFind the acute angle between the two curves y=x2 and y=(x−3)2
View SolutionThe acute angle between the planes 2x−y+z=6 and x+y+2z=3 is
View SolutionIf sinx-cosx=1,where'x'is an acute angle,the value of (sinx+cosx) is:
View SolutionWhat is the acute angle between the planes x+y+2z=3and−2x+y−z=11?
View Solution