O is point on the line LM A line ON is drawn which is neither coincident with OL nor with OM if ∠MON is one third of ∠LON then ∠MON equal to
A)45∘
B)60∘
C)75∘
D)80∘
The correct Answer is:a
To solve the problem step by step, we will analyze the given information and use some basic properties of angles.
1. Understanding the Setup:
- We have a line LM with points L, O, and M on it.
- Point O is between points L and M.
- A line ON is drawn from point O, which is not coincident with OL or OM.
2. Identifying the Angles:
- We denote:
- ∠LON as the angle formed between line OL and line ON.
- ∠MON as the angle formed between line OM and line ON.
- According to the problem, ∠MON is one-third of ∠LON.
3. Setting Up the Relationship:
- Let ∠LON=3k (where k is a variable representing the angle).
- Therefore, ∠MON=k.
4. Using the Straight Line Property:
- Since points L, O, and M are on a straight line, the sum of angles ∠LON and ∠MON must equal 180 degrees:
∠LON+∠MON=180∘
- Substituting the expressions for the angles:
3k+k=180∘
5. Solving for k:
- Combine like terms:
4k=180∘
- Now, divide both sides by 4:
k=180∘4=45∘
6. Finding the Value of ∠MON:
- Since ∠MON=k, we have:
∠MON=45∘
Conclusion:
The value of ∠MON is 45∘.
- We have a line LM with points L, O, and M on it.
- Point O is between points L and M.
- A line ON is drawn from point O, which is not coincident with OL or OM.
- We denote:
- ∠LON as the angle formed between line OL and line ON.
- ∠MON as the angle formed between line OM and line ON.
- According to the problem, ∠MON is one-third of ∠LON.
- Let ∠LON=3k (where k is a variable representing the angle).
- Therefore, ∠MON=k.
- Since points L, O, and M are on a straight line, the sum of angles ∠LON and ∠MON must equal 180 degrees:
∠LON+∠MON=180∘
- Substituting the expressions for the angles:
3k+k=180∘
- Combine like terms:
4k=180∘
- Now, divide both sides by 4:
k=180∘4=45∘
- Since ∠MON=k, we have:
∠MON=45∘
The value of ∠MON is 45∘.
Topper's Solved these Questions
- LINES AND ANGLES BOOK - LUCENT PUBLICATIONCHAPTER - LINES AND ANGLES EXERCISE - EXERCISE 4B
5 Videos
- LINES AND ANGLES BOOK - LUCENT PUBLICATIONCHAPTER - LINES AND ANGLES EXERCISE - EXERCISE 4B
5 Videos
- INDICES AND SURDS BOOK - LUCENT PUBLICATIONCHAPTER - INDICES AND SURDS EXERCISE - Exercise - 2B
14 Videos
- QUADRILATERAL BOOK - LUCENT PUBLICATIONCHAPTER - QUADRILATERAL EXERCISE - Exercise 7B
6 Videos
Similar Questions
Knowledge Check
L M is a straight line and O is a point on LM .Line ON us drawn not coinciding with OL or OM .If ∠MON is three times ∠LON, then ∠MON is equal to :
A45∘B60∘C105∘D135∘AB is a straight line and O is a point on AB, if a line OC is drawn not coinciding with OA or OB, then ∠AOC and ∠BOC are
AequalBcomplementaryCsupplementaryDtogether equal to 100AB is a straight line and O is a point on AB, if a line OC is drawn not coinciding with OA or OB, then ∠AOC and ∠BOC are
AequalBcomplementaryCsupplementaryDtogether equal to 100
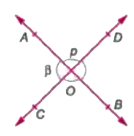
In the given figure, straight lines AB and CD intersect at O.If ∠β = 3∠p,then ∠p is equal to
View SolutionA point on the line x=4 from which the tangents drawn to the circle 2(x2+y2)=25 are at right angles, is
View SolutionThere are n-points (n>2) in each to two parallel lines. Every point on one line is joined to every point on the other line by a line segment drawn within the lines. The number of point (between the lines) in which these segments intersect, is
View SolutionThe slope of the line which bisects the angles in the first and third quadrants is
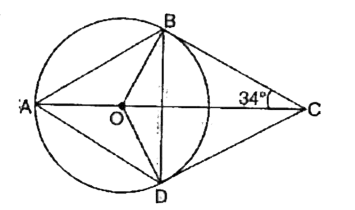
View SolutionIn the diagram, CB and CD are tangents to the circle with centre O. AOC is a straight line and ∠OCB=34∘.∠ABO equals.
View Solution
