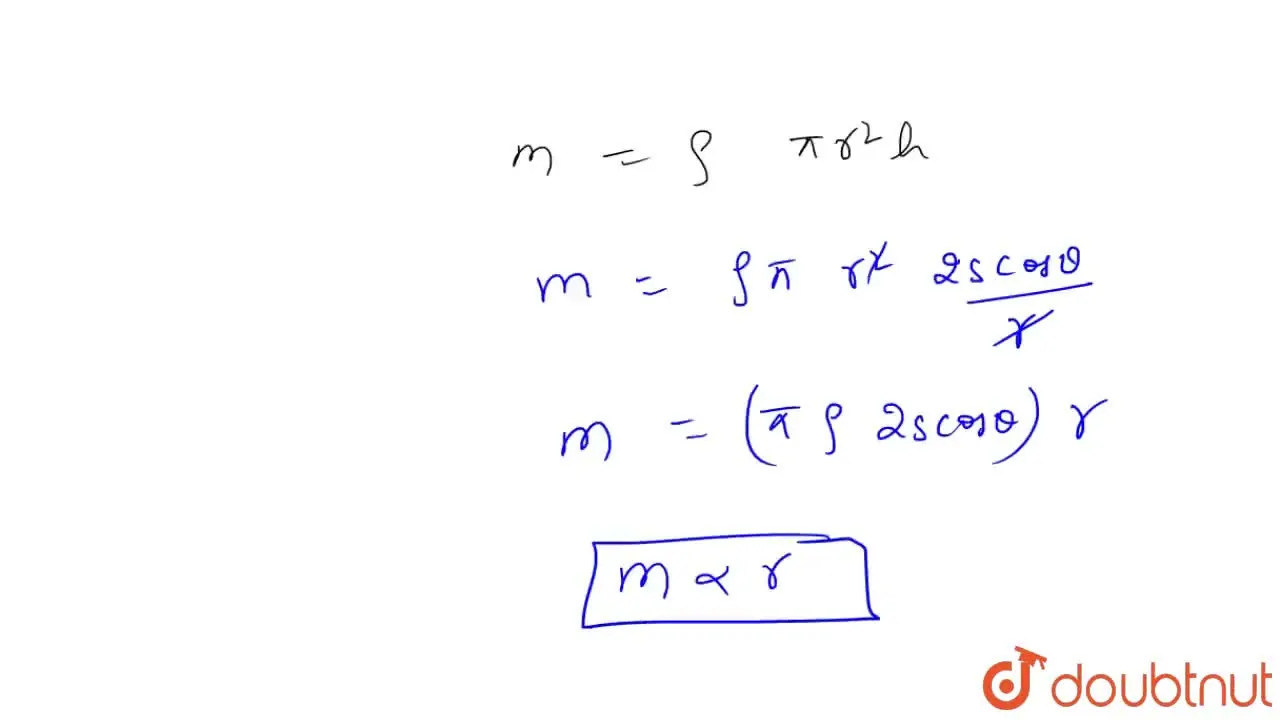A capillary tube of radius r is immersed vertically in a liquid such that liquid rises in it to height h (less than the length of the tube). Mass of liquid in the capillary tube is m. If radius of the capillary tube is increased by 50%, then mass of liquid that will rise in the tube, is
23m
m
32m
94m
The correct Answer is:C
Step by step video, text & image solution for A capillary tube of radius r is immersed vertically in a liquid such that liquid rises in it to height h (less than the length of the tube). Mass of liquid in the capillary tube is m. If radius of the capillary tube is increased by 50%, then mass of liquid that will rise in the tube, is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
The rise of a liquid in a capillary tube is due to :
View SolutionThe rise of a liquid in a capillary tube is due to :
View SolutionThe liquid in the capillary tube will rise if the angle of contact is
View SolutionThe rise of liquid in a capillary tube depends on :
View SolutionA capillary tube of radius r is immersed in a liquid. The liquid rises to a height h. The corresponding mass is m. What mass of water shall rise in the capillary if the radius of the tube is doubled?
View SolutionThe rise of a liquid inn a capillary tube depends on
View SolutionWhen a capillary tube of radius r is immersed in a liquid of density ρ, the liquid rises to a height h in it. If m is the mass of the liquid in the capillary tube, find the potential energy of this mass of the liquid in the tube.
View SolutionIF the diameter of a capillary tube is doubled, then the height of the liquid that will rise is
View SolutionA liquid rises to a height of 3.6 cm in capillary tube of radius r . If the radius of the capillary tube is made 0.75 r, then the height to which the liquid will rise in the capillary tube is
View SolutionObtain an expression for the rise of a liquid in a capillary tube.
View Solution