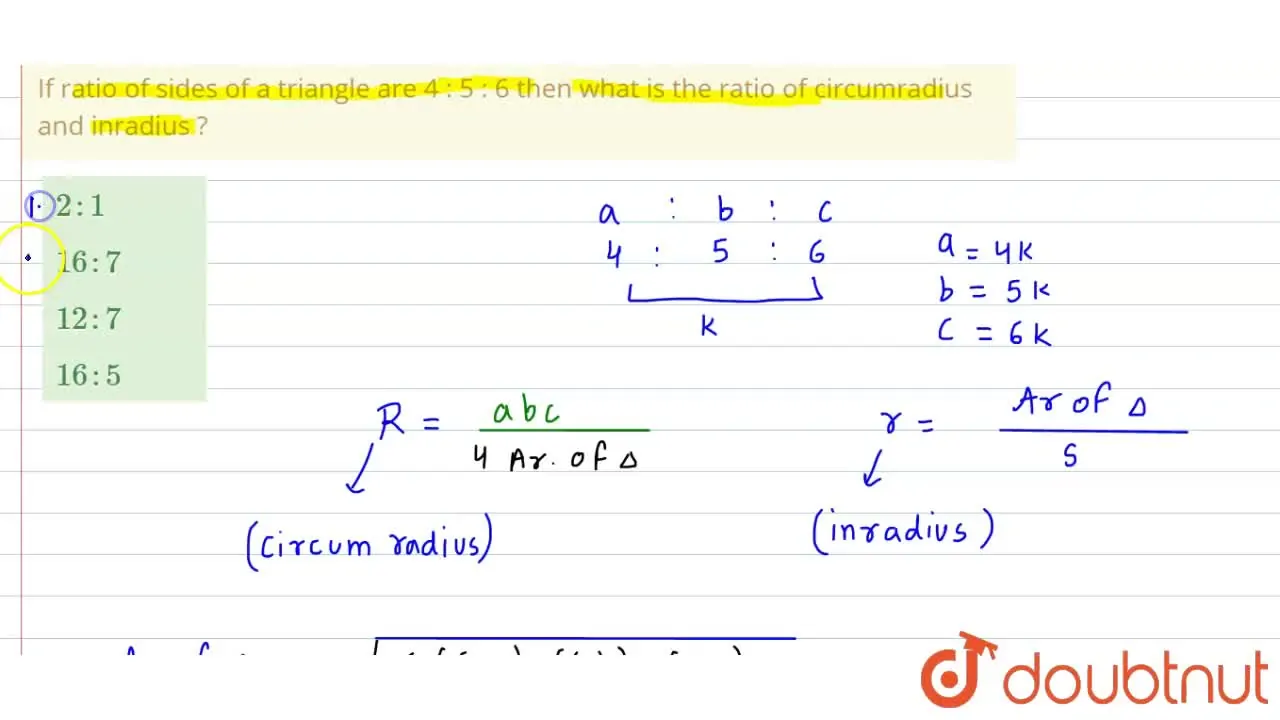
If ratio of sides of a triangle are 4 : 5 : 6 then what is the ratio of circumradius and inradius ?
2:1
16:7
12:7
16:5
The correct Answer is:B
Step by step video, text & image solution for If ratio of sides of a triangle are 4 : 5 : 6 then what is the ratio of circumradius and inradius ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B8videos
CENTRE OF TRIANGLE
- BOOK - LUCENT PUBLICATIONCHAPTER - CENTRE OF TRIANGLEEXERCISE - EXERCISE-6B8videos
CENTRE OF TRIANGLE
- BOOK - LUCENT PUBLICATIONCHAPTER - ALGEBRAIC IDENTITIES EXERCISE - Exercise - 1B28videos
ALGEBRAIC IDENTITIES
- BOOK - LUCENT PUBLICATIONCHAPTER - CIRCLE AND ITS TANGENT LINESEXERCISE - EXERCISE 8B27videos
CIRCLE AND ITS TANGENT LINES
Similar Questions
The ratio of corresponding sides of similar triangles is 5:7, then what is the ratio of their areas?
View SolutionThe ratio of corresponding sides of similar triangles is 3:5, then what is the ratio of their areas.
View SolutionIn a right-angled isosceles triangle, the ratio of the circumradius and inradius is
View SolutionIn a right angled isoceles triangle , the ratio of the circumradius and inradius is
View SolutionIn a triangle ABC if a: b: c= 4:5:6 then ratio between its circumradius and inredius is
View SolutionIn a right - angled isosceles triangle , the ratio of the circumradius and inradius is
View SolutionIf the ratio of sides two squares is 5 :6 , then
What is the ratio in their diagonals?View SolutionIf the ratio of sides two squares is 5 :6 , then
What is the ratio in their perimeter?View SolutionIf the ratio of the sides of two similar triangles is 3 : 5, then the ratio of their areas is :
View SolutionA triangle has its sides in the ratio 4:5:6, then the ratio of circumradius to the inradius of the triangle is
View Solution