Question

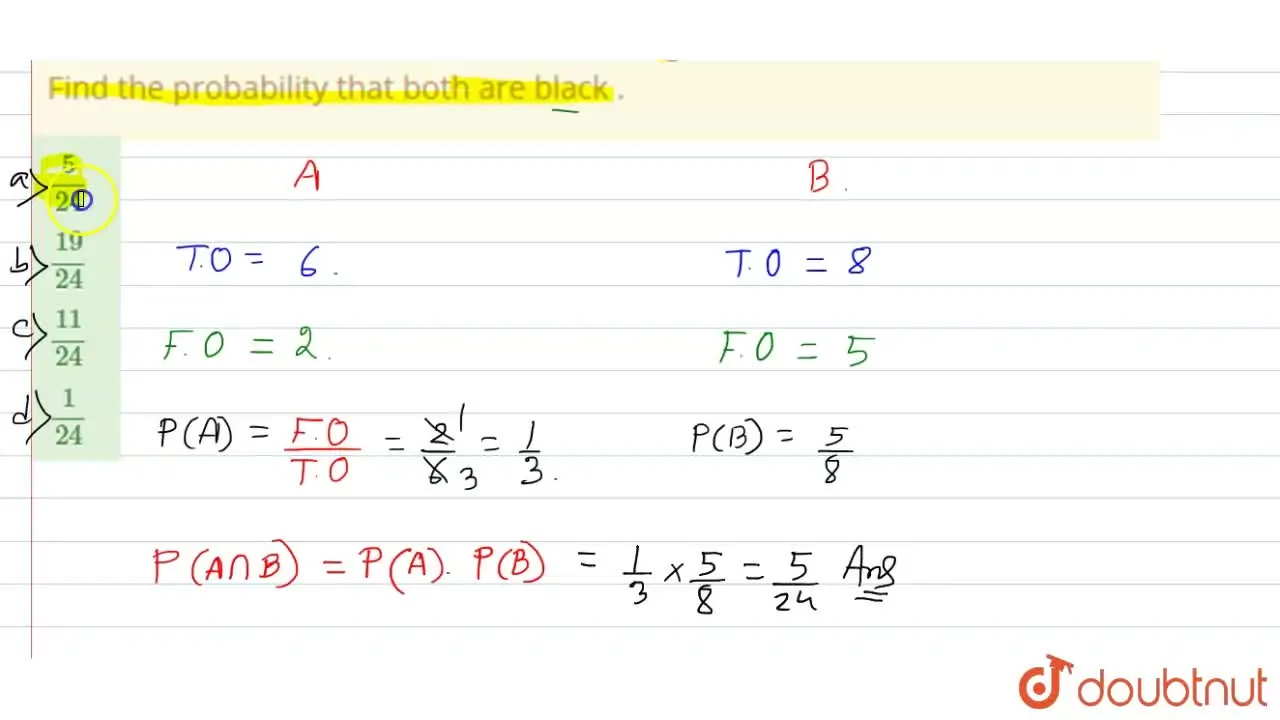
Find bag contains 4 white and 2 black balls .Another contains 3 white and 5 black balls .One ball is drawn from each bag .
Find the probability that both are black .

Options

A. `(5)/(24)`
B. `(19)/(24)`
C. `(11)/(24)`
D. `(1)/(24)`

Answer

A

Explanation

To solve the problem of finding the probability that both balls drawn from two bags are black, we can follow these steps:

Step 1: Identify the contents of each bag
- Bag A contains 4 white balls and 2 black balls.
- Bag B contains 3 white balls and 5 black balls.

Step 2: Calculate the total number of balls in each bag
- For Bag A: Total = 4 (white) + 2 (black) = 6 balls.
- For Bag B: Total = 3 (white) + 5 (black) = 8 balls.

Step 3: Calculate the probability of drawing a black ball from Bag A
- Favorable outcomes for Bag A (black balls) = 2.
- Total outcomes for Bag A = 6.
- Probability of drawing a black ball from Bag A (P(A)) = Favorable outcomes / Total outcomes = 2 / 6 = 1 / 3.

Step 4: Calculate the probability of drawing a black ball from Bag B
- Favorable outcomes for Bag B (black balls) = 5.
- Total outcomes for Bag B = 8.
- Probability of drawing a black ball from Bag B (P(B)) = Favorable outcomes / Total outcomes = 5 / 8.

Step 5: Calculate the probability that both balls drawn are black
Since the events of drawing a ball from Bag A and Bag B are independent, we can use the multiplication rule for independent events:
- Probability of both A and B (P(A ∩ B)) = P(A) * P(B) = (1/3) * (5/8).

Step 6: Perform the multiplication
- P(A ∩ B) = (1/3) * (5/8) = 5 / 24.

Final Answer
The probability that both balls drawn are black is 5/24.

---