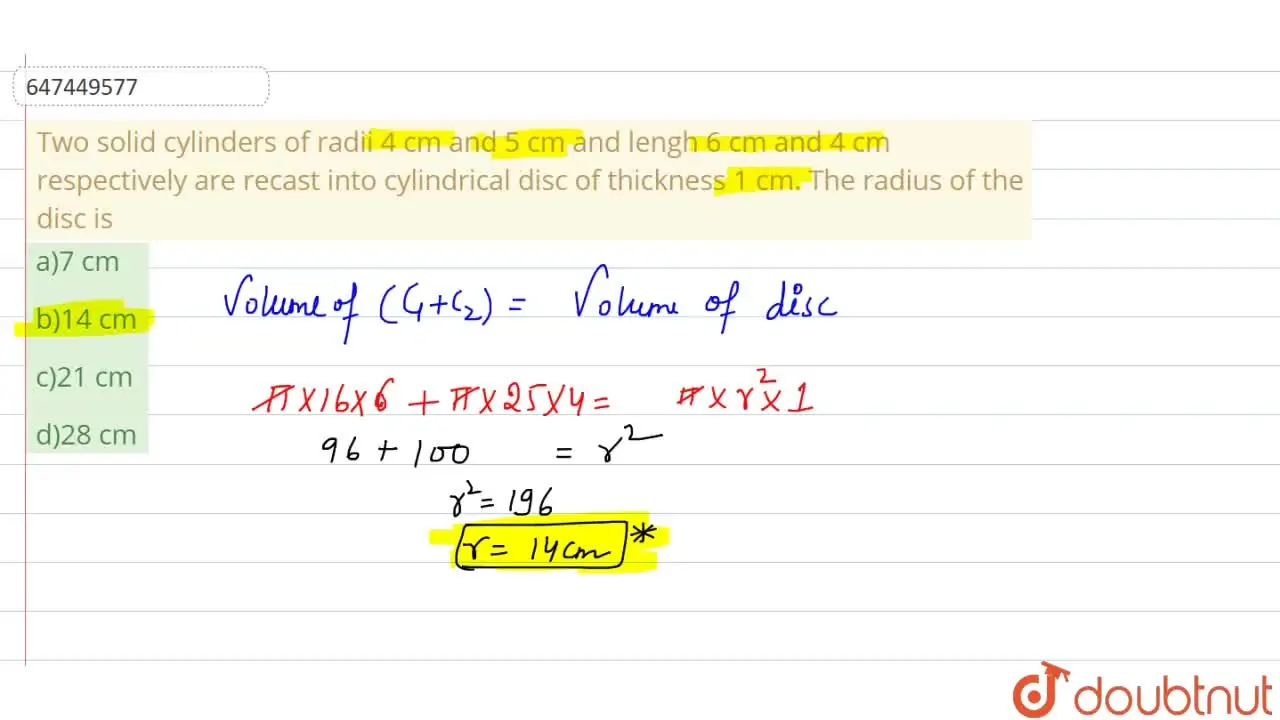
Two solid cylinders of radii 4 cm and 5 cm and lengh 6 cm and 4 cm respectively are recast into cylindrical disc of thickness 1 cm. The radius of the disc is
a)7 cm
b)14 cm
c)21 cm
d)28 cm
The correct Answer is:B
Step by step video, text & image solution for Two solid cylinders of radii 4 cm and 5 cm and lengh 6 cm and 4 cm respectively are recast into cylindrical disc of thickness 1 cm. The radius of the disc is by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE VI47videos
MENSURATION
- BOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE VII60videos
MENSURATION
- BOOK - KIRAN PUBLICATIONCHAPTER - MENSURATIONEXERCISE - TYPE - IV169videos
MENSURATION
- BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself18videos
LCM AND HCF
- BOOK - KIRAN PUBLICATIONCHAPTER - MISCELLANEOUSEXERCISE - TYPE-VI15videos
MISCELLANEOUS
Similar Questions
The volume of a cylinder having radius and height 4 cm and 8 cm respectively, will be :
View SolutionThe radius of the base and height of a metallic solid cylinder are 5 cm and 6 cm respectively. It is melted and recast into a solid cone of the same radius of base. The height of the cone is :
View SolutionTwo cylindrical vessels with radii 15 cm and 10 cm and heights 35 cm and 15 cm respectively are filled with water. If this water is poured into a cylindrical vessel 15 cm in height, then the radius of the vessel is (a) 17.5 cm (b) 18 cm (c) 20 cm (d) 25 cm
View SolutionA solid sphere of radius 3 cm is melted and recast into a cylinder of radius 2cm. The height of the cylinder is :
View SolutionA solid sphere of radius 3 cm is melted and recast into a cylinder of radius 2 cm. The height of the cylinder is :
View SolutionThe radius of the base and height of a metallic solid cylinder are r cm and 6 cm respectively. It is melted and recast into a solid cone of the same radius of base. The height of the cone is (a) 9 cm (b) 18 cm (c) 27 cm (d) 54 cm
View SolutionThe inner and outer radii of a hollow cylinder are 15 cm and 20 cm, respectively. The cylinder is melted and recast into a solid cylinder of the same height. Find the radius of the base of new cylinder.
View SolutionGiven a solid cylinder of radius 10 cm and length 1000 cm a cylinder hold is made into it to obtain a cylindrical shell of uniform thickness and having a volume equal to one-fourth of original volume. The thickness of the cylindrical shell is:
a. √5−2 cm
b. 5√5 cm
c. 4(√5−2) cm
d. 5(2−√3 cmView SolutionHow many solid cylinders of radius 6 cm and height 12 cm can be made by melting a solid shpere of radius 18 cm ?
Radius of the sphere, r=18cm
For cylinder, radius R = 6 cm, height H = 12 cm.
∴ Number of cylinder can be made View Solution
View SolutionA cone and a cylinder of equal radius and height 20 cm are melted and recast into a sphere of diameter 10 cm. Find the radius of the cone.
View Solution