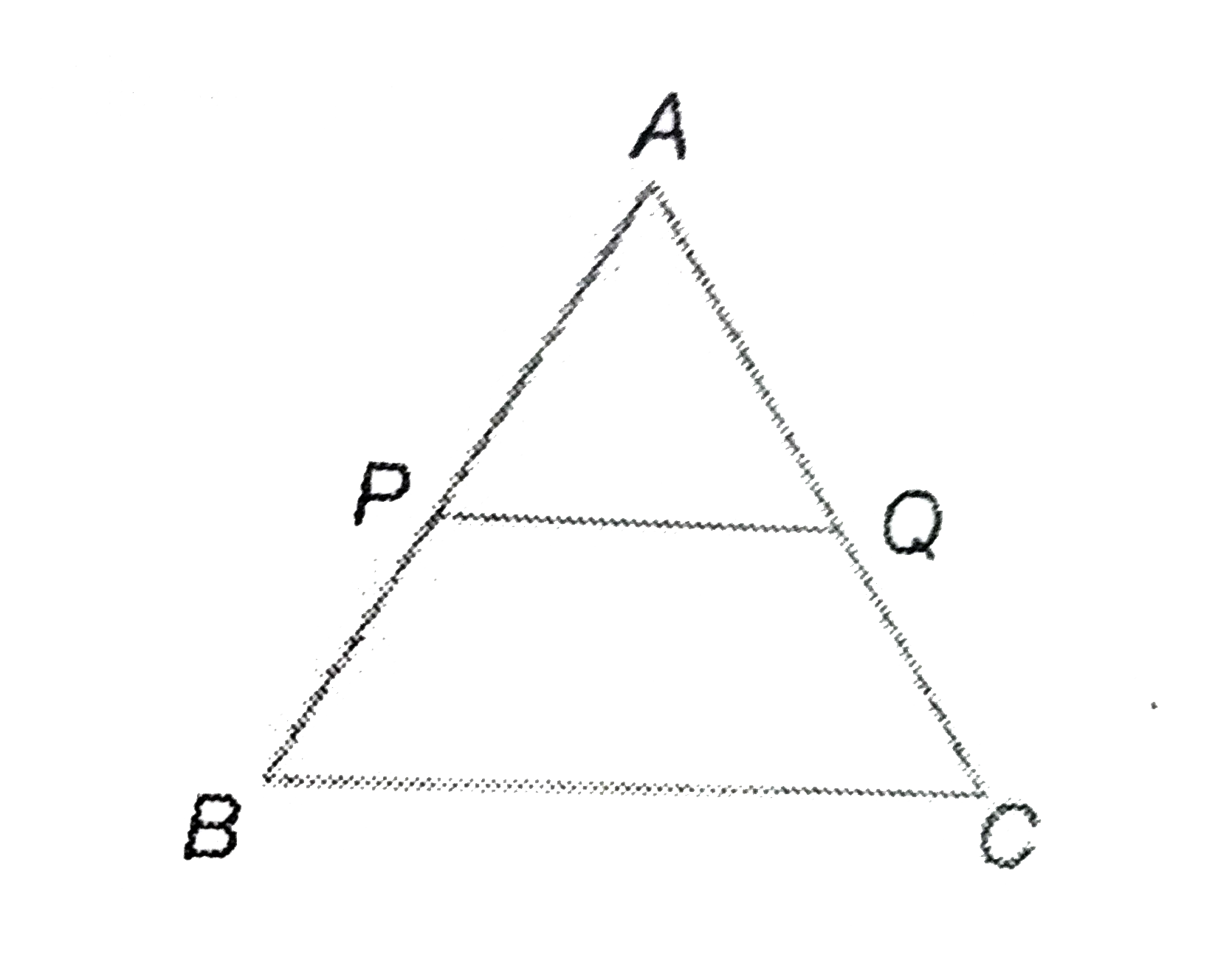
Points P and Q lie on sides AB and AC of triangle ABC respectively such that segment PQ is parallel to side BC. If the ratio of areas of triangle APQ: triangle ABC is 25 : 36, then the ratio PA : PB is
5:6
1:5
6:5
5:1
The correct Answer is:D
To solve the problem, we will use the properties of similar triangles and the relationship between the areas of triangles.
1. Understanding the Problem:
We have triangle ABC with points P and Q on sides AB and AC, respectively, such that line segment PQ is parallel to side BC. We are given that the ratio of the areas of triangle APQ to triangle ABC is 25:36.
2. Using Area Ratio:
The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides. Since PQ is parallel to BC, triangles APQ and ABC are similar.
Area of △APQArea of △ABC=(APAB)2
Given that:
Area of △APQArea of △ABC=2536
3. Setting Up the Equation:
We can set up the equation based on the area ratio:
(APAB)2=2536
4. Taking the Square Root:
To find the ratio of the sides, we take the square root of both sides:
APAB=56
5. Finding the Ratios of PA and PB:
Since AB=PA+PB, we can express PB in terms of PA:
PA=56ABandPB=AB−PA=AB−56AB=16AB
6. Calculating the Ratio PA:PB:
Now we can find the ratio PA:PB:
PA:PB=56AB:16AB=5:1
Final Answer:
The ratio PA:PB is 5:1.
---
We have triangle ABC with points P and Q on sides AB and AC, respectively, such that line segment PQ is parallel to side BC. We are given that the ratio of the areas of triangle APQ to triangle ABC is 25:36.
The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding sides. Since PQ is parallel to BC, triangles APQ and ABC are similar.
Area of △APQArea of △ABC=2536
We can set up the equation based on the area ratio:
(APAB)2=2536
To find the ratio of the sides, we take the square root of both sides:
APAB=56
Since AB=PA+PB, we can express PB in terms of PA:
PA=56ABandPB=AB−PA=AB−56AB=16AB
Now we can find the ratio PA:PB:
PA:PB=56AB:16AB=5:1
The ratio PA:PB is 5:1.
Topper's Solved these Questions
- MENSURATIONBook:KIRAN PUBLICATIONChapter:MENSURATIONExercise:TYPE - III
Explore 21 Videos
- MENSURATIONBook:KIRAN PUBLICATIONChapter:MENSURATIONExercise:TYPE - IV
Explore 169 Videos
- MENSURATIONBook:KIRAN PUBLICATIONChapter:MENSURATIONExercise:Test Yourself
Explore 28 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
- MISCELLANEOUSBook:KIRAN PUBLICATIONChapter:MISCELLANEOUSExercise:TYPE-VI
Explore 15 Videos
Similar Questions
Suppose ABC is a triangle with AB of unit length. D and E are the points lying on AB and AC respectively such that BC and DE are parallel. If the area of triangle ABC is twice the area of triangle ADE, then the length of AD is
View SolutionIn the following figure, PQ is parallel to BC and PQ:BC=1:3. If the area of the triangle ABC is 144cm2, then what is the area of the triangle APQ?
View Solution
Knowledge Check
Points P and Q lie on the sides AB and AC of triangle ABC respectivey such that line segment PQ is parallel to side BC. If the ratio of AP : PB is 1 : 4 and area of ΔAPQ is 4 square cm., what is the area of trapezium PQCB ?
A60 sq. cmB16 sq. cmC96 sq. cmD21 sq. cmIf D, E and F are the mid-points of sides BC, CA and AB respectively of △ABC, then the ratio of the areas of △DEF to the area of △ABC is :
A1:4B1:2C1:3D2:3In the following figure, PQ is parallel to BC and PQ:BC=1:3. If the area of the triangle ABC is 144cm2, then what is the area of the triangle APQ?
 A48cm2B36cm2C16cm2D9cm2
A48cm2B36cm2C16cm2D9cm2
Three points P, Q, R lie on the side BC of triangle ABC such that BP = PQ = QR = RC. If G be centroid of ΔABC then what is ratio of areas of ΔPGRandΔABC.
View SolutionIf K is a point on the side BC of an equilateral triangle ABC and if ∠BAK=15∘, then the ratio of AKAB is
View SolutionIf a line intersects sides AB and AC of a ΔABCat D and E respectively and is parallel to BC, prove that ADAB=AEAC
View SolutionLet P, Q, R be the mid-points of sides AB, BC, CA respectively of a triangle ABC. If the area of the triangle ABC is 5 square units, then the area of the triangle PQR is
View SolutionIn Figure the line segment XY is parallel to side AC of ΔABCand it divides the triangle into two parts of equal areas. Find the ratio AXAB.
View Solution