A rectangle with one side of length 4 cm. is inscribed in a circle of diameter 5 cm. Find, the area of the rectangle.
The correct Answer is:B
To find the area of the rectangle inscribed in a circle, we will follow these steps:
Step 1: Understand the dimensions of the rectangle and the circle.
We know that one side of the rectangle (length) is 4 cm, and the diameter of the circle is 5 cm.
Step 2: Calculate the radius of the circle.
The radius (r) of the circle is half of the diameter.
r=diameter2=5cm2=2.5cm
Step 3: Use the Pythagorean theorem to find the other side (breadth) of the rectangle.
In a rectangle inscribed in a circle, the diagonal of the rectangle is equal to the diameter of the circle. We can denote the length of the rectangle as l=4cm and the breadth as b. The relationship can be expressed using the Pythagorean theorem:
l2+b2=d2
where d is the diameter of the circle. Substituting the known values:
42+b2=52
16+b2=25
Step 4: Solve for the breadth (b).
Rearranging the equation gives:
b2=25−16
b2=9
Taking the square root of both sides, we find:
b=3cm
Step 5: Calculate the area of the rectangle.
The area (A) of the rectangle is given by the formula:
A=l×b
Substituting the values we found:
A=4cm×3cm=12cm2
Final Answer:
The area of the rectangle is 12cm2.
---
We know that one side of the rectangle (length) is 4 cm, and the diameter of the circle is 5 cm.
The radius (r) of the circle is half of the diameter.
r=diameter2=5cm2=2.5cm
In a rectangle inscribed in a circle, the diagonal of the rectangle is equal to the diameter of the circle. We can denote the length of the rectangle as l=4cm and the breadth as b. The relationship can be expressed using the Pythagorean theorem:
l2+b2=d2
where d is the diameter of the circle. Substituting the known values:
42+b2=52
16+b2=25
Rearranging the equation gives:
b2=25−16
b2=9
Taking the square root of both sides, we find:
b=3cm
The area (A) of the rectangle is given by the formula:
A=l×b
Substituting the values we found:
A=4cm×3cm=12cm2
The area of the rectangle is 12cm2.
Topper's Solved these Questions
- MENSURATIONBook:KIRAN PUBLICATIONChapter:MENSURATIONExercise:TYPE - III
Explore 21 Videos
- MENSURATIONBook:KIRAN PUBLICATIONChapter:MENSURATIONExercise:TYPE - IV
Explore 169 Videos
- MENSURATIONBook:KIRAN PUBLICATIONChapter:MENSURATIONExercise:Test Yourself
Explore 28 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
- MISCELLANEOUSBook:KIRAN PUBLICATIONChapter:MISCELLANEOUSExercise:TYPE-VI
Explore 15 Videos
Similar Questions
Knowledge Check
A rectangle with one side of length 4 cm. is inscribed in a circle of diameter 5 cm. Find, the area of the rectangle.
A21cm2 / सेमी2B12cm2 / सेमी2C4cm2 / सेमी2D3cm2 / सेमी2A rectangle of maximum area is drawn inside a circle of diameter 5 cm. What is the maximum area of such a rectangle?
A25cm2B12cm2C12.5cm2DNone of theseIn the given figure, ABCD is a rectangle inscribed in a quadrant of a circle of radius 10 cm. If AD=√25 cm then area of the rectangle is
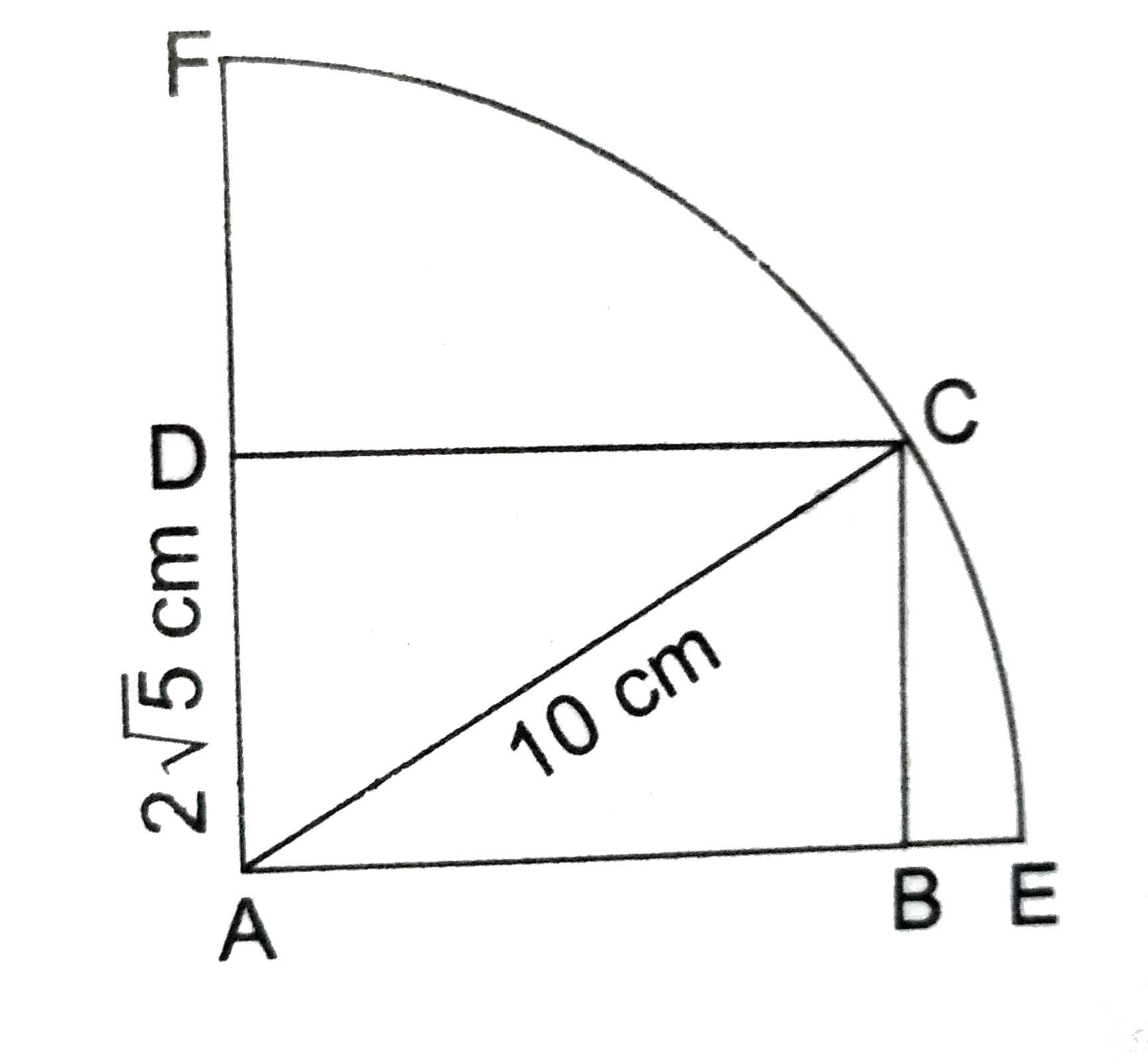 A32cm2B40cm2C44cm2D48cm2
A32cm2B40cm2C44cm2D48cm2
Perimeter of a square and a rectangle is same. If a side of the square is 15cm one side of the rectangle is 18cm, find the area of the rectangle.
View SolutionA rectangle in inscribed in a circle of radius 5 cm. if the breadth of the rectangle is 6 cm. then find the length of the rectangle.
View SolutionThe circumference of the circle is 2 cm more than to the perimeter of the rectangle. The length of the rectangle is equal to the diameter of the circle and the breadth of the rectangle is equal to the radius of the circle. Then find the radius of the circle.
View SolutionFind the Area of Shaded Region bounded between two semicircles drawn on side of length 28cm of a rectangle as diameter. The other side of the rectangle is 14 cm long. Semi-Circles Rectangle Shaded Area
View SolutionMaximum area of the rectangle inscribed in a circle of radius 10 cms is
View Solution