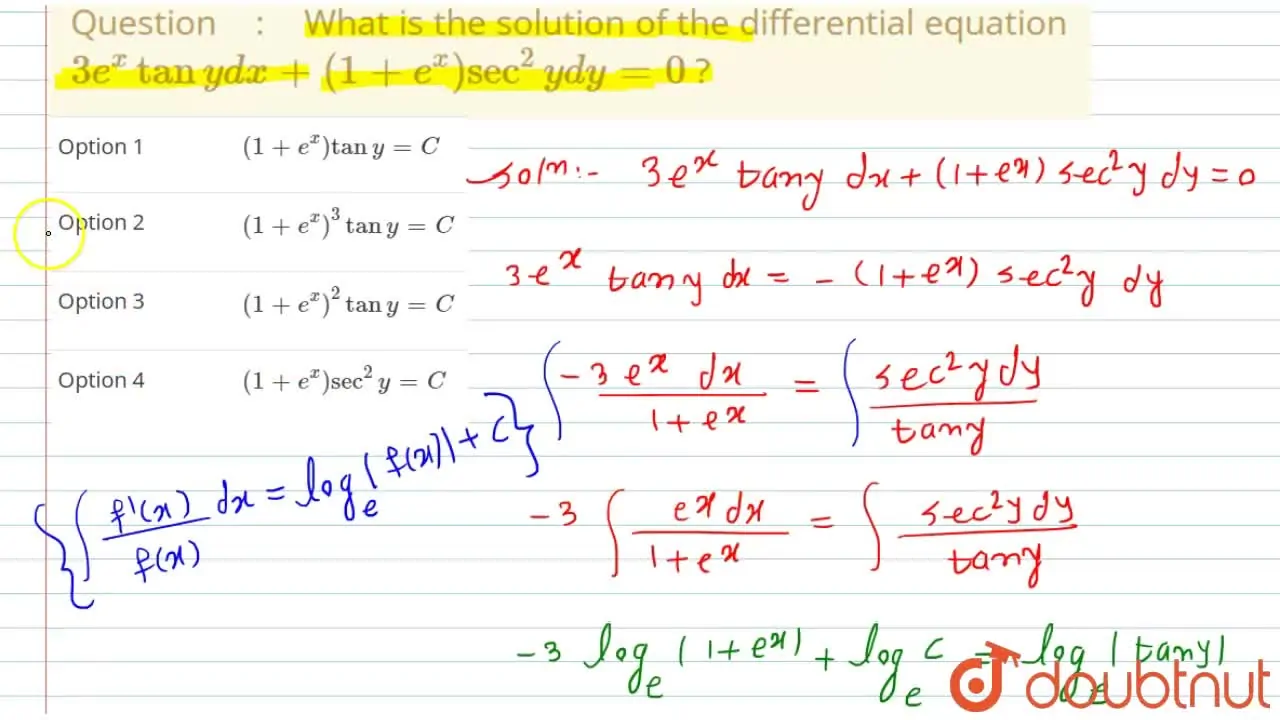
What is the solution of the differential equation 3extanydx+(1+ex)sec2ydy=0 ?
siny=C(1−ex)
cosy=C(ex−1)
tany=C(ex−1)
None of these
The correct Answer is:D
Step by step video, text & image solution for What is the solution of the differential equation 3e^(x) tan y dx + (1 + e^(x)) sec^(2) y dy = 0 ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
what is the solution of the differential equaiton
3extanydx+(1+ex)sec2ydy=0 ?View SolutionSolve the differential equation extanydx+(1−ex)sec2ydy=0
View SolutionWhat is the solution of the differential equation 3extanydx+(1+ex)sec2ydy=0 ?
View SolutionThe solution of the differential equation 3extanydx+(1+ex)sec2ydy=0 is
View SolutionFind the particular solution of the differential equation 3extanydx+(1+ex)sec2ydy=0, when x= 0, y=π
View SolutionWhat is the solution of the differential equation
dydx=sec(x+y) ?View SolutionWhat is the solution of the differential equation dydx=sec(x+y) ?
View SolutionThe solution of the differential equation dydx−ytanx=exsecx is
View SolutionThe solution of the differential equation exdx+eydy=0 is
View SolutionThe solution of the differential equation exdx+eydy=0 is
View Solution