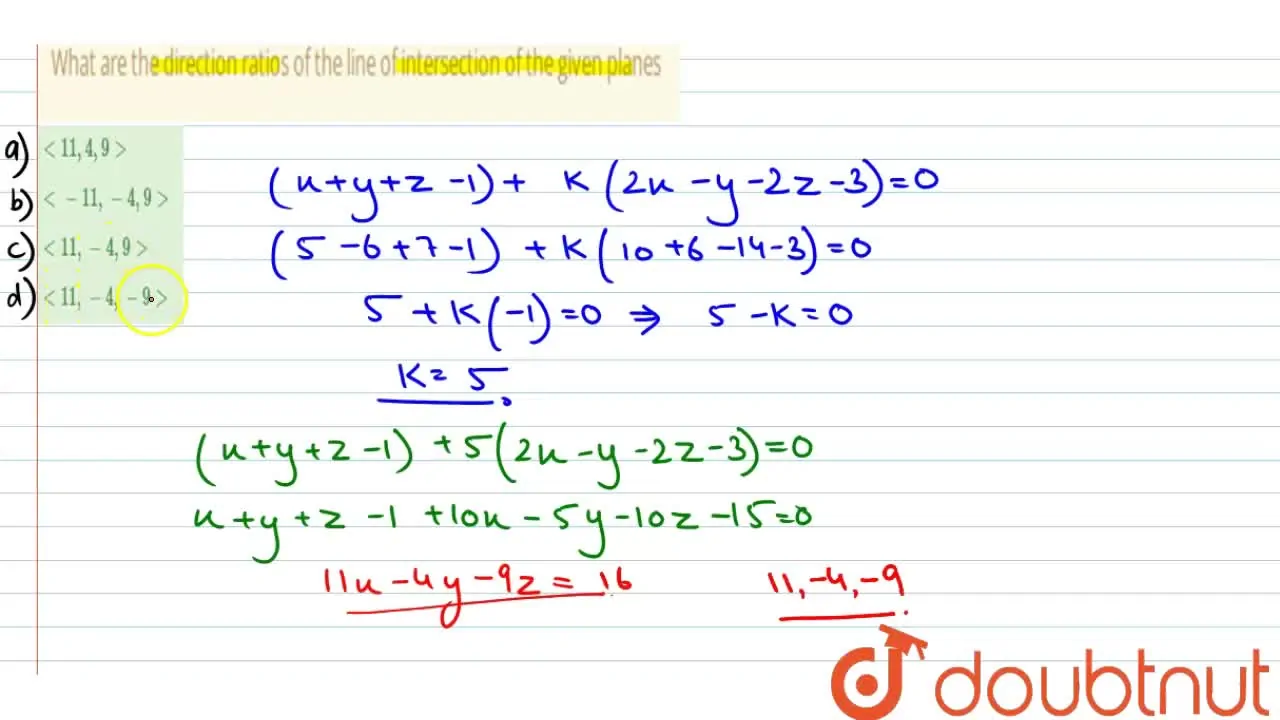
A line L passes through the point P(5,−6,7) and is parallel to the planes x+y+z=1and2x−y−2z=3.
What are the direction ratios of the line of intersection of the given planes
<11,4,9>
<−11,−4,9>
<11,−4,9>
<11,−4,−9>
The correct Answer is:C
Step by step video, text & image solution for A line L passes through the point P(5,-6,7) and is parallel to the planes x+y+z=1 and 2x-y-2z=3. What are the direction ratios of the line of intersection of the given planes by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
A line L passes through the point P(5,−6,7) and is parallel to the planes x+y+z=1and2x−y−2z=3.What is the equation of the line L ?
View SolutionWhat are the direction ratios of the line of intersection of the planes x=3z+4andy=2z−3?
View SolutionA plane P passes through the line of intersection of the planes 2x−y+3z=2,x+y−z=1 and the point (1,0,1).
What are the direction ratios of the line of intersection of the given planes ?View SolutionA plane P passes through the line of intersection of the planes 2x−y+3z=2,x+y−2=1 and the point (1,0,1). What are the direction ratios of the line of intersection of the given planes? What is the equation of the plane P? If the plane P touches the sphere x2+y2+z2=r2, then what is r equal to?
View SolutionA plane P passes through the line of intersection of the planes 2x−y+3z=2.x+y−z=1 and the point (1,0,1)
What are the direction ration of the line of intersection of the given planesView SolutionA straight line passes through (1, -2, 3) and perpendicular to the plane 2x+3y−z=7.
What are the direction ratios of normal to plane?View SolutionEquation of a line passing through (1,-2,3) and parallel to the plane 2x+3y+z+5=0 is
View SolutionWhat are the direction ratios of the line determined by the planes x−y+2z=1andx+y−z=3?
View SolutionA straight line passes through (1,−2,3) and perpendicular to the plane 2x+3y−z=7
What are the direction rations of normal to plane ?View SolutionEquation of the line passing through (1,1,1) and parallel to the plane 2x+3y+z+5=0 is
View Solution