The autorickshaw fare consists of a fixed charge together with the charge for the distance covered. For a journey of 10 km, the charge paid is 85 and for a journey of 15 km, the charge paid is 120. The fare for a journey of 25 km will be
175
190
180
225
The correct Answer is:B
To solve the problem of finding the fare for a journey of 25 km based on the given information, we can set up a linear equation. The fare consists of a fixed charge plus a variable charge per kilometer traveled.
1. Define Variables:
Let F be the fixed charge and C be the charge per kilometer.
2. Set Up Equations:
From the information provided:
- For a journey of 10 km, the total fare is 85:
F+10C=85(Equation 1)
- For a journey of 15 km, the total fare is 120:
F+15C=120(Equation 2)
3. Solve the Equations:
We can solve these two equations simultaneously.
- First, subtract Equation 1 from Equation 2:
(F+15C)−(F+10C)=120−85
This simplifies to:
5C=35
Dividing both sides by 5 gives:
C=7
4. Find the Fixed Charge:
Now that we have C, we can substitute it back into either equation to find F. Using Equation 1:
F+10(7)=85
Simplifying this gives:
F+70=85
Therefore:
F=85−70=15
5. Calculate the Fare for 25 km:
Now that we have both F and C:
Fare for 25 km=F+25C
Substituting the values:
Fare for 25 km=15+25(7)=15+175=190
Final Answer:
The fare for a journey of 25 km will be 190.
Let F be the fixed charge and C be the charge per kilometer.
From the information provided:
- For a journey of 10 km, the total fare is 85:
F+10C=85(Equation 1)
- For a journey of 15 km, the total fare is 120:
F+15C=120(Equation 2)
We can solve these two equations simultaneously.
(F+15C)−(F+10C)=120−85
This simplifies to:
5C=35
Dividing both sides by 5 gives:
C=7
Now that we have C, we can substitute it back into either equation to find F. Using Equation 1:
F+10(7)=85
Simplifying this gives:
F+70=85
Therefore:
F=85−70=15
Now that we have both F and C:
Fare for 25 km=F+25C
Substituting the values:
Fare for 25 km=15+25(7)=15+175=190
The fare for a journey of 25 km will be 190.
Topper's Solved these Questions
- LINEAR EQUATIONSBOOK - DISHA PUBLICATIONCHAPTER - LINEAR EQUATIONSEXERCISE - STANDARD LEVEL
19 Videos
- LINEAR EQUATIONSBOOK - DISHA PUBLICATIONCHAPTER - LINEAR EQUATIONSEXERCISE - EXPERT LEVEL
13 Videos
- LINEAR EQUATIONSBOOK - DISHA PUBLICATIONCHAPTER - LINEAR EQUATIONSEXERCISE - TEST YOUSELF
15 Videos
- INTERESTBOOK - DISHA PUBLICATIONCHAPTER - INTERESTEXERCISE - Test Yourself
15 Videos
- LOGARITHMSBOOK - DISHA PUBLICATIONCHAPTER - LOGARITHMSEXERCISE - Test Yourself
15 Videos
Similar Questions
The taxi charges in a city comprise of a fixed charge together with the charge for the distance covered. For a journey of 10 km the charge paid is Rs 75 and for a journey of 15 km the charge paid is Rs 110. What will a person have to pay for travelling a distance of 25 km?
View SolutionThe car hire charges in a city comprise of a fixed charges together with the charge for the distance covered. For a journey of 12 km, the charge paid is Rs 89 and for a journey of 20 km, the charge paid is Rs 145. What will a person have to pay for travelling a distance of 30 km?
View Solution
Knowledge Check
A private taxi company charges a fixed charge along with a per kilometre charge based on the distance covered. For a journey of 24 km, the charges paid are Rs. 368 and for a journey of 32 km, the charges paid Rs. 464. How much will a person have to pay for travelling a distance of 15 km?
ARs. 180BRs. 260CRs. 290DRs. 280
The taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 20 km, the charge paid is 205 and for a distance of 25 km, the charge paid is 255. Find the fixed charges and the charge per km. How much does a person have to pay for covering a distance of 50 km? Sol. Let the fixed charges be x and charge per km be y.
View SolutionThe taxi charges in a city comprise of a fixed charge, together with the charge of the distance covered. For a journey of 16 km, the charges paid are Rs 156 and for a journey of 24 km, the charges paid are Rs 204. What will a person have to pay for travelling a distance of 30 km? (a) Rs 236 (b) Rs 240 (c) Rs 248 (d) Rs 252
View Solution[" (IV) The taxi charges in a city consist of a fixed charge together with the charge for the "],[" distance covered.For a distance of "10km" ,the charge paid is "₹105" and for a "],[" journey of "15km" ,the charge paid is "₹155" .What are the fixed charges and the "],[" charge per km? How much does a person have to pay for travelling a distance of "],[25km" ? "]
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
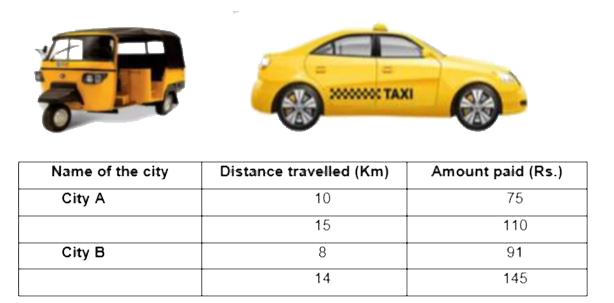
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
The graph of lines representing the conditions are: (situation 2)
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
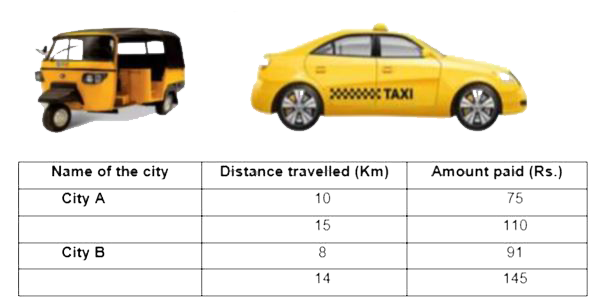
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
If the fixed charges of auto rickshaw be Rs x and the running charges be Rs y km/hr, the pair of linear equations representing the situation is
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
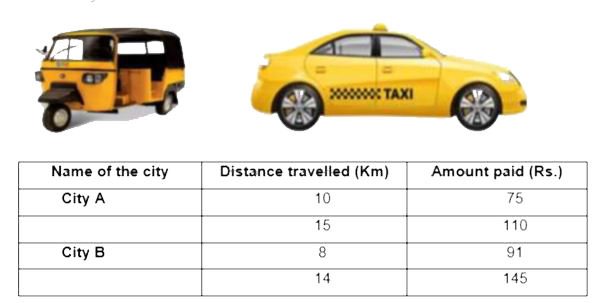
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
Refer to situation 1:
A person travels a distance of 50km. The amount he has to pay is
View SolutionIt is common that Governments revise travel fares from time to time based on various factors such as inflation ( a general increase in prices and fall in the purchasing value of money) on different types of vehicles like auto, Rickshaws, taxis, Radio cab etc. The auto charges in a city comprise of a fixed charge together with the charge for the distance covered. Study the following situations
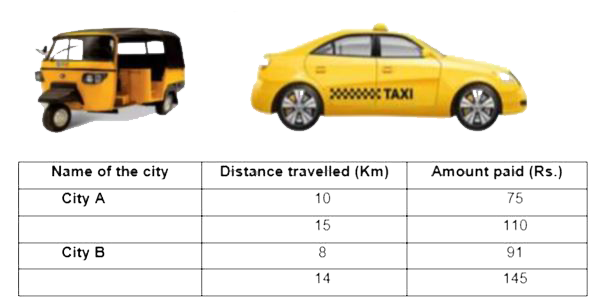
Situation 1: In city A, for a journey of 10 km, the charge paid is Rs 75 and for a journey of 15 km, the charge paid is Rs 110.
Situation 2: In a city B, for a journey of 8km, the charge paid is Rs91 and for a journey of 14km, the charge paid is Rs 145.
Refer to situation 2:
What will a person have to pay for travelling a distance of 30km?
View Solution
