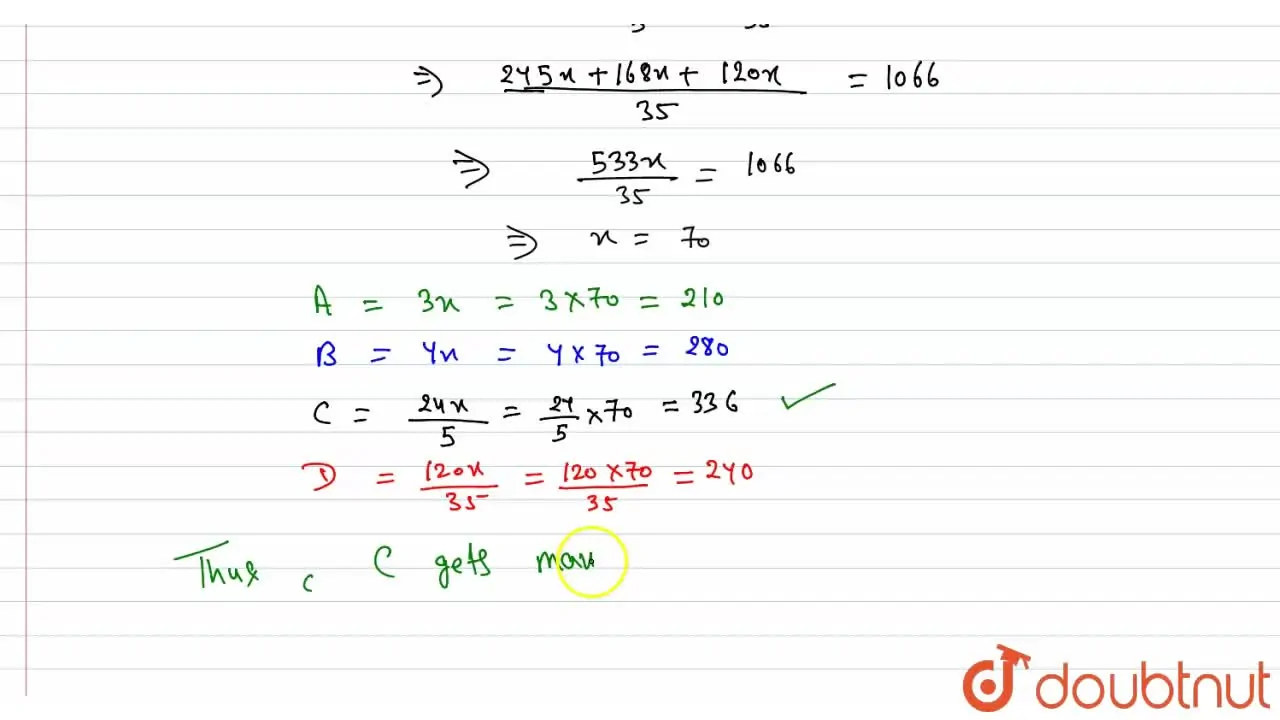
If '1066 is divided among A, B, C and D such that A : B = 3 : 4, B : C = 5 : 6 and C : D = 7 : 5, who will get the maximum?
B
A
C
D
The correct Answer is:C
Step by step video, text & image solution for If '1066 is divided among A, B, C and D such that A : B = 3 : 4, B : C = 5 : 6 and C : D = 7 : 5, who will get the maximum? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
- BOOK - DISHA PUBLICATIONCHAPTER - RATIO,PROPORTION AND VARIATIONEXERCISE - Practice Exercises ( Standard Level)26videos
RATIO,PROPORTION AND VARIATION
- BOOK - DISHA PUBLICATIONCHAPTER - RATIO,PROPORTION AND VARIATIONEXERCISE - Practice Exercises (Expert Level)12videos
RATIO,PROPORTION AND VARIATION
- BOOK - DISHA PUBLICATIONCHAPTER - RATIO,PROPORTION AND VARIATIONEXERCISE - Test Yourself15videos
RATIO,PROPORTION AND VARIATION
- BOOK - DISHA PUBLICATIONCHAPTER - QUADRATIC AND CUBIC EQUATIONSEXERCISE - Test Yourself15videos
QUADRATIC AND CUBIC EQUATIONS
- BOOK - DISHA PUBLICATIONCHAPTER - SET THEORYEXERCISE - Test Yourself15videos
SET THEORY
Similar Questions
If Rs. 1066 are divided among A, B, C and D such that A:B=3:4, B:C=5:6 and C:D=7:5, who will get the maximum? A b. B c. C d. D
View SolutionIf A:B=3:4,B:C=5:7 and C:D=8:9 then A:D is equal to
View SolutionRs. 2420 were divided among a,B C so that A:B=5:4andB:C=9:10 then C gets
View SolutionIf A : B = 1 : 2, B : C = 3 : 4 and C : D = 5 : 6, then find the value of D : C : B.
View SolutionIf A:B=1:2,B:C=3:4andC:D=5:6 find D: C : B :A
View SolutionIf A : B = 5 : 2, B : C = 2 : 3 and C: D = 5: 3 , then the ratio A: B : C : D is
View SolutionDivide 581 among A,B,C so that 4A=5B=7C
View SolutionIf A:B = 3:4, B: C= 5:7 and C:D = 8: 9, then, the ratio A:D is :
View SolutionIf A, B, C and D are four numbers such that A : B = 2 : 3, B : C = 4 : 5, C : D = 5 : 8, then A : D is equal to
View SolutionA sum of ₹14460 is divided among A, B, C and D such that the ratio of share of A and B is 3 : 5, that of B and C is 6 : 7 and that of C and D is 14 : 15. What is the difference between the shares of A and C?
View Solution