Given A=(1, 1) and AB is any line through it cutting the x-axis at B. If AC is perpendicular to AB and meets the y-axis in C, then the equation of the locus of midpoint P of BC is Solution in Malayalam
x+y=1
x+y=2
x+y= 2xy
2x + 2y = 1
The correct Answer is:A
Step by step video & text solution for Given A=(1, 1) and AB is any line through it cutting the x-axis at B. If AC is perpendicular to AB and meets the y-axis in C, then the equation of the locus of midpoint P of BC is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
the line xa−yb=1 cuts the x-axes at P.the equation of the line passes through point P and perpendicular to the line is:
View SolutionLet A be any point on the x-axis and B=(2,3). The perpendicular at A to the line AB meets the y-axis at C. Then the locus of midpoint of segment AC as A moves is given by
View SolutionThe line 3x+2y=24 meets the y-axis at A and the x-axis at B. The perpendicular bisector of AB meets the line through (0,-1) parallel to the x-axis at C. If the area of triangle ABC is A, then the Value of A/13 is _________
View SolutionGiven A-=(1,1) and AB is any line through it cutting the x-axist at B. If AC is perpendicular to AB and meets the y-axis in c, then the equatio of the locus of midpoint P of BC is
View SolutionThe line xa−yb=1 cuts the x-axis at P. The equation of the line through P and perpendicular to the given line is
View SolutionLet A be any variable point on the X-axis and B the point (2,3). The perpendicular at A to the line AB meets the Y-axis at C. Then the locus of the mid-point of the segment AC as A moves is given by the equation
View SolutionA line AB meets X-axis at A and Y-axis at B. P(4, -1) divides AB in the ratio 1:2.
(ii) Find the equation of the line through P and perpendicular to AB
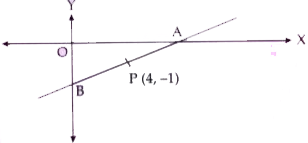
View SolutionA straight line through the point (1,1) meets the X-axis at A and Y-axis at B. The locus of the mid-point of AB is
View Solution
