An object is put in turn, in three liquids having different densities . The object floats with 3/5,2/9 and 8/11 parts of its volume inside the liquid surface in liquids of densities ρ1,ρ2 and ρ3 respectively . Which of the following is correct ?
ρ1>ρ2>ρ3
ρ3<ρ1<ρ2
ρ3>ρ1>ρ2
ρ1<ρ3<ρ2
The correct Answer is:B
To solve the problem, we need to analyze the relationship between the volume of the object submerged in each liquid and the densities of those liquids. Here's a step-by-step solution:
Step 1: Understand the concept of buoyancy
When an object floats in a liquid, the weight of the liquid displaced by the submerged part of the object is equal to the weight of the object. This is known as Archimedes' principle.
Step 2: Set up the relationship
Let:
- V0 = volume of the object submerged in the liquid
- V = total volume of the object
- ρ = density of the liquid
- g = acceleration due to gravity (constant)
According to Archimedes' principle, the thrust (upward force) is equal to the weight of the displaced liquid:
Thrust=V0⋅ρ⋅g
Since the object is in equilibrium, this thrust equals the weight of the object:
V0⋅ρ⋅g=m⋅g
Where m is the mass of the object. Since g is constant, we can simplify this to:
V0⋅ρ=m
Step 3: Express V0 in terms of ρ
From the equation above, we can express the volume submerged V0 as:
V0=mρ
This shows that the volume submerged is inversely proportional to the density of the liquid.
Step 4: Analyze the given fractions
For the three liquids, we have:
- For liquid 1: V0=35V → ρ1
- For liquid 2: V0=29V → ρ2
- For liquid 3: V0=811V → ρ3
Step 5: Write the relationships
Using the inverse relationship, we can write:
ρ1∝135=53
ρ2∝129=92
ρ3∝1811=118
Step 6: Compare the densities
Now we need to compare these values:
- ρ1 is proportional to 53 (approximately 1.67)
- ρ2 is proportional to 92 (approximately 4.5)
- ρ3 is proportional to 118 (approximately 1.375)
Step 7: Determine the order of densities
From the proportional values, we can see:
- ρ3<ρ1<ρ2
Conclusion
Thus, the correct relationship between the densities is:
ρ3<ρ1<ρ2
Final Answer
The correct option is: ρ3<ρ1<ρ2.
---
When an object floats in a liquid, the weight of the liquid displaced by the submerged part of the object is equal to the weight of the object. This is known as Archimedes' principle.
Let:
- V0 = volume of the object submerged in the liquid
- V = total volume of the object
- ρ = density of the liquid
- g = acceleration due to gravity (constant)
Thrust=V0⋅ρ⋅g
Since the object is in equilibrium, this thrust equals the weight of the object:
V0⋅ρ⋅g=m⋅g
Where m is the mass of the object. Since g is constant, we can simplify this to:
V0⋅ρ=m
From the equation above, we can express the volume submerged V0 as:
V0=mρ
This shows that the volume submerged is inversely proportional to the density of the liquid.
For the three liquids, we have:
- For liquid 1: V0=35V → ρ1
- For liquid 2: V0=29V → ρ2
- For liquid 3: V0=811V → ρ3
Using the inverse relationship, we can write:
ρ1∝135=53
ρ2∝129=92
ρ3∝1811=118
Now we need to compare these values:
- ρ1 is proportional to 53 (approximately 1.67)
- ρ2 is proportional to 92 (approximately 4.5)
- ρ3 is proportional to 118 (approximately 1.375)
From the proportional values, we can see:
- ρ3<ρ1<ρ2
Thus, the correct relationship between the densities is:
ρ3<ρ1<ρ2
The correct option is: ρ3<ρ1<ρ2.
Topper's Solved these Questions
- THRUST AND PRESSUREBOOK - MTG IIT JEE FOUNDATIONCHAPTER - THRUST AND PRESSUREEXERCISE - Exercise (Integer /Numerical value type)
5 Videos
- SOUNDBOOK - MTG IIT JEE FOUNDATIONCHAPTER - SOUNDEXERCISE - Olympiad/HOTS Corner
15 Videos
- WORK AND ENERGYBOOK - MTG IIT JEE FOUNDATIONCHAPTER - WORK AND ENERGYEXERCISE - Olympaid/HOTS Corner
20 Videos
Similar Questions
Knowledge Check
An object is put turn in three liquids having different densities. The object floats with 19, 211 and 37 parts of its volume outside the liquid surfacei n liquids of densities d1,d2 and d3 respectively. Which of the following statements is correct?
Ad1>d2>d3Bd1>d2<d3Cd1<d2>d3Dd1<d2<d3An object is put one by one in three liquids having different densities. The object floats with 19,212and37 parts of their volumes outside the liquid surface in liquids of densities d1,d2andd3, respectively. Which of the following statement is correct ?
Ad1>d2>d3Bd1>d2<d3Cd1<d2>d3Dd1<d2<d3An object is put on by one in three liquids having different densities. The object floats with 1/9, 2/11 and 3/7 parts of their volumes outsides the liquid surface in liquids of densities d1, d2 and d3 respectively. Which of the following statements is correct?
Ad1>d2>d3Bd1>d2<d3Cd1<d2>d3Dd1<d2<d3
If two liquids of same masses but densities ρ1andρ2, respectively are mixed, then the density of mixture is given by
View SolutionIf two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
View SolutionThree different liquids are filled in a U-tube as shown in figure. Their densities are ρ1,ρ2 and ρ3 respectively. From the figure we may conclude that
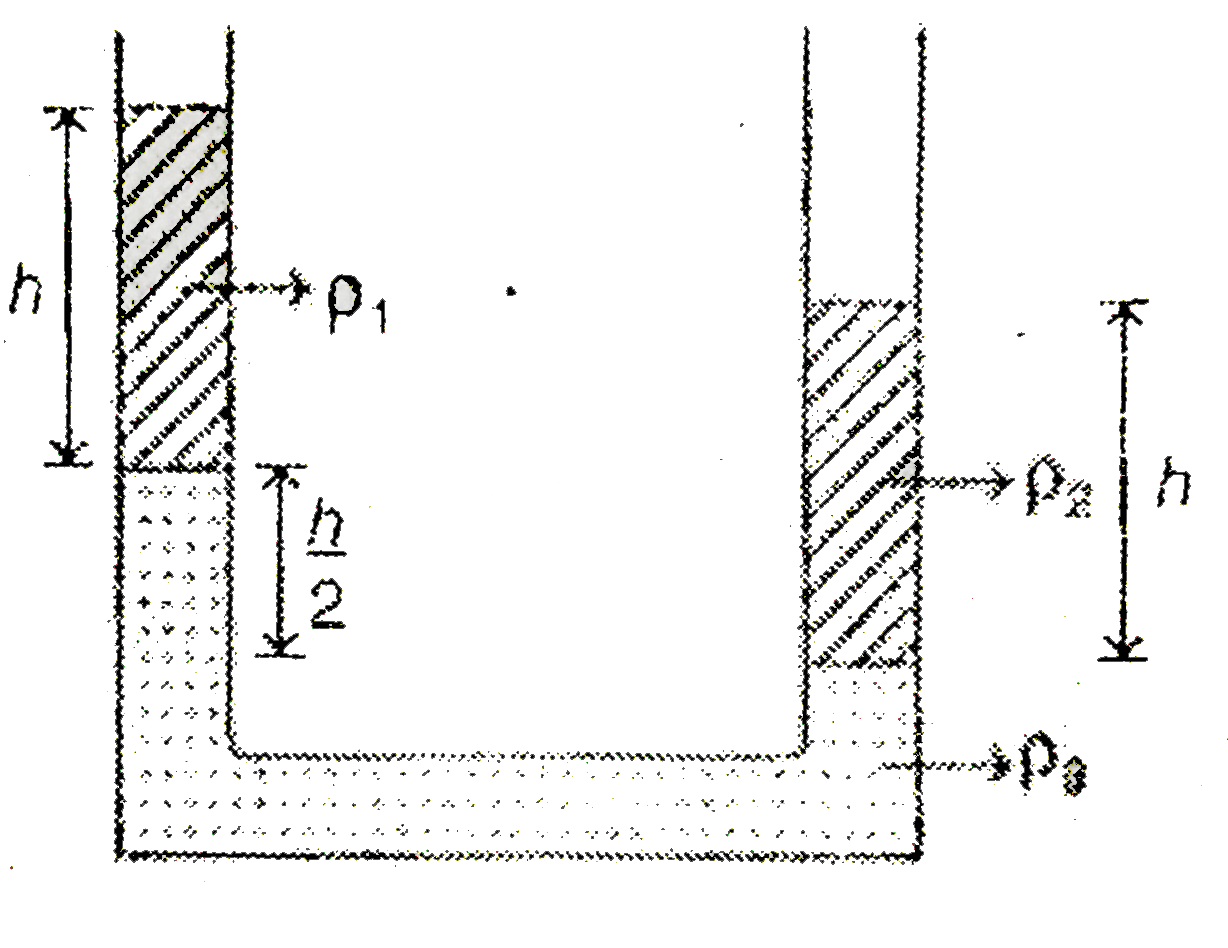
View SolutionWhen an object is made to float in two different liquids of density d1andd2, the lengths of the object seen above the liquid surface are l1andl2, respectively.
Which of the following is the correct alternative ?
View SolutionIf two liquids of same volume but different densities ρ1andρ2 are mixed, then density of mixture is given by
View Solution
