The cube of the number ρ is 16 times the number. Than find ρ where ρ≠0 and ρ≠−4.
The correct Answer is:Hance p=4.
To solve the problem, we need to find the value of the number ρ such that the cube of ρ is 16 times the number itself. We can follow these steps:
Step 1: Set up the equation
The problem states that the cube of the number ρ is equal to 16 times the number. We can express this mathematically as:
ρ3=16ρ
Step 2: Rearrange the equation
To solve for ρ, we can rearrange the equation:
ρ3−16ρ=0
Step 3: Factor the equation
Next, we can factor out ρ from the equation:
ρ(ρ2−16)=0
Step 4: Further factor the quadratic
The expression ρ2−16 is a difference of squares, which can be factored further:
ρ(ρ−4)(ρ+4)=0
Step 5: Solve for ρ
Now we can set each factor equal to zero:
1. ρ=0
2. ρ−4=0 → ρ=4
3. ρ+4=0 → ρ=−4
Step 6: Exclude the invalid solutions
The problem states that ρ≠0 and ρ≠−4. Therefore, we can exclude ρ=0 and ρ=−4.
Conclusion
The only valid solution left is:
ρ=4
The problem states that the cube of the number ρ is equal to 16 times the number. We can express this mathematically as:
ρ3=16ρ
To solve for ρ, we can rearrange the equation:
ρ3−16ρ=0
Next, we can factor out ρ from the equation:
ρ(ρ2−16)=0
The expression ρ2−16 is a difference of squares, which can be factored further:
ρ(ρ−4)(ρ+4)=0
Now we can set each factor equal to zero:
1. ρ=0
2. ρ−4=0 → ρ=4
3. ρ+4=0 → ρ=−4
The problem states that ρ≠0 and ρ≠−4. Therefore, we can exclude ρ=0 and ρ=−4.
The only valid solution left is:
ρ=4
Topper's Solved these Questions
- CUBES AND CUBE ROOTSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CUBES AND CUBE ROOTSEXERCISE - Olympiad/HOTS Corner
20 Videos
- CUBES AND CUBE ROOTSBOOK - MTG IIT JEE FOUNDATIONCHAPTER - CUBES AND CUBE ROOTSEXERCISE - Exercise (Subjective Problems.) (Long Answer Type)
5 Videos
- COMPARING QUANTITIES BOOK - MTG IIT JEE FOUNDATIONCHAPTER - COMPARING QUANTITIES EXERCISE - Olympiad/HOTS Cornet
15 Videos
- DATA HANDLINGBOOK - MTG IIT JEE FOUNDATIONCHAPTER - DATA HANDLINGEXERCISE - Olympiad/HOTS Corner
7 Videos
Similar Questions
The density of the core of planet is ρ1 and that of the outer shell is ρ2. The radius of the core that of the planet are R and 2R respectively. Gravitastional intensity at the surface of the planet is same as at a depth R. Find the ratio ρ1ρ2 , assuming them to be uniform independently.
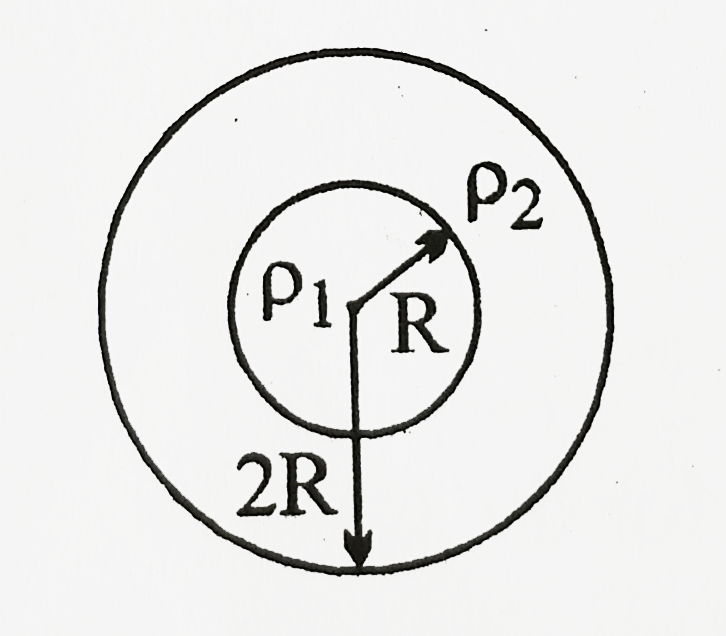
View SolutionThe density of the core a planet is ρ1 and that of the outer shell is ρ2. The radii of the core and that of the planet are R and 2R respectively. The acceleration due to gravity at the surface of the planet is same as at a depth R. Find the radio of ρ1ρ2

View Solution
Knowledge Check
If P is the pressure of a gas and ρ is its density , then find the dimension of velocity in terms of P and ρ .
AP1/2ρ−1/2BP1/2ρ1/2CP−1/2ρ−1/2DP−1/2ρ−1/2It P is the pressure and ρ is the density of a gas, then P and ρ are realted as :
AP∝ρBP∝ρ2CP∝1/ρDP∝1/ρ2
Find the length of the curve φ=12(ρ+1/ρ) between ρ=2andρ=4
View SolutionOn R, the set of real numbers, a relation ρ is defined as aρb if and only if 1+ab>0 Then
View SolutionFind the area of the regions bounded by the curve ρ=2acos3φ and the arcs of the circle ρ=a and situated outside the circle.
View SolutionA steel ball floats in a vessel with mercury. How will be volume of the part of the ball submerged in mercury change if a layer of water completely covering the ball is poured above the mercury? If ρw,ρs and ρm are the densities of water, steel and mercury, find the ratio of these two volumes in terms in of ρw,ρs and ρm.
View SolutionA spherical region of space has a distribution of charge such that the volume charge density varies with the radial distance from the centre r as ρ=ρ0r3,0≤r≤R where ρ0 is a positive constant. The total charge Q on sphere is
View SolutionA cube of 1 kg is floating at the interface of two liquids of density ρ1=3/4gm/cm3andρ2=2.5gm/cm3 The cube has (3/5)th part in ρ2 and (2/5)th part in ρ1 .Find the density of the cube .

View Solution
