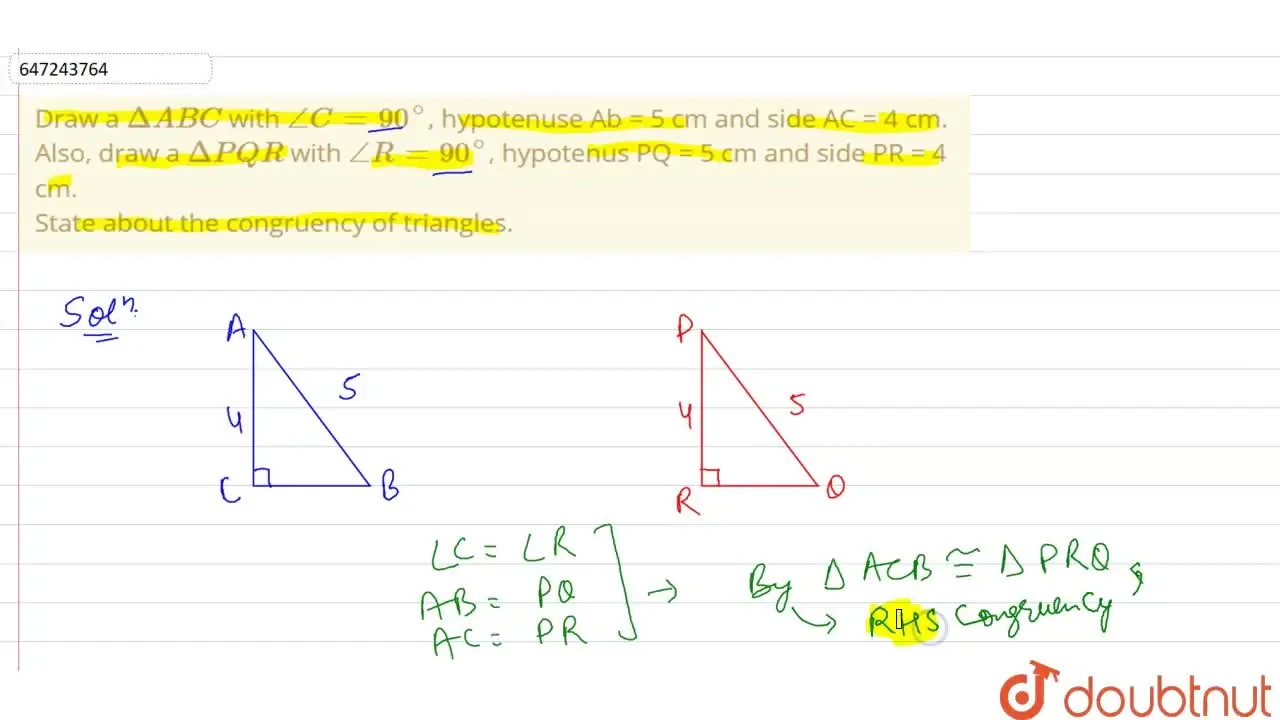
Draw a ΔABC with ∠C=90∘, hypotenuse Ab = 5 cm and side AC = 4 cm. Also, draw a ΔPQR with ∠R=90∘, hypotenus PQ = 5 cm and side PR = 4 cm.
State about the congruency of triangles.
The correct Answer is:ΔABC≅ΔPQR
Step by step video, text & image solution for Draw a DeltaABC with angleC=90^(@), hypotenuse Ab = 5 cm and side AC = 4 cm. Also, draw a DeltaPQR with angleR=90^(@), hypotenus PQ = 5 cm and side PR = 4 cm. State about the congruency of triangles. by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Related Playlists
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - Solved Examples24videos
CONGRUENCE OF TRIANGLES
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - CONGRUENCE OF TRIANGLES EXERCISE - NCERT Section (Exercise 7.1)9videos
CONGRUENCE OF TRIANGLES
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - COMPARING QUANTITIES EXERCISE - Olympaid/HOTS Corner20videos
COMPARING QUANTITIES
- BOOK - MTG IIT JEE FOUNDATIONCHAPTER - DATA HANDLINGEXERCISE - Olympiad / Hots Corner20videos
DATA HANDLING
Similar Questions
Draw a ΔABC with AB = 4 cm, BC = 6 cm and CA = 3 cm. Draw another ΔPQR with PQ = 4 cm, QR = 6 cm and RP = 3 cm. State about the congruency of triangles.
 View Solution
View SolutionDraw a right triangle with hypotenuse of length 5 cm and one side of length 4 cm.
View SolutionIn a triangle PQR,∠PQR=90∘,PQ=10cmandPR=26cm, then what is the value (in cm) of inradius of incircle?
View SolutionIn ΔPQR right angled at Q , PQ = 3 cm and PR = 6 cm .
Determine side QR.View SolutionIn ΔPQR,∠PQR=90∘,PQ=5cmandQR=12cm. What is the radius (in cm) of the circum-circle of ΔPQR?
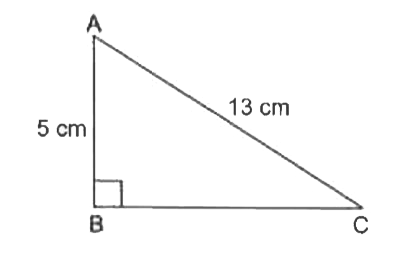
View SolutionIn △ABC, ∠B=90∘ , AB=5cm and AC=13cm. Then, BC = ?
 View Solution
View SolutionDraw a right triangle whose hypotenuse is of length 4 cm and one side is of length 2.5 cm.
View SolutionIn ΔABC,AB=4cm,AC=5cmandBC=6cm In ΔPQR,PR=4cm,PQ=5cmandRQ=6cm.ΔABC is congruent to
View SolutionIn ΔPQR,∠PQR=90∘,PQ=5cmandQR=12cm. What is the radius (in cm) of the circum-circle of ΔPQR?
View SolutionIn an isosceles triangle ABC,∠B=90∘ and side AB=6cm. Side AC will be
View Solution