Derive an expression for torque acting on a bar magnet placed in a uniform magnetic field.Solution in Punjabi
Consider a rectangular loop ABCD carrying current I in anticlockwise direction i.e., in direction ABCD.
Let l=AB=CD= Length of the rectangular loop.
b=BC=DA= Breadth of the rectangular loop.
→B= Strength of magnetic field directed from left to right in the plane of paper.
θ= Angle between the normal to plane of coil and direction of magnetic field.
Force acting on the arm ABof the loop.
→F1=I(→l×→B)
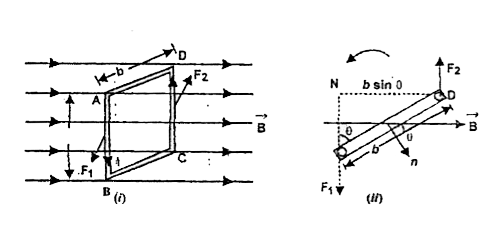
Direction of →F1 is perpendicular to the length of arm AB and directed outward of the sheet of paper (Fleming.s Left Hand Rule ).
Similary, force acting on the arm CD of the loop.
→F2=I(→l×→B) ...(ii)
According to Fleming.s Left Hand Rule, →F2is normal to the length of arm CD and is directed inside the sheet of the paper.
Forces acting on arm BC and on arm DA are equal and opposite to each other and act alongthe same line of action and hence cancel out in pairs.
→F1and→F2 are also equal and opposite to each other, but their line of action is not same.
So →F1and→F2 form a couple and try to rotate the loop anticlockwise.
The magnitude of torque is given by
τ= Either force ×Perpendicular distance between →F1and→F2.
τ=F1×DN
τ=I(→l×→B)×DN
=I(lBsin90∘)×bsinθ
=I(lb)Bsinθ
τ=IABsinθ
where A=lb= Area of the loop
if the loop has N turns then
τ=NIABsinθ
This torque will be maximum when θ=90∘
So τmaxi=NIAB
This torque will be minimum when θ=0∘
So τmini=NIABsin0
τmini=0
Topper's Solved these Questions
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CURRENTEXERCISE - SHORT ANSWER TYPE QUESTIONS (MOST EXPECTED QUESTIONS)5videos
FORCE ON A CURRENT
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CURRENTEXERCISE - LONG ANSWER TYPE QUESTIONS15videos
FORCE ON A CURRENT
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CURRENTEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS (MOST EXPECTED QUESTIONS )5videos
FORCE ON A CURRENT
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - FORCE ON A CHARGE EXERCISE - NUMERICAL PROBLEMS14videos
FORCE ON A CHARGE
- BOOK - BETTER CHOICE PUBLICATIONCHAPTER - GAUSS'S THEOREMEXERCISE - MOST EXPECTED NUMERICALS4videos
GAUSS'S THEOREM
Similar Questions
What is a safety fuse? Explain its function.
02:40View SolutionState the principle of moving coil galvanometer?
03:16View SolutionDefine an ampere in terms of the force between current carrying conductors.
03:06View SolutionAn electron (with charge 1.6×10−19C) moving at right angles to a uniform magnetic field completes a circular orbit in 10−6sec. Calculate the vlaue of magnetic field. Given mass of electron =9×10−31kg.
03:31View SolutionA box encloses an electric dipole of 3μCand−3C, if the dipole length is 6 cm, then find total electric flux associated with box.
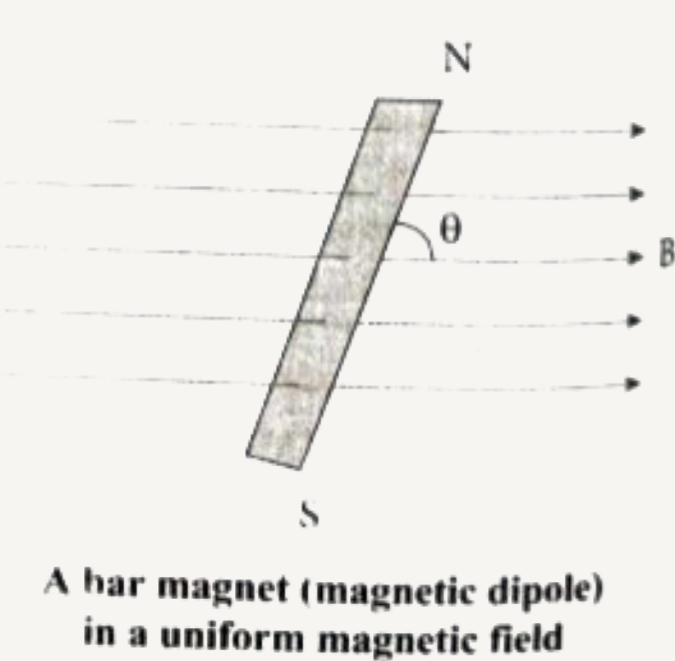
02:59View SolutionDerive an expression for torque acting on a bar magnet held at an angle with the direction of a uniform magnetic field.
02:59View SolutionThe magnetic moment of a a small bar magnet is 0.9a.m2 If it is placed in a uniform magnetic field making an angle of 30∘ It , a torque of 0.063N.m Acts on it. What is the magnetic field ?
06:43View SolutionFind an expression for the torque acting on a magnetic dipole placed in an uniform magnetic field . Hence define magnetic dipole moment .
03:51View SolutionDerive an expression for potenstial energy of bar magnet in a uniform magnetic field.
 04:34View Solution
04:34View SolutionWrite the equation of torque acting on bar magnet placed in uniform magnetic field.
08:43View SolutionWrite the equation of torque acting on bar magnet placed in uniform magnetic field.
03:12View SolutionDerive an expression for torque acting on a barmagnet placed in magnetic field
05:16View SolutionDerive an expression for torque acting on a bar magnet placed in a uniform magnetic field.
04:40View SolutionDerive an expression for Torque acting on a bar magnet placed in a uniform magnetic field.
46:11View SolutionDerive an expression for torque acting on a rectangular current loop carrying coil placed in a uniform magnetic field.
 View Solution
View SolutionDerive an expression for a torque acting on a bar magnet placed in uniform magnetic field B.
View Solution
