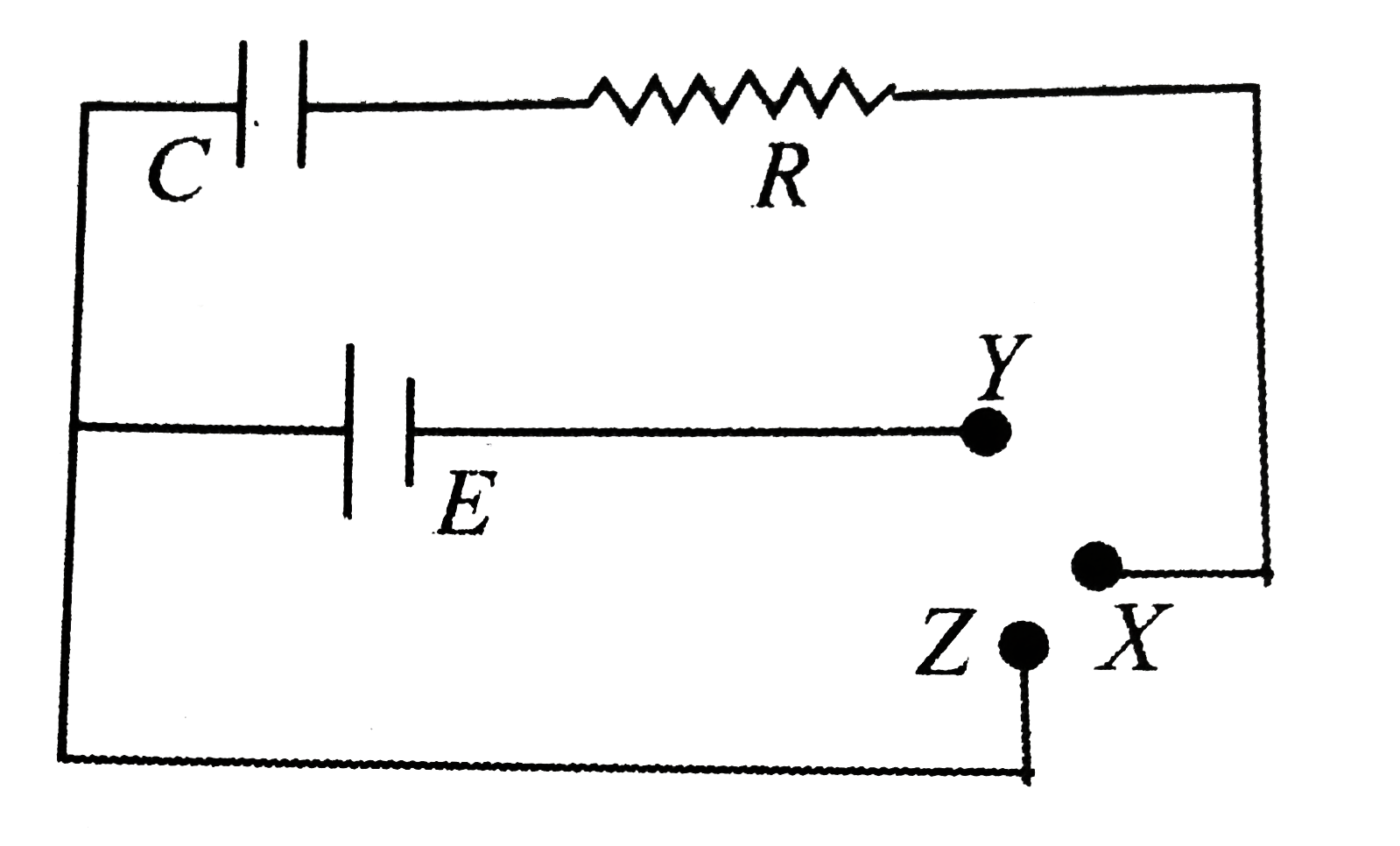
In the current shown Fig., X is joined to Y for a long time and then X is joined to Z. The total heat produced in R2 is

LE22R21
LE22R22
LE22R1R2
LE2R22R31
The correct Answer is:A
Steady state current in L=i0=ER1Energy stored in L=12L(ER1)2 = heat produced in R2 during discharge =LE22R21
Similar Questions
In the circuit shown, initially the switch is in position 1 for a long time. Then the switch is shifted to position 2 for a long time. Find the total heat produced in R2
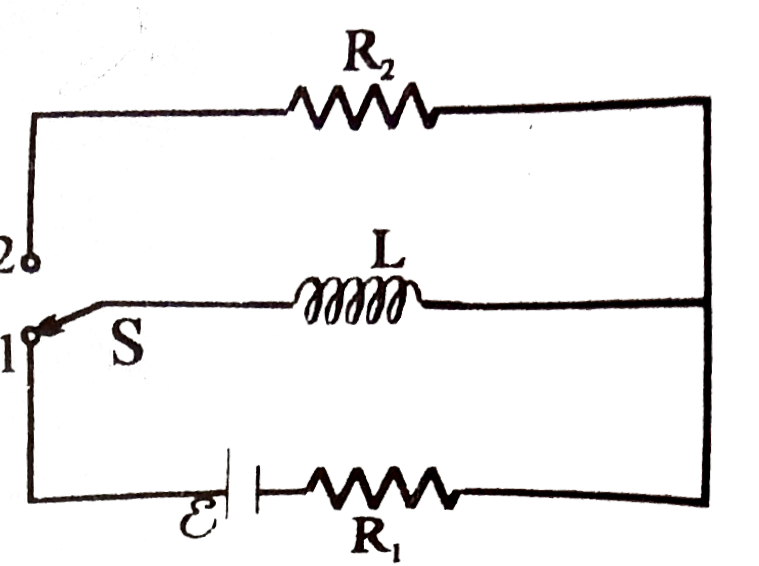
View Solution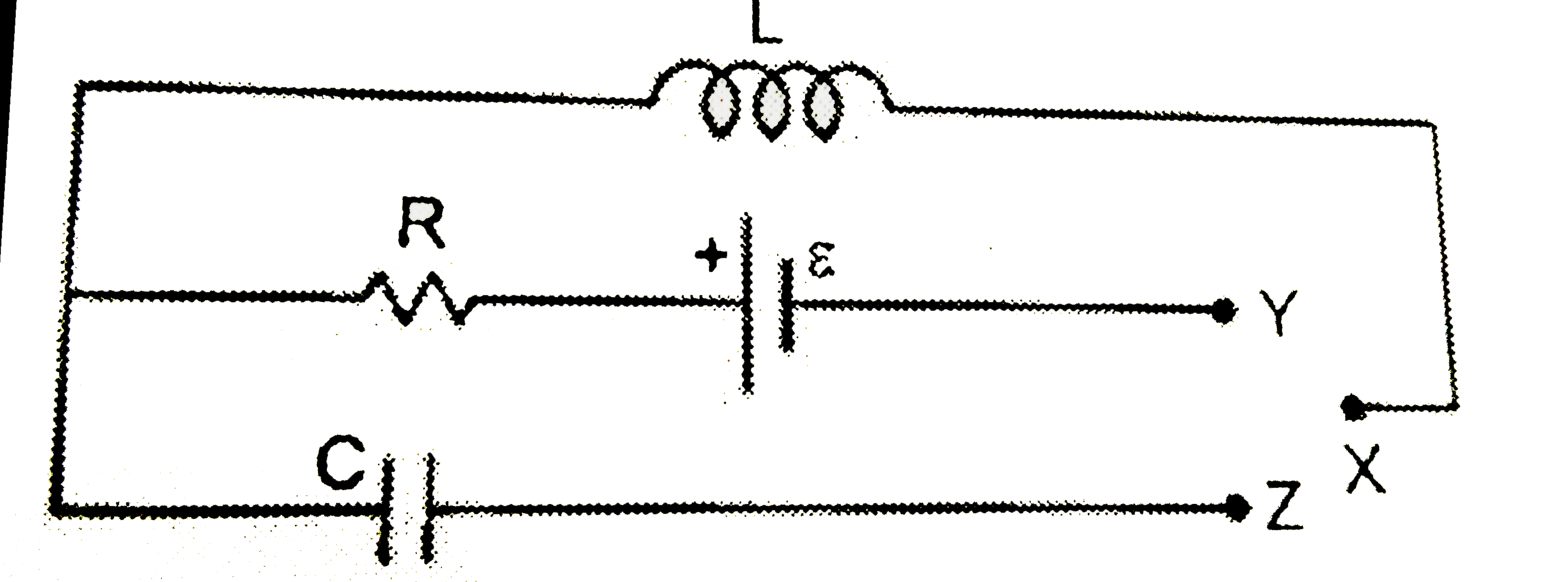
In the circuit shown, the symbols have their usual meanings. The cell has emf ɛ.X is initially joined to Y for a long time. Then X is joined to Z. The maximum charge on C at any later time will be
View Solution
Knowledge Check
In the current shows Fig x is joined to Y for a long time and then X is joined to Z. The total heat produced in R2 is
 ALE22R21BLE22R22CLE22R1R2DLE2R22R31
ALE22R21BLE22R22CLE22R1R2DLE2R22R31In the circuit shown switch S is connected to position 2 for a long time and then joined to position 1. The total heat produced in resistance R1 is:
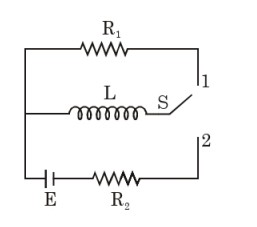 ALE22R22BLE22R21CLE22R1R2DLE2(R1+R2)22R21R22
ALE22R22BLE22R21CLE22R1R2DLE2(R1+R2)22R21R22
In the circuit shown, the symbols have their usual meanings. The cell has emf ɛ.X is initially joined to Y for a long time. Then X is joined to Z. The maximum charge on C at any later time will beAER√LCBER2√LCCE√LC2RDE√LCR
In the circuit shown the cells are ideal and of equal emfs, the capacitance of the capacitor is C and the resistance of the resistor is R. X is first joined to Yand then to Z. After a long time, the total heat produced in the resistor will be
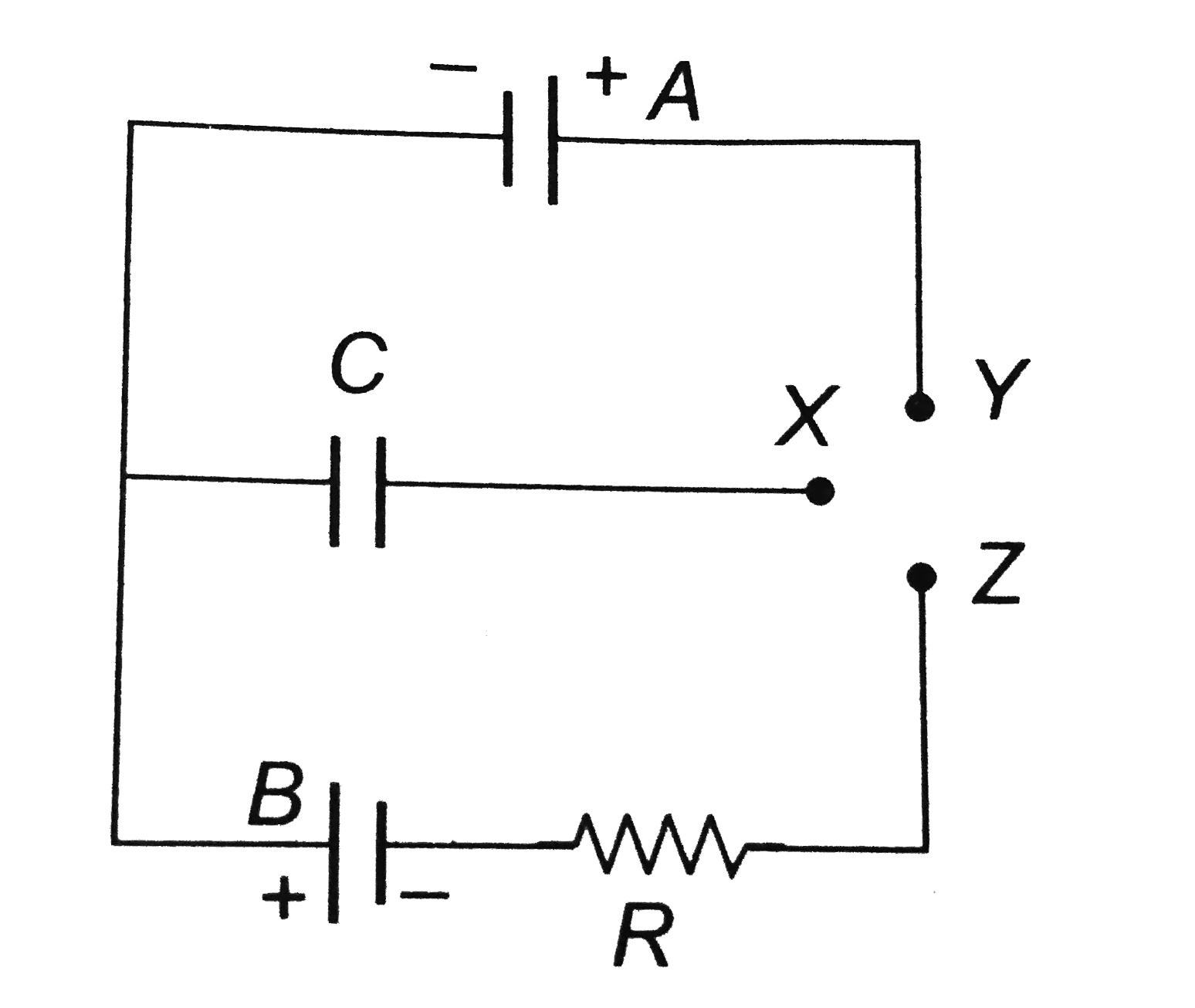
View SolutionThe capacitor C is initially without charge. X is now joined to Y for a long time, during which H1 heat is produced in the resistance R. X is now joined to Z for a long time, during which H2 heat is produced in R
View SolutionIn the circuit shows Fig the coil has inductance and resistance. When X is joined to Y, the time constant is t during the growth of current. When the steady state is reached, heat is produced in the coil at a rate P. X is now joined to Z. After joining X and Z:

View SolutionThe charge flowing through a resistance R varies with time t as Q=at−bt2. The total heat produced in R is
View SolutionRelation between current in a conductor and time is shown in fig.

If the resistance of the conductor is R, then the total heat disspipated across resistance R is
View Solution