आयताकार प्लेट पर दर्शायी अक्ष के सापेक्ष जड़त्व आघूर्ण दिया गया है :
I1=Mb212 तथा I3=M(a2+b2)12
I2 ज्ञात कीजिए।
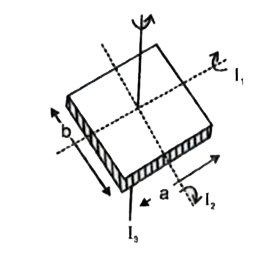
I1+I2=I3 ( लम्बवत् प्रमेय से )
अतः I2=I3−I1
I2=Ma212
Similar Questions
The moment of inertia of thin square plate ABCD of uniform thickness about an axis passing through the center O and perpendicular to the plane of the plate is ( i ) I_(1)+I_(2) ( ii ) I_(2)+I_(4) ( iii ) I_(1)+I_(3) ( iv ) I_(1)+I_(2)+I_(3)+I_(4) where I_(1) , I_(2) , I_(3) and I_(4) are repectively moments of inertia about axes 1 , 2 , 3 and 4 which are in the plane of the plane
View SolutionFind relation in between current i_(1), i_(2), i_(3), i_(4), i_(5)" and "i_(6) .
View SolutionIf ⇒In=∫a+π/2acos2nxsinxdx,thenI2−I1,I3−I2,I4−I3 are in
View Solution{:(" 'अ' ", " 'ब' "),(1."जूल" xx "सेकण्ड",(i)" आयताकार पटल का जड़त्व आघूर्ण"),(2." "[ML^(2)T^(-1)],(ii)" "(2)/(5)MR^(2)),(3." जड़त्व आघूर्ण",(iii) " कोणीय संवेग"),(4. " "I=M((l^(2)+b^(2))/(12)),(iv)" घूर्णन गति"),(5. " एक ठोस गोला ( द्रव्यमान M, त्रिज्या",(v)" कोणीय संवेग का विमीय सूत्र"),(" R ) व्यास के परितः जड़त्व आघूर्ण",):}
View SolutionIf I1,I2,I3 are excentres of the triangle with vertices (0, 0), (5, 12), (16, 12) then the orthocentre of ΔI1I2I3 is
View Solutionउन रेखाओं के मध्य कोण ज्ञात कीजिए जिसमें एक दिक् - अनुपात 2, 1, 2 है और रेखा बिंदुओं (1, 4, 3) तथा (2, 12, 7) से जाती है ।
View SolutionI,I1,I2,I3 are the in centre and excentres of ΔABC .If I(0,0),I1(2,3),I2(5,7) then the, distance between the orthocentres of ΔII1I3 and ΔI1I2I3is
View SolutionIf In=∫cotnxdx then I0+I1+2(I2+I3)+I4+I5=
View Solution
