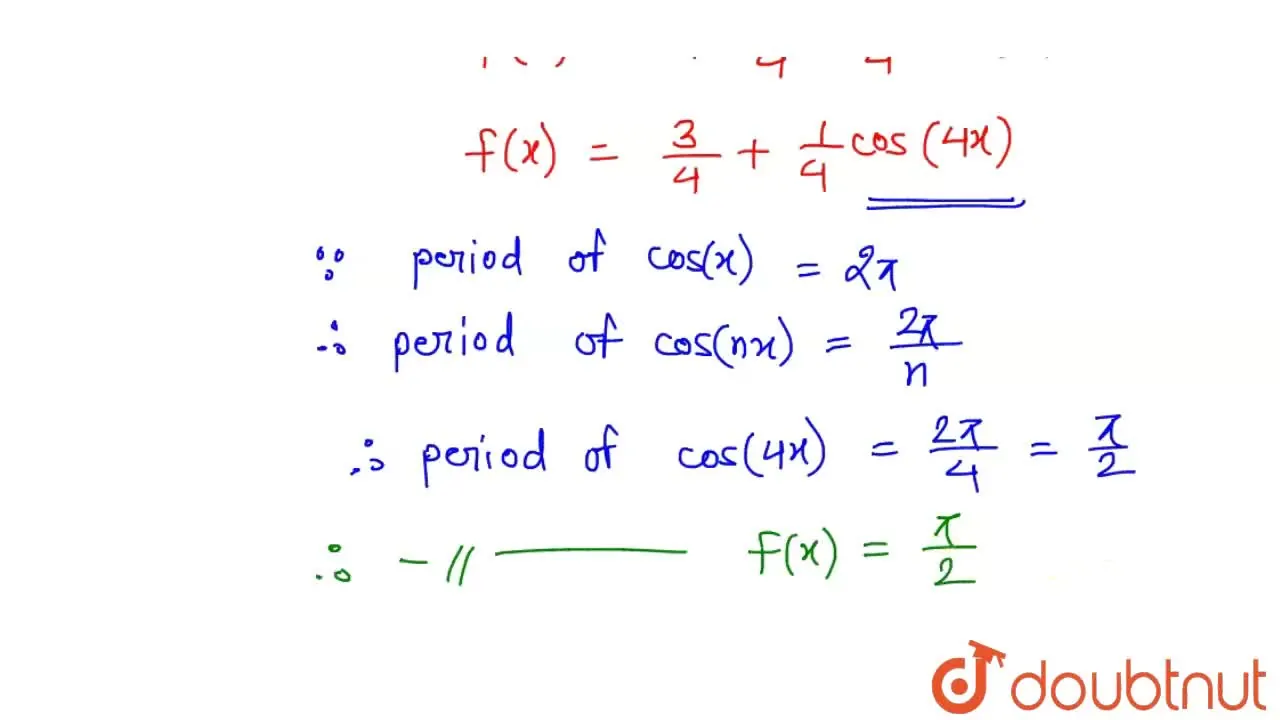
Fundamental period of the function f(x)=sin4x+cos4x=
π
2π
π/2
None
The correct Answer is:C
Step by step video, text & image solution for Fundamental period of the function f (x) = sin^4 x+ cos^4 x= by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - FUNCTIONSEXERCISE - PROBLEM SET (2)40videos
FUNCTIONS
- BOOK - ML KHANNACHAPTER - FUNCTIONSEXERCISE - PROBLEM SET (3)71videos
FUNCTIONS
- BOOK - ML KHANNACHAPTER - EXPONENTIAL AND LOGARITHMIC SERIES EXERCISE - Problem Set (2) (Self Assessment Test)8videos
EXPONENTIAL AND LOGARITHMIC SERIES
- BOOK - ML KHANNACHAPTER - HEIGHTS AND DISTANCESEXERCISE - Problem Set (3) FILL IN THE BLANKS9videos
HEIGHTS AND DISTANCES
Similar Questions
Fundamental period of the function f(x) = cos (sinx) + cos(cosx) is :
View SolutionThe period of the function
f (x) =|sin xl + |cos x| isView SolutionIf fundamental period of the functions f(x)=sin2x+cos4x and g(x)=cos(sin2x)+cos(cos2x) are λ1 and λ2 respectively then λ1λ2=
View SolutionFind the fundamental period of the following function:
f(x)=sin3x+cos2x+|tanx|View SolutionThe period of the function f (x)=sin 4x+tan 2x is
View SolutionThe period of the function f(x)=|sinx|+|cosx| is
View SolutionThe fundamental period of the function f(x)=|sin2x|+|cos2x| is
View SolutionThe period of the function f(x)=sin43x+cos43x, is
View SolutionThe function f(x)=sin4x+cos4x increases if
View SolutionThe period of the function f(x)=|sin2x|+|cos8x| is
View Solution