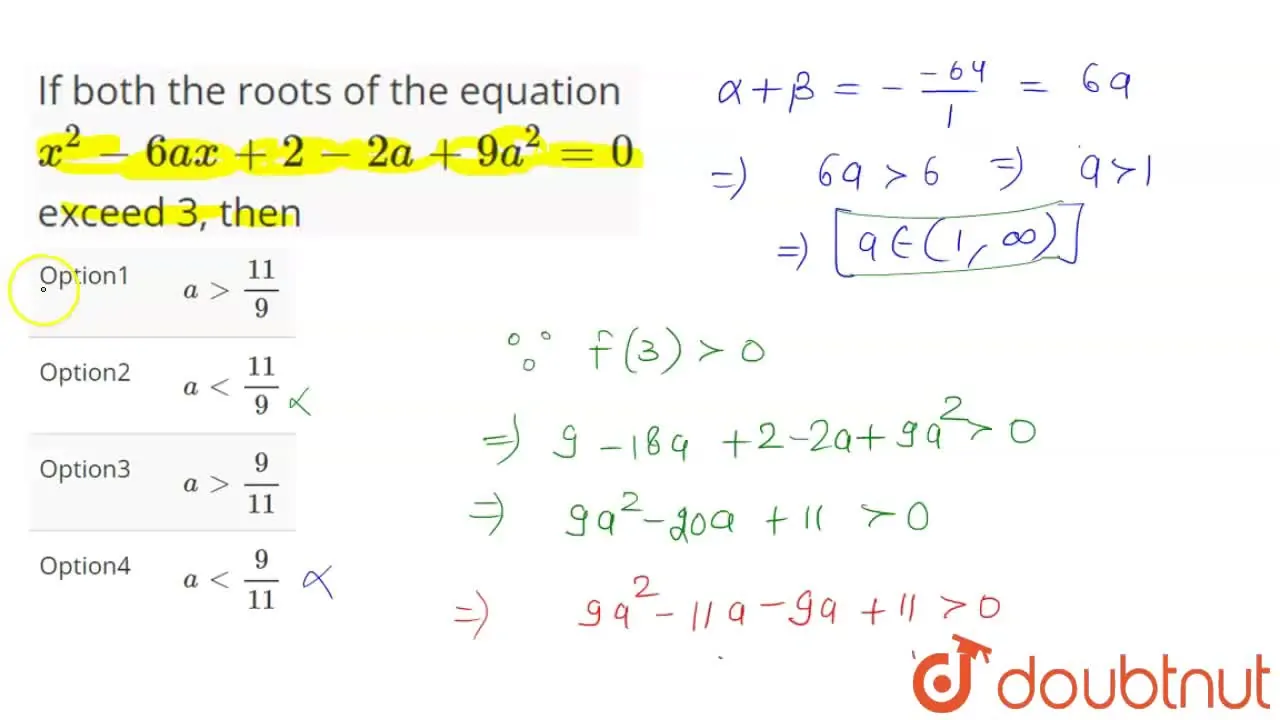
If both the roots of the equation x2−6ax+2−2a+9a2=0 exceed 3, then
a>119
a<119
a>911
a<911
The correct Answer is:A
Step by step video, text & image solution for If both the roots of the equation x^(2)-6ax+2-2a+9a^(2)=0 exceed 3, then by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - THEORY OF QUADRATIC EQUATIONS EXERCISE - Problem Set - 2 (True And False)4videos
THEORY OF QUADRATIC EQUATIONS
- BOOK - ML KHANNACHAPTER - THEORY OF QUADRATIC EQUATIONS EXERCISE - Problem Set - 2 (Fill In The Blanks)3videos
THEORY OF QUADRATIC EQUATIONS
- BOOK - ML KHANNACHAPTER - THEORY OF QUADRATIC EQUATIONS EXERCISE - Problem Set - 1 (Fill In The Blanks)4videos
THEORY OF QUADRATIC EQUATIONS
- BOOK - ML KHANNACHAPTER - THE PARABOLA EXERCISE - MISCELLANEOUS EXERCISE (Assertion/ Reason)1videos
THE PARABOLA
- BOOK - ML KHANNACHAPTER - TRIGONOMETRICAL EQUATIONS EXERCISE - SELF ASSESSMENT TEST27videos
TRIGONOMETRICAL EQUATIONS
Similar Questions
The roots of the equation 2x2−6x+3=0 are
View Solutionif both root of equation x2−6ax+2−2a+9a2=0 exceeds 3 . then show that a>119
View SolutionFind all values of a for which both roots of the equation x2−6ax+2−2a+9a2=0 are greater then 3.
View SolutionFind all the parameters p for which both the roots of the equation x2−6px+(3−4p+9p2)=0 exceed the number 4.
View SolutionLet S denotes the set of real values of 'a' for which the roots of the equation x2−ax−a2=0 exceeds 'a', then S equals to
View SolutionIf both roots of the equation x2−2ax+a2−1=0 lie between (−2,2) then a lies in the interval
View SolutionThe roots of the equation x2+6ix−9=0 are :
View SolutionFor what values of mεR, both roots of the equation x2−6mx+9m2−2m+2=0 exceed 3?
View SolutionIf the absolute value of the difference of the roots of the equation x2+ax+1=0exceeds√3a, then
View SolutionIf both roots of the equation x2−2ax+a2−1=0 lie between -3 and 4 ,then [a] is/are , where [ ] represents the greatest ineger function
View Solution