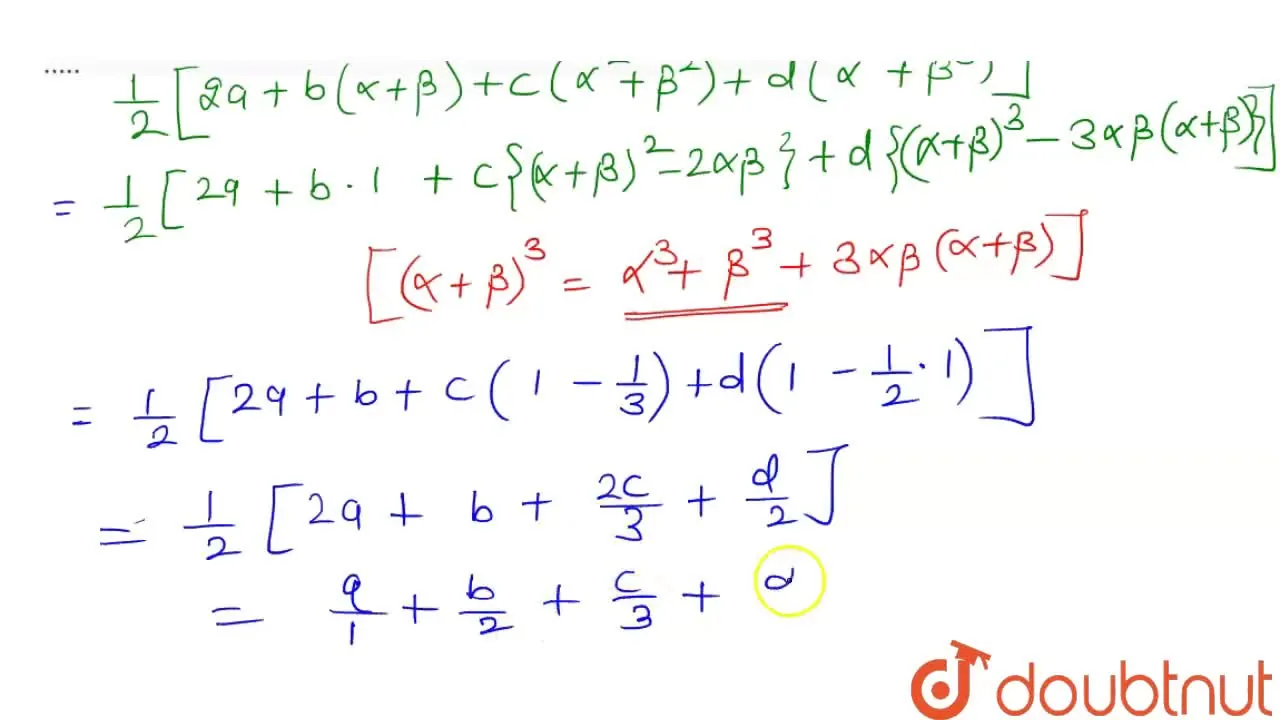
If α,β are the roots of the quadratic equation 6x2−6x+1=0, then
12(a+bα+cα2+dα3)+12(a+bβ+cβ2+dβ3)= …..
The correct Answer is:a1+b2+c3+d4
Step by step video, text & image solution for If alpha, beta are the roots of the quadratic equation 6x^(2)-6x+1=0, then (1)/(2) (a+balpha+c alpha^(2)+d alpha^(3))+(1)/(2) (a+b beta+c beta^(2)+ d beta^(3))= ….. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - THEORY OF QUADRATIC EQUATIONS EXERCISE - Problem Set - 266videos
THEORY OF QUADRATIC EQUATIONS
- BOOK - ML KHANNACHAPTER - THEORY OF QUADRATIC EQUATIONS EXERCISE - Problem Set - 2 (True And False)4videos
THEORY OF QUADRATIC EQUATIONS
- BOOK - ML KHANNACHAPTER - THEORY OF QUADRATIC EQUATIONS EXERCISE - Problem Set - 1 (True And False)3videos
THEORY OF QUADRATIC EQUATIONS
- BOOK - ML KHANNACHAPTER - THE PARABOLA EXERCISE - MISCELLANEOUS EXERCISE (Assertion/ Reason)1videos
THE PARABOLA
- BOOK - ML KHANNACHAPTER - TRIGONOMETRICAL EQUATIONS EXERCISE - SELF ASSESSMENT TEST27videos
TRIGONOMETRICAL EQUATIONS
Similar Questions
If α,β are the roots of the equation 5x2−5x+1=0, then find the value of 12(a+bα+cα2+dα3)+12(a+bβ+cβ2+dβ3).
View SolutionIf α,β are the roots of the quadratic equation 4x2−4x+1=0 then α3+β3=
View SolutionIf α and β are the roots of the quadratic equation x2−3x−2=0, then αβ+βα=
View SolutionIf αandβ are the roots of the quadratic equation 2x2+6x−5=0, then find (α+β) and α×β.
View SolutionIf αandβ are the roots of the quadratic equation 2x2+6x−5=0, then find (α+β) and α×β.
View SolutionIf α and β are the roots of the quadratic equation x2−5x+6=0 then find alpha^2 + beta^2
View SolutionIf α,β are the roots of the equation x2−p(x+1)−c=0, then (α+1)(β+1)=
View SolutionIf α and β are the roots of the quadratic equation x2−5x+6=0 then find αβ+βα
View SolutionIf αandβ are the roots of the quadratic equation x2−6x+1=0, then the value of α2β2+β2α2 is
View SolutionIf α and β are the roots of the equation 3x2+8x+2=0 then (1α+1β)=?
View Solution