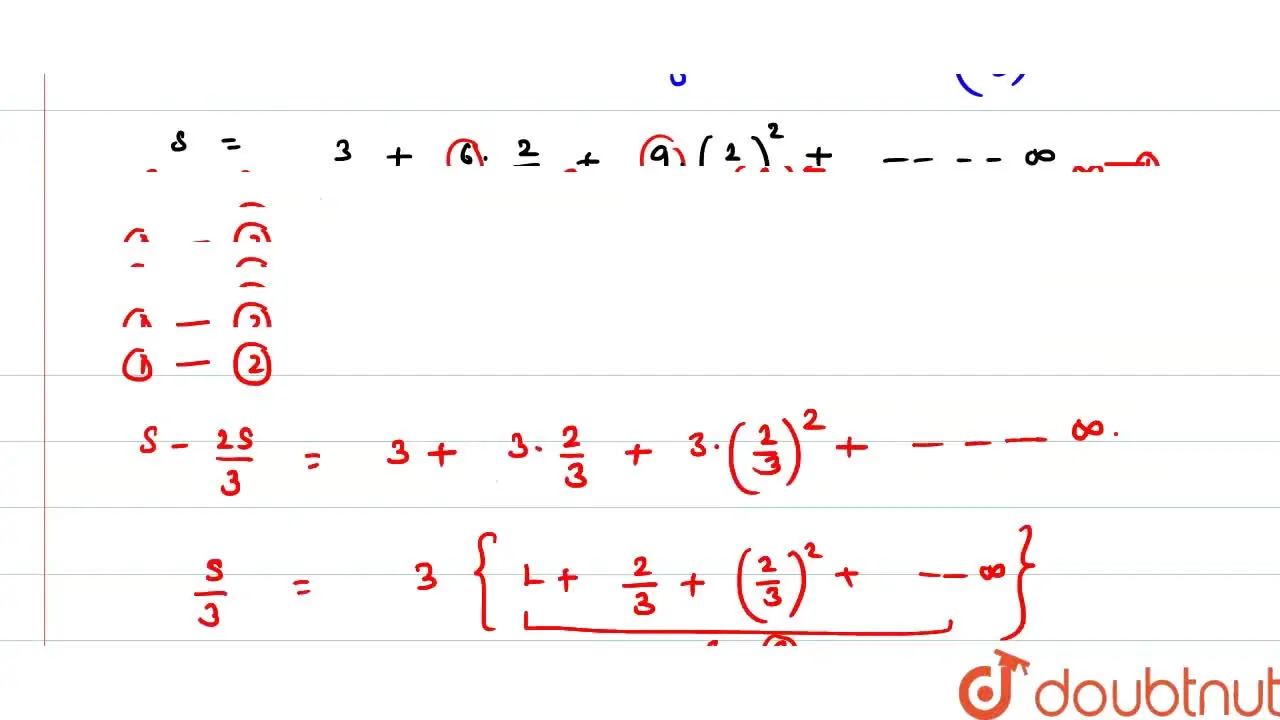
The sum to infinite terms of the arithmetic

Given that $a_{4} + a_{8} + a_{12} + a_{16} = 224$ , the sum of the first nineteen terms of the arithmetic progression
$a_{1}, a_{2}, a_{3}, \dots .$ is equal to
A1540
B1064
C3125
D1980
Find the 15th term of the arithmetic progression 10, 4, -2, ….
A $- 721 =$
B $- 74$
C $- 76$
D $- 78$
The sum of the first three terms of the arithmetic progression is 30 and the sum of the squares of the first term and the second term of the same progression is 116. Find the seventh term of the progression if its fith term is known to be exactly divisible by 14.
A36
B40
C43
D22

The sum to infinite terms of the arithmetic - gemoetric progression $3, 4, 4, \frac{32}{9}, \dots \dots$ is equal to