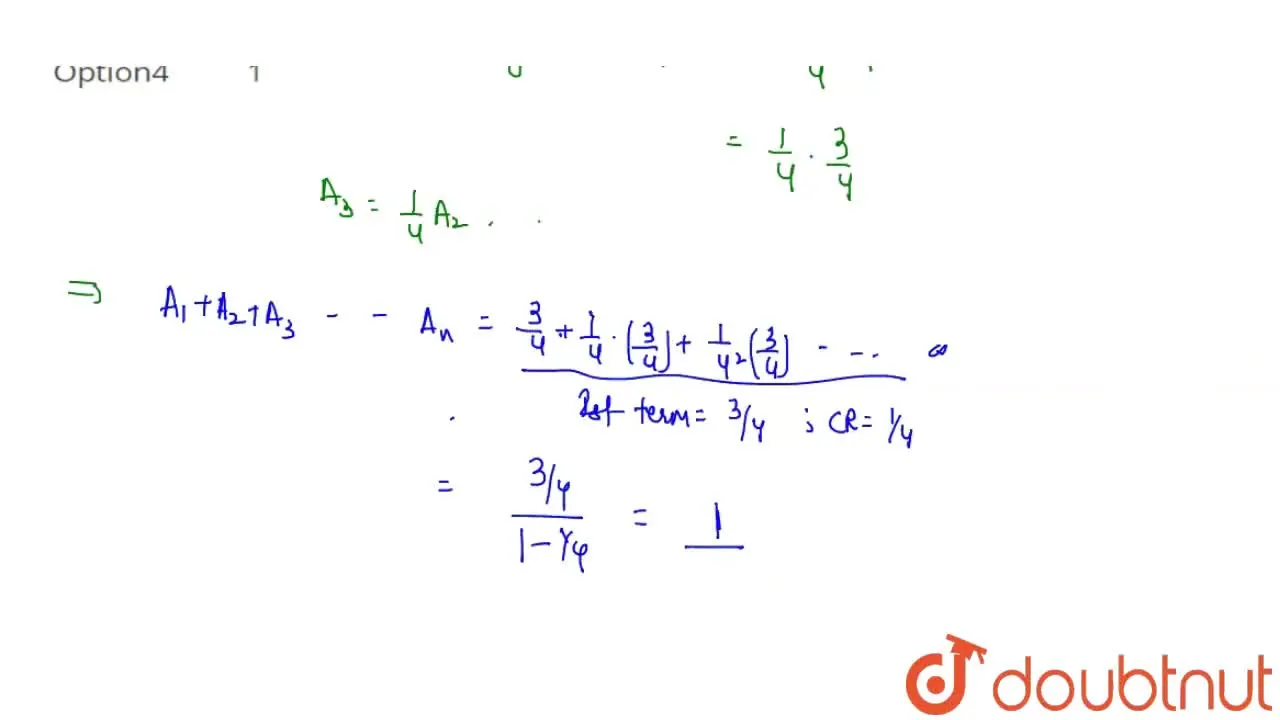
An equilateral triangle is drawn by joining the mid-points of a given equilateral triangle, A third equilateral triangle is drawn inside the second in the same manner and the process is continued indefinitely. If the side of the first equilateral triangle is 31/4 inch, then the sum of the areas in sq. inch of all these triangles is
√3
3
3√3
1
The correct Answer is:D
Step by step video, text & image solution for An equilateral triangle is drawn by joining the mid-points of a given equilateral triangle, A third equilateral triangle is drawn inside the second in the same manner and the process is continued indefinitely. If the side of the first equilateral triangle is 3^(1//4) inch, then the sum of the areas in sq. inch of all these triangles is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ML KHANNACHAPTER - PROGRESSIONS EXERCISE - PROBLEM SET - 2 (TRUE AND FALSE)2videos
PROGRESSIONS
- BOOK - ML KHANNACHAPTER - PROGRESSIONS EXERCISE - PROBLEM SET - 2 (FILL IN THE BLANKS)3videos
PROGRESSIONS
- BOOK - ML KHANNACHAPTER - PROGRESSIONS EXERCISE - PROBLEM SET - 1 (FILL IN THE BLANKS)4videos
PROGRESSIONS
- BOOK - ML KHANNACHAPTER - PROBABILITYEXERCISE - MISCELLANEOUS EXERCISE6videos
PROBABILITY
- BOOK - ML KHANNACHAPTER - PROPERTIES OF TRIANGLES EXERCISE - Self Assessment Test (Multiple Choise Questions)34videos
PROPERTIES OF TRIANGLES
Similar Questions
Area of an equilateral triangle
View Solutionthe area of an equilateral triangle is .
View SolutionAn equilateral triangle is drawn by joining the midpoints of the sides of another equilateral triangle. A third equilateral triangle is drawn inside the second one joining the midpoints of the sides of the second equilateral tringle, and the process continues infinitely. Find the sum of the perimeters of all the equilateral triangles, if the side of the largest equilateral triangle is 24 units.
View SolutionIf the side of an equilateral triangle is 16 cm, then the area of the triangle is
View SolutionA new triangle is formed by joining the mid-points of an equilateral triangle. Again a new triangle is formed by using the mid-points of this triangle, this process goes on till infinity. If the side of the big equilateral triangle is 24 units, find the total perimeter of such triangle formed ?
View SolutionWrite the type of triangle formed by joining the midpoints of the sides of an equilateral triangle.
View SolutionIf the side of an equilateral triangle is r, then the area of the triangle varies directly as .
View SolutionIf the side of an equilateral triangle is r, then the area of the triangle varies directly as .
View SolutionThe height of an equilateral triangle is 6cm, then the area of the triangle is
View SolutionWhat is the area of an equilateral triangle with side 2cm?
View Solution