In . What is the value (in degrees) of ?
50
70
90
100
The correct Answer is:C
To solve the problem, we need to find the value of ∠B+∠C given the ratio of the angles in triangle ABC as ∠A:∠B:∠C=5:4:1.
1. Assign Variables Based on Ratios:
Let the angles be represented in terms of a variable x:
- ∠A=5x
- ∠B=4x
- ∠C=1x
2. Use the Triangle Angle Sum Property:
The sum of the angles in a triangle is always 180∘.
Therefore, we can set up the equation:
∠A+∠B+∠C=180∘
Substituting the expressions for the angles:
5x+4x+1x=180∘
3. Combine Like Terms:
Combine the terms on the left side:
10x=180∘
4. Solve for x:
Divide both sides by 10:
x=180∘10=18∘
5. Find the Angles:
Now, we can find the individual angles:
- ∠A=5x=5×18∘=90∘
- ∠B=4x=4×18∘=72∘
- ∠C=1x=1×18∘=18∘
6. Calculate ∠B+∠C:
Now we can find ∠B+∠C:
∠B+∠C=72∘+18∘=90∘
Final Answer:
The value of ∠B+∠C is 90∘.
---
Let the angles be represented in terms of a variable x:
- ∠A=5x
- ∠B=4x
- ∠C=1x
The sum of the angles in a triangle is always 180∘.
Therefore, we can set up the equation:
∠A+∠B+∠C=180∘
Substituting the expressions for the angles:
5x+4x+1x=180∘
Combine the terms on the left side:
10x=180∘
Divide both sides by 10:
x=180∘10=18∘
Now, we can find the individual angles:
- ∠A=5x=5×18∘=90∘
- ∠B=4x=4×18∘=72∘
- ∠C=1x=1×18∘=18∘
Now we can find ∠B+∠C:
∠B+∠C=72∘+18∘=90∘
The value of ∠B+∠C is 90∘.
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)
35 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
30 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-II)
25 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
Knowledge Check
In ΔABC,∠C=54∘, the perpendicular bisector of AB at D meets BC at E. If ∠EAC=42∘, then what is the value (in degrees) of ∠ABC?
A25B42C50D60In ΔABC ,if A:B:C =3:5:4,then a+b+√2c=
A2bB2cC3bD3aIn a ΔABC, if 2∠A=3∠B=6∠C, value of ∠B is
A60∘B30∘C45∘D90∘
In a ΔABC,∠A+∠B=70∘ and ∠B+∠C=130∘, value of ∠A is
View SolutionIn a triangle ABC, if ∠A>∠B>∠C and the measures of ∠A,∠B and ∠C in degrees are integers, then the least possible value of ∠A is
View SolutionIn a ΔABC, if a = 4, b = 5 , c = 6 then angle C is equal to
View SolutionIn a Δ ABC, if 2∠A=3∠B=6∠C then calculate ∠A,∠B and ∠C.
View SolutionFor the figure given below, find the value (in degree) of 2∠A+∠B.
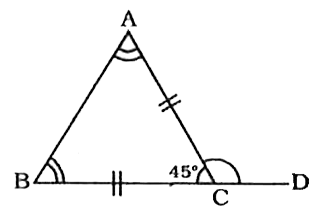
View Solution
