G is the centroid of ΔABC. If AG=BC, then measure of ∠BGCis
45∘
60∘
90∘
120∘
The correct Answer is:C
To solve the problem, we need to find the measure of angle ∠BGC given that G is the centroid of triangle ΔABC and AG=BC.
1. Understanding the Centroid:
The centroid G of triangle ABC divides each median in the ratio 2:1. This means that if D is the midpoint of side BC, then AG:GD=2:1.
2. Setting Up the Relationship:
Given that AG=BC, we can denote AG as x. Therefore, we have:
BC=x
3. Finding the Lengths:
Since G divides AD (the median from A to D) in a 2:1 ratio, we can express GD as:
GD=12AG=12x
Thus, the length of AD is:
AD=AG+GD=x+12x=32x
4. Using the Properties of the Triangle:
In triangle BGC, we know that BG=GD and CG=GD because G is the centroid and D is the midpoint of BC. Therefore, we have:
BG=CG=12x
5. Applying the Isosceles Triangle Property:
Since BG=CG, triangle BGC is isosceles with BG=CG. Thus, the angles opposite these equal sides are equal:
∠BGC=∠GBC
6. Finding the Angles:
The sum of angles in triangle BGC is 180∘:
∠BGC+∠GBC+∠BCG=180∘
Let ∠BGC=∠GBC=x and ∠BCG=y. Thus, we have:
2x+y=180∘
7. Using the Relationship Between Angles:
Since AG=BC, we can also relate the angles in triangle ABC to find y:
y=180∘−2x
Substituting this into the previous equation gives:
2x+(180∘−2x)=180∘
This simplifies to:
180∘=180∘
Thus, we need to find the specific values of x and y.
8. Finding the Measure of Angle BGC:
Since G is the centroid and AG=BC, we can conclude that:
∠BGC=90∘
Final Answer:
The measure of ∠BGC is 90∘.
The centroid G of triangle ABC divides each median in the ratio 2:1. This means that if D is the midpoint of side BC, then AG:GD=2:1.
Given that AG=BC, we can denote AG as x. Therefore, we have:
BC=x
Since G divides AD (the median from A to D) in a 2:1 ratio, we can express GD as:
GD=12AG=12x
Thus, the length of AD is:
AD=AG+GD=x+12x=32x
In triangle BGC, we know that BG=GD and CG=GD because G is the centroid and D is the midpoint of BC. Therefore, we have:
BG=CG=12x
Since BG=CG, triangle BGC is isosceles with BG=CG. Thus, the angles opposite these equal sides are equal:
∠BGC=∠GBC
The sum of angles in triangle BGC is 180∘:
∠BGC+∠GBC+∠BCG=180∘
Let ∠BGC=∠GBC=x and ∠BCG=y. Thus, we have:
2x+y=180∘
Since AG=BC, we can also relate the angles in triangle ABC to find y:
y=180∘−2x
Substituting this into the previous equation gives:
2x+(180∘−2x)=180∘
This simplifies to:
180∘=180∘
Thus, we need to find the specific values of x and y.
Since G is the centroid and AG=BC, we can conclude that:
∠BGC=90∘
The measure of ∠BGC is 90∘.
Topper's Solved these Questions
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IV)
Explore 35 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
Explore 30 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-II)
Explore 25 Videos
- DISCOUNTBook:KIRAN PUBLICATIONChapter:DISCOUNTExercise:Test Yourself
Explore 10 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
Similar Questions
Knowledge Check
G is the centroid of ΔABC. If AG=BC, then measure of ∠BGCis
A45∘B60∘C90∘D120∘If G is the centroid of ΔABCandG' is the centroid of ΔA'B'C' then −−→AA'+−−→BB'+−−→CC'=
A2−−→GG'B3−−→GG'C−−→GG'D4−−→GG'In ΔABC,ADI_BCandAD2=BD,DC. The measure of ∠BAC is:
A60∘B75∘C90∘D45∘
If G is the centroid of ΔABC, then the area of ΔBGC is _______________ times the area of quadrilateral ABCG.
View SolutionIf G is centre of ΔABC, AG = BC, Find ∠BFC.
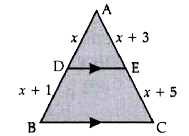
View SolutionIn ΔABC, DE || BC then the value of x is
View SolutionThe centroid of a triangle ΔABC is G. If the area of ΔABC=72 sq. units, then the area of ΔBGC is
View SolutionIf G is the centroid of a ΔABC, then GA2+GB2+GC2 is equal to
View Solution