The ratio of the number of boys and girls of a school with 504 students is 13:11. What will be the new ratio if 12 more girls are admitted?
91:81
81:91
9:10
10:9
The correct Answer is:A
To solve the problem step by step, we will follow these instructions:
Step 1: Understand the given ratio
The ratio of boys to girls is given as 13:11. This means that for every 13 boys, there are 11 girls.
Step 2: Calculate the total parts in the ratio
To find the total parts represented in the ratio, we add the parts for boys and girls:
Total parts=13+11=24
Step 3: Determine the value of one part
We know the total number of students in the school is 504. To find the value of one part, we divide the total number of students by the total parts:
Value of one part=50424=21
Step 4: Calculate the number of boys and girls
Now, we can find the actual number of boys and girls by multiplying the number of parts by the value of one part:
- Number of boys (B):
B=13×21=273
- Number of girls (G):
G=11×21=231
Step 5: Add the new girls
According to the question, 12 more girls are admitted. We will add this to the current number of girls:
New number of girls=231+12=243
Step 6: Find the new ratio of boys to girls
Now, we can find the new ratio of boys to girls:
New ratio=BNew number of girls=273243
Step 7: Simplify the ratio
To simplify the ratio, we can divide both numbers by their greatest common divisor (GCD). The GCD of 273 and 243 is 9:
273÷9243÷9=30.3327
However, we need to express this in whole numbers. We can multiply both parts by 3 to eliminate the decimal:
New ratio=3027 or 30:27
Step 8: Final simplification
This can be further simplified by dividing both parts by 3:
Final new ratio=109 or 10:9
Final Answer
The new ratio of boys to girls after admitting 12 more girls is 10:9.
---
The ratio of boys to girls is given as 13:11. This means that for every 13 boys, there are 11 girls.
To find the total parts represented in the ratio, we add the parts for boys and girls:
Total parts=13+11=24
We know the total number of students in the school is 504. To find the value of one part, we divide the total number of students by the total parts:
Value of one part=50424=21
Now, we can find the actual number of boys and girls by multiplying the number of parts by the value of one part:
- Number of boys (B):
B=13×21=273
- Number of girls (G):
G=11×21=231
According to the question, 12 more girls are admitted. We will add this to the current number of girls:
New number of girls=231+12=243
Now, we can find the new ratio of boys to girls:
New ratio=BNew number of girls=273243
To simplify the ratio, we can divide both numbers by their greatest common divisor (GCD). The GCD of 273 and 243 is 9:
273÷9243÷9=30.3327
However, we need to express this in whole numbers. We can multiply both parts by 3 to eliminate the decimal:
New ratio=3027 or 30:27
This can be further simplified by dividing both parts by 3:
Final new ratio=109 or 10:9
The new ratio of boys to girls after admitting 12 more girls is 10:9.
Topper's Solved these Questions
- RATIO AND PROPORTION Book:KIRAN PUBLICATIONChapter:RATIO AND PROPORTION Exercise:TYPE-III
Explore 116 Videos
- RATIO AND PROPORTION Book:KIRAN PUBLICATIONChapter:RATIO AND PROPORTION Exercise:TYPE-IV
Explore 30 Videos
- RATIO AND PROPORTION Book:KIRAN PUBLICATIONChapter:RATIO AND PROPORTION Exercise:TEST YOURSELF
Explore 19 Videos
- PROFIT AND LOSS Book:KIRAN PUBLICATIONChapter:PROFIT AND LOSS Exercise:TEST YOURSELF
Explore 23 Videos
- SEQUENCE AND SERIES Book:KIRAN PUBLICATIONChapter:SEQUENCE AND SERIES Exercise:TEST YOURSELF
Explore 20 Videos
Similar Questions
The ratio of the number of boys and girls in a school of 720 students is 7 : 5. How many more girls should be admitted to make the ratio 1 : 1? 90 b. 120 c. 220 d. 240
View SolutionStudy the given pie chart and answer the question that follows
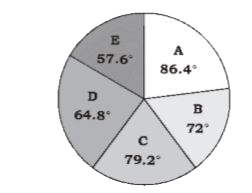
In the ratio of the number of boys to that of girls in school B is 7:6 and the ratio of the number of boys to that of girls in school D is 4 :5 then what is the ratio of the number of boys in B to that of girls in D
View Solution
Knowledge Check
The ratio of the numbers of boys and girls of a school with 504 students is 13:11. What will be the new ratio if 12 more girls are admitted?
A91:81B81:91C9:10D10:9The ratio of the number of boys to the number of girls in a school of 640 students is 5:3. If 30 more girls are admitted in the school, then how many more boys should be admitted so that the ratio of boys to that of girls, becomes 14:9?
A25B15C20D30The ratio of the number of boys to the number of girls in a school of 640 students, is 5:3. If 30 more girls are admitted in the school, then how many more boys should be admitted so that the ratio of boys to that of the girls, becomes 14:9.
A20B15C20D30
The ratio of number of boys and girls in a school of 720 students is 7:5. How many more girls should be admitted to make the ratio 1:1?
View SolutionThe ratio of number of boys to the number of girls in a school of 432 pupils is 5: 4. When some new boys and girls are admitted, the number of boys increase by 12 and the ratio of the boys to girls changes to 7:6. Find the number of new girls admitted.
View SolutionThe ratio of number of boys to the number of girls in a school of 432, pupils is 5:4. When some new boys and girls are admitted, the number of boys increase by 12 and the ratio of the boys to girls changes to 7:6. The number of new girls admitted is:
View SolutionThe ratio of number of boys to the number of girls in a school of 432, pupils is 5:4. When some new boys and girls are admitted, the number of boys increase by 12 and the ratio of the boys to girls changes to 7:6. The number of new girls admitted is:
View SolutionIn a school , the number of boys and girls are in Ratio 5:3 Some new boys and girls took admission in the school in the ratio 5:7 Now , the total number of students in the school are 1200 and the ratio of total number of boys and girls become 7:5 Find the number of students initially .
View Solution