The average marks obtained by a student in 6 subjects is 88. On subsequent verification it was found that the marks obtained by him in a subject was wrongly copied as 86 Instead of 68. The correct average of the marks obtained by him is:
87
85
84
86
The correct Answer is:B
To solve the problem step by step, we will first find the total marks obtained by the student based on the average, then adjust for the error in the marks, and finally calculate the new average.
Step 1: Calculate the total marks based on the average.
The average marks obtained by the student in 6 subjects is given as 88.
To find the total marks, we can use the formula for average:
Average=Total MarksNumber of Subjects
Rearranging this formula gives us:
Total Marks=Average×Number of Subjects
Substituting the values:
Total Marks=88×6=528
Step 2: Identify the error in the marks.
It was found that one subject's marks were wrongly recorded as 86 instead of 68.
To find the correct total marks, we need to adjust for this error:
Correct Total Marks=Total Marks−Wrong Marks+Correct Marks
Correct Total Marks=528−86+68
Calculating this gives:
Correct Total Marks=528−86+68=510+68=510
Step 3: Calculate the new average.
Now that we have the correct total marks, we can find the new average:
New Average=Correct Total MarksNumber of Subjects
New Average=5106
Calculating this gives:
New Average=85
Final Answer:
The correct average of the marks obtained by the student is 85.
---
The average marks obtained by the student in 6 subjects is given as 88.
Average=Total MarksNumber of Subjects
Total Marks=Average×Number of Subjects
Total Marks=88×6=528
It was found that one subject's marks were wrongly recorded as 86 instead of 68.
Correct Total Marks=Total Marks−Wrong Marks+Correct Marks
Correct Total Marks=528−86+68
Correct Total Marks=528−86+68=510+68=510
Now that we have the correct total marks, we can find the new average:
New Average=Correct Total MarksNumber of Subjects
New Average=5106
New Average=85
The correct average of the marks obtained by the student is 85.
Topper's Solved these Questions
- BOOK - KIRAN PUBLICATIONCHAPTER - AVERAGEEXERCISE - TYPE-VI13videos
AVERAGE
- BOOK - KIRAN PUBLICATIONCHAPTER - AVERAGEEXERCISE - TYPE-VII22videos
AVERAGE
- BOOK - KIRAN PUBLICATIONCHAPTER - AVERAGEEXERCISE - TYPE-IV10videos
AVERAGE
- BOOK - KIRAN PUBLICATIONCHAPTER - ARITHMETICAL PROBLEMSEXERCISE - TYPE-X32videos
ARITHMETICAL PROBLEMS
- BOOK - KIRAN PUBLICATIONCHAPTER - BOAT AND STREAMEXERCISE - TEST YOURSELF10videos
BOAT AND STREAM
Similar Questions
The average marks obtained by a student in 9 subjects is 98. On subsequent verification it was found that the marks obtained by him in a subject was wrongly copied as 86 instead of 68. The correct average of the marks obtained by him is:
एक छात्र द्वारा 9 विषयों में प्राप्त औसत अंक 98 है | फिर से जांच करने पर यह पाया गया कि उसके द्वारा एक विषय में प्राप्त किये गए अंक 68 के बजाय 86 लिखे गए थे | उसके द्वारा प्राप्त अंकों का सही औसत है :View SolutionThe table given below shows the marks obtained by 6 students in 3 subjects.
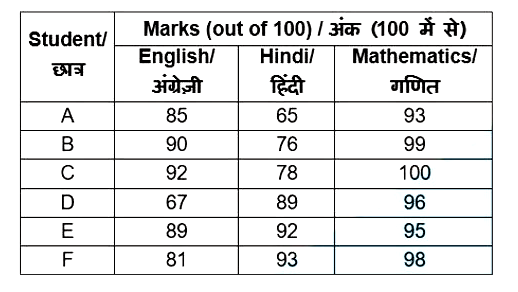
In which subject the total marks obtained by the given six students are highest?View Solution
Knowledge Check
- Question 1 - Select One
What are the average marks obtained by the student?
A57B63C80D48 - Question 2 - Select One
What is the average of marks obtained by all the students in subject B?
A71.4B73.14C107.14D114.07 - Question 3 - Select One
The average marks of 18 students in an examination was 60. It was later found that the marks of one student had been wrongly entered as 63 instead of 36. The correct average is:
A59B59.5C58D58.5
The table given below shows the marks obtained by 6 students in 3 subjects.
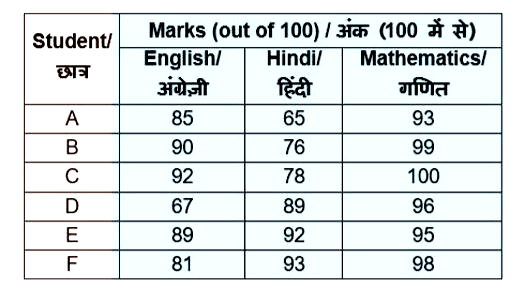
Who has obtained the highest total marks in the given three subjects?View SolutionThe average marks of 50 students in an examination was 65. It was later found that the marks of one student had been wrongly entered as 83 instead of 38. The correct average is?
View Solution
The above bar graph show the marks obtained by a students in an examination. What is the average marks obtained by the student ?View SolutionWhat is the average percentage of marks obtained by all the students in subject C and D together.
View SolutionThe average of marks of 14 student was calculated as 71. But It was later found that the marks of one student had been wrongly entered as 42 instead of 56 and of another as 74 instead of 32. The correct average is:
View Solution
