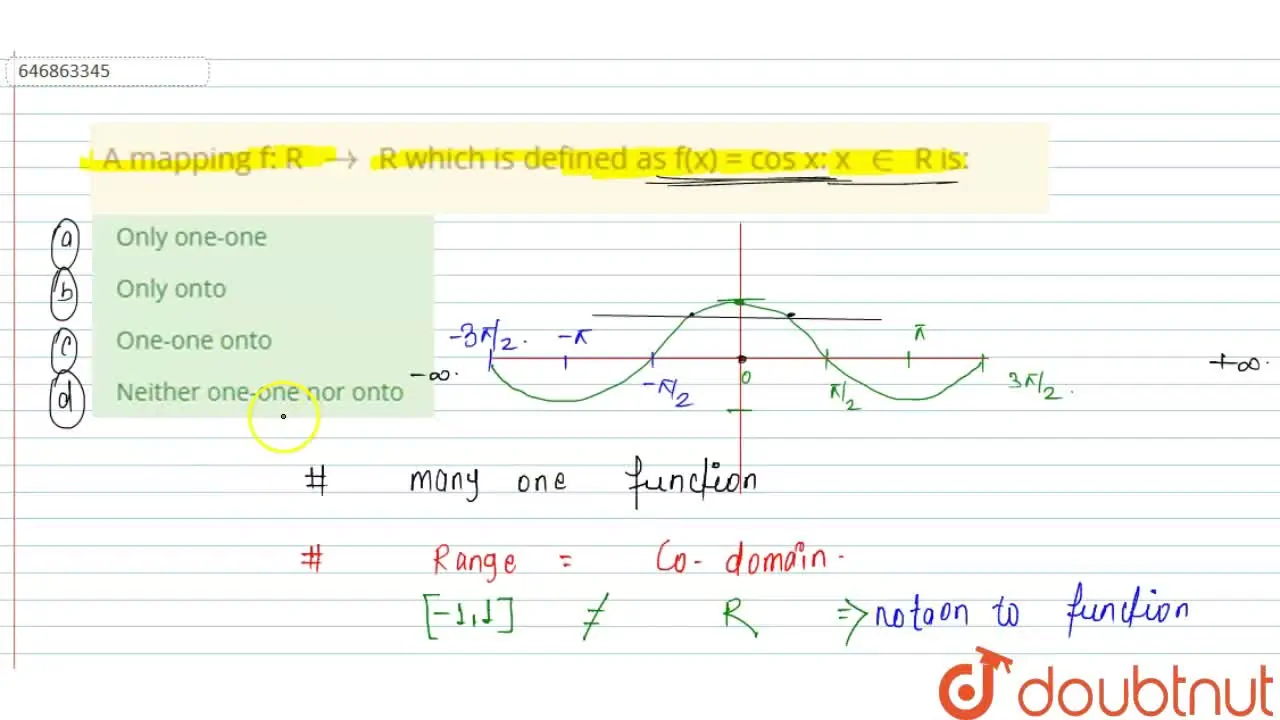
A mapping f: R → R which is defined as f(x) = cos x: x ∈ R is:
Only one-one
Only onto
One-one onto
Neither one-one nor onto
The correct Answer is:D
Step by step video, text & image solution for A mapping f: R rarr R which is defined as f(x) = cos x: x in R is: by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
81. A mapping f : R -> R which is defined as f(x) = cos x; x∈R is: (a) oniy one-one (b) only onto (C) one-one onto (d) Neither one-one nor onto
View SolutionMapping f:R→R which is defined as f(x)=cosx,x∈R will be
View SolutionIf f: R → R be defined by f(x)=ex and g:R → R be defined by g(x) =x2 the mapping gof : R → R be defined by (gof)(x) =g[f(x)] ∀x∈R then
View SolutionThe function f:R→R defined as f(x)=x3 is:
View SolutionIf : R→ R defined by f(x)=x|x|, then f(x) is
View SolutionLet f:R→R, be defined as f(x)=ex2+cosx, then f is a
View SolutionThe function f:R→R is defined by f(x)=3−x
View SolutionThe function f:R→R is defined by f(x)=cos2x+sin4x for x∈R. Then f(R) is
View SolutionThe function f:R→R be defined by f(x)=2x+cosx then f
View SolutionIf f:R→R is defined by f(x)=3x+2, define f [f(x)].
View Solution