How many zeroes are there in 80! ?
16
18
19
20
The correct Answer is:C
To find the number of trailing zeros in 80!, we need to determine how many times 10 is a factor in the numbers from 1 to 80. Since 10 is made up of 2×5, and there are generally more factors of 2 than 5 in factorials, we only need to count the number of times 5 is a factor in 80!.
1. Identify the formula for counting trailing zeros:
The number of trailing zeros in n! can be found using the formula:
Number of zeros=⌊n5⌋+⌊n52⌋+⌊n53⌋+…
until 5k>n.
2. Calculate for n=80:
- First, calculate ⌊805⌋:
⌊805⌋=⌊16⌋=16
- Next, calculate ⌊8025⌋:
⌊8025⌋=⌊3.2⌋=3
- Finally, calculate ⌊80125⌋:
⌊80125⌋=⌊0.64⌋=0
3. Sum the results:
Now, add the results from the calculations:
16+3+0=19
4. Conclusion:
Therefore, the number of trailing zeros in 80! is 19.
Final Answer:
The number of trailing zeros in 80! is 19.
The number of trailing zeros in n! can be found using the formula:
Number of zeros=⌊n5⌋+⌊n52⌋+⌊n53⌋+…
until 5k>n.
- First, calculate ⌊805⌋:
⌊805⌋=⌊16⌋=16
- Next, calculate ⌊8025⌋:
⌊8025⌋=⌊3.2⌋=3
- Finally, calculate ⌊80125⌋:
⌊80125⌋=⌊0.64⌋=0
Now, add the results from the calculations:
16+3+0=19
Therefore, the number of trailing zeros in 80! is 19.
The number of trailing zeros in 80! is 19.
Topper's Solved these Questions
Similar Questions
Knowledge Check
How many zeroes are there in ten lakh?
A6B7C8D4How many zeroes are there in the product48×125×125×625×32×128?
Aa)10Bb)12Cc)14Dd)20How many zeroes are there in product 46×1510?
Aa)10Bb)12Cc)11Dd)6
Answer the following questions below :
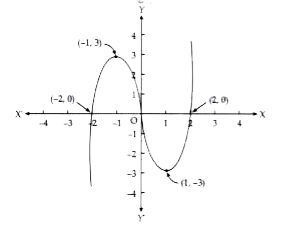
How many zeroes are there for the polynomial ?
View SolutionAnswer the following questions below :
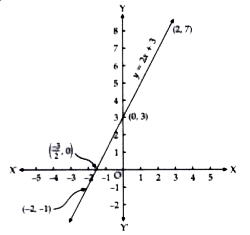
How many zeroes are there for the polynomial (shape of the wire)?
View Solution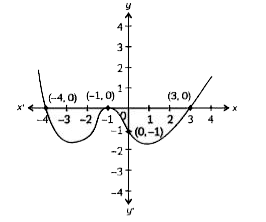
The graph of y = f(x) is given. How many zeroes are there of f(x)?
View SolutionRead the following text and answer the following questions on the basis of the same:
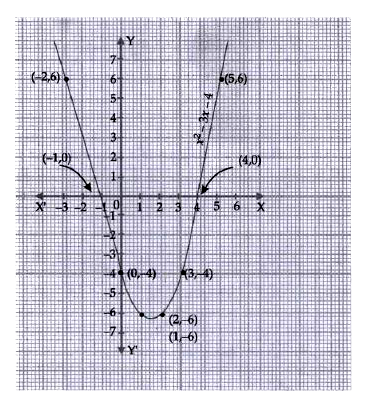
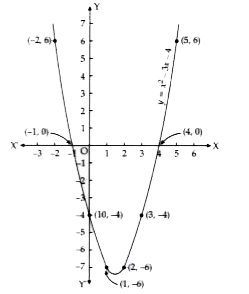
How many zeroes are there for the polynomial?
View SolutionHow many zeroes are there at the end of product 35×27×52?
View Solution