For a parabola , it's vertex is at 2 units from the origin. focus is at distances of 4unit from the origin. A pair of tangents are drawn from origin to the parabola which meet it at P and Q . Find the area of ΔOPQ (O:origin)
16
32
16√2
32√2
The correct Answer is:A
To solve the problem step by step, we need to find the area of triangle OPQ formed by the tangents drawn from the origin to the parabola.
Step 1: Determine the vertex and focus of the parabola
The vertex of the parabola is given to be at a distance of 2 units from the origin, which we can place at the point V(2,0). The focus is at a distance of 4 units from the origin, which we can place at the point F(4,0).
Step 2: Write the equation of the parabola
The standard form of a parabola that opens to the right is given by:
y2=4a(x−h)
where (h,k) is the vertex and a is the distance from the vertex to the focus. Here, h=2, k=0, and a=2 (since the distance from the vertex at (2,0) to the focus at (4,0) is 2 units).
Thus, the equation of the parabola becomes:
y2=4⋅2(x−2)⟹y2=8(x−2)
Step 3: Find the directrix of the parabola
The directrix of a parabola is given by the equation:
x=h−a
Substituting h=2 and a=2:
x=2−2=0
So, the directrix is the line x=0 (the y-axis).
Step 4: Find the points of tangency (P and Q)
The tangents drawn from the origin (0, 0) to the parabola will meet the parabola at points P and Q. The coordinates of points P and Q can be found using the equation of the parabola.
Using the equation y2=8(x−2), we can find the points of tangency. The points of tangency can be derived from the condition for tangents from a point to a parabola. The equation for the tangents from the point (0,0) to the parabola y2=8(x−2) can be derived as:
y=mx(where m is the slope)
Substituting y=mx into the parabola's equation:
(mx)2=8(x−2)
m2x2=8x−16
Rearranging gives:
m2x2−8x+16=0
The discriminant of this quadratic must be zero for the tangents to touch the parabola:
D=b2−4ac=(−8)2−4(m2)(16)=64−64m2=0
Thus, m2=1 implies m=1 or m=−1.
Step 5: Find coordinates of points P and Q
For m=1:
y=x⟹y2=8(x−2)⟹x2=8(x−2)
Solving gives:
x2−8x+16=0⟹(x−4)2=0⟹x=4
So, y=4 giving point P(4,4).
For m=−1:
y=−x⟹y2=8(x−2)⟹x2=8(x−2)
This gives the same x-coordinate:
x=4⟹y=−4 giving point Q(4,−4).
Step 6: Calculate the area of triangle OPQ
The coordinates of points are:
- O(0,0)
- P(4,4)
- Q(4,−4)
The area A of triangle OPQ can be calculated using the formula:
A=12×base×height
Here, the base PQ is the vertical distance between P and Q, which is:
|4−(−4)|=8
The height from O to line PQ (which is vertical) is the horizontal distance from O to line x=4, which is:
4
Thus, the area is:
A=12×8×4=16
Final Answer
The area of triangle OPQ is 16.
The vertex of the parabola is given to be at a distance of 2 units from the origin, which we can place at the point V(2,0). The focus is at a distance of 4 units from the origin, which we can place at the point F(4,0).
The standard form of a parabola that opens to the right is given by:
y2=4a(x−h)
where (h,k) is the vertex and a is the distance from the vertex to the focus. Here, h=2, k=0, and a=2 (since the distance from the vertex at (2,0) to the focus at (4,0) is 2 units).
y2=4⋅2(x−2)⟹y2=8(x−2)
The directrix of a parabola is given by the equation:
x=h−a
Substituting h=2 and a=2:
x=2−2=0
So, the directrix is the line x=0 (the y-axis).
The tangents drawn from the origin (0, 0) to the parabola will meet the parabola at points P and Q. The coordinates of points P and Q can be found using the equation of the parabola.
y=mx(where m is the slope)
Substituting y=mx into the parabola's equation:
(mx)2=8(x−2)
m2x2=8x−16
Rearranging gives:
m2x2−8x+16=0
The discriminant of this quadratic must be zero for the tangents to touch the parabola:
D=b2−4ac=(−8)2−4(m2)(16)=64−64m2=0
Thus, m2=1 implies m=1 or m=−1.
For m=1:
y=x⟹y2=8(x−2)⟹x2=8(x−2)
Solving gives:
x2−8x+16=0⟹(x−4)2=0⟹x=4
So, y=4 giving point P(4,4).
y=−x⟹y2=8(x−2)⟹x2=8(x−2)
This gives the same x-coordinate:
x=4⟹y=−4 giving point Q(4,−4).
The coordinates of points are:
- O(0,0)
- P(4,4)
- Q(4,−4)
A=12×base×height
Here, the base PQ is the vertical distance between P and Q, which is:
|4−(−4)|=8
The height from O to line PQ (which is vertical) is the horizontal distance from O to line x=4, which is:
4
Thus, the area is:
A=12×8×4=16
The area of triangle OPQ is 16.
Similar Questions
Let a parabola P be such that its vertex and focus lie on the positive x- axis at a distance 2 and 4 units from the origin , respectively .If tangents are drawn from O(0,0) to the parabola P which meet P at S and R , then the area (in sq .units)of ΔSOR is equal to :
View SolutionThe distance of the point P(2,4) from the origin is :
View Solution
Knowledge Check
The distance of the point p(3,4) from the origin is
A3B4C7D5
The angle between the tangents drawn from the origin to the parabola y2=4a(x−a) is
View SolutionThe angle between the tangents drawn from the origin to the paraboala y2=4a(x−a), is
View SolutionThe tangents from the origin to the parabola y2+4=4x are inclined at
View SolutionO is the origin and B is a point on the x-axis at a distance of 2 units from the origin. Match the following lists.
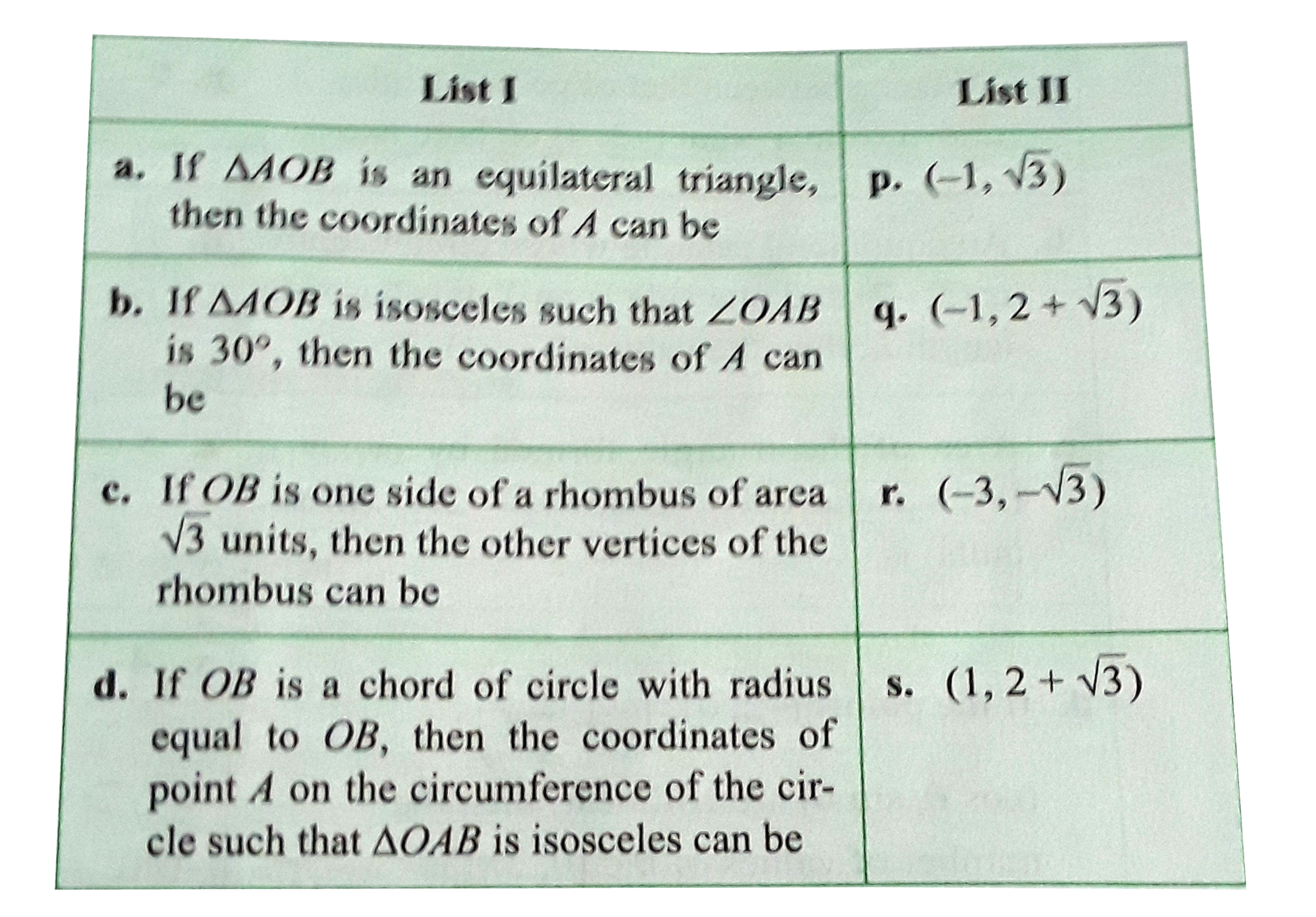
View SolutionFind th equation of the plane which is at distance of 8 units from origin and the perpendicular vector from origin to this plane is (2ˆi+ˆj−2ˆk).
View SolutionTwo tangents OP & OQ are drawn from origin to the circle x2+y2−x+2y+1=0 then the circumcentre of ΔOPQ is
View SolutionFind the distance of the point P (4, 2) from origin.
View Solution