The tension at any point of the string AB will be same if
√T21+T222
⎛⎜ ⎜⎝√T21−T222⎞⎟ ⎟⎠
⎛⎜ ⎜⎝√T21+T222⎞⎟ ⎟⎠
none of the above.
The correct Answer is:C
Referring to the free budy diagrams of the bob we obtain,
T1−mg=ma
⇒T1=m(g+a) ...(1)
mg−T2=ma
⇒T2=m(g−a) ...(2)
When the cage moves horizontally with an acceleration a, the tension be T.
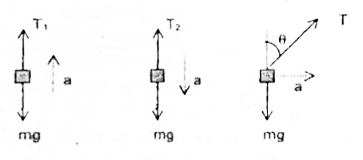
From the free body diagram, T sinθ=ma
And Tcosθ−mg=0
⇒(Tsinθ)2+(T−cosθ)2=(ma)2+(mg)2
⇒T2=m2(g2+a2) ..(3)
From (1) and (2)
(T1m)2+(T2m)2=(g+a)2+(g−a)2
⇒T21+T222=(g2+a2)m2 ...(4)
Equations (3) and (4), we obtain
T2=12(T21+T22)⇒T=√T21+T222
Similar Questions
The tension T in the string shown in figure is

View SolutionIn the following figure, if the tension in the string AB is 51N, then the tension in the string BC will be :-
View SolutionFind the tension in the string AB loaded with weight W at the middlw, when AB is horizontal:

View Solution
A rod is supported horizontally by means of two strings of equal length as shown in figure. If one of the string is cut. Then tension in other string at the same instant will.
View SolutionIn the given figure tension at the mid-point of string −2. If mass of string−1 is 6kg and mass of string−2 is 4kg.

View SolutionThe length , radius , tension and density of stringA are twice the same parameters of string B. Find the ratio of fundamental frequency of B to the fundamental frequency of A.
View SolutionThe tension, length, diameter and density of a string B are double than that of another string A. Which of the following overtones of B is same as the fundamental frequency of A?
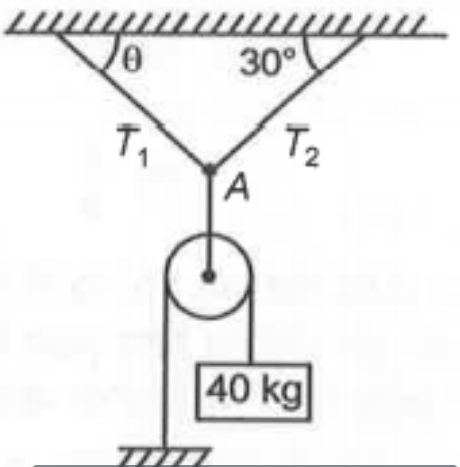
View SolutionFor the arrangement shown in figure, angle θ for which tension T1 in the left string will be minimum is (Pulley and strings are ideal)
View SolutionThe tension in the string connected between blocks is

View Solutionn number of waves are produced on a string in 0.5 s. Now, the tension in the string is doubled (Assume length and radius constant), the number of waves produced in 0.5s for the same harmonic will be
View Solution
