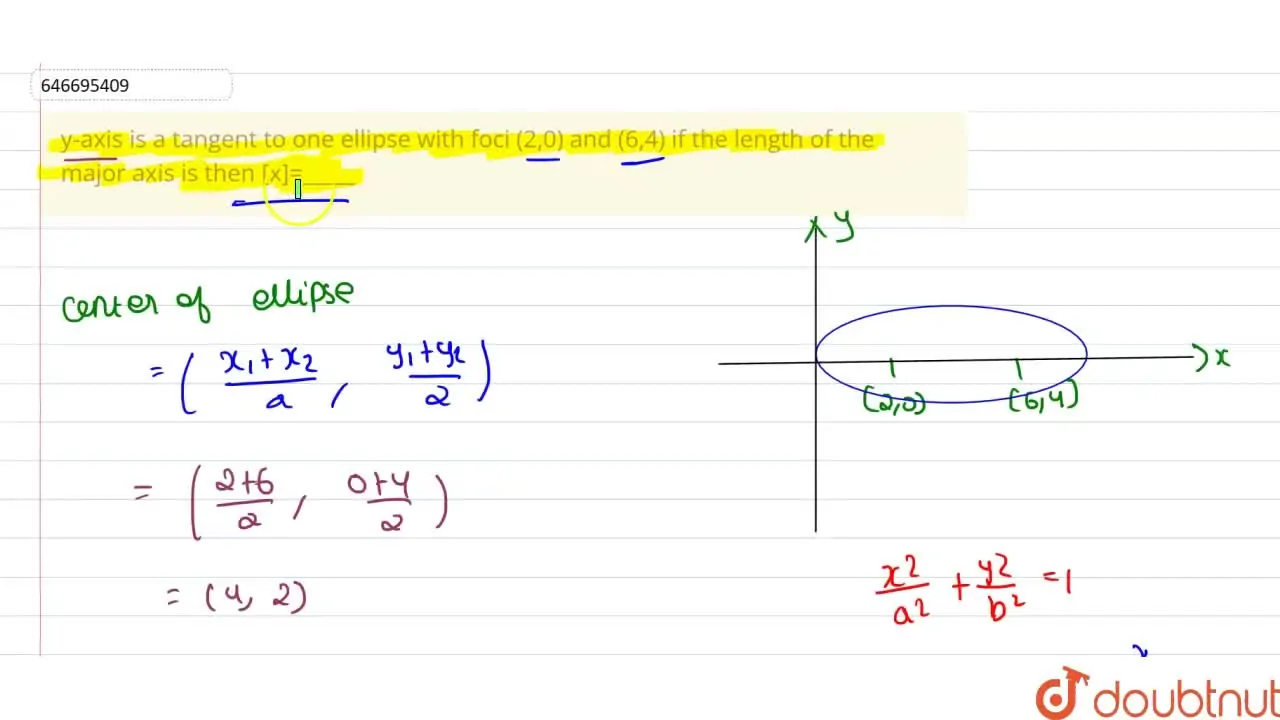
y-axis is a tangent to one ellipse with foci (2,0) and (6,4) if the length of the major axis is then [x]=_____
Step by step video & image solution for y-axis is a tangent to one ellipse with foci (2,0) and (6,4) if the length of the major axis is then [x]=_____ by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
If (0,3+√5) is a point on the ellipse whose foci and (2, 3) and (−2,3), then the length of the semi - major axis is
View SolutionThe equation of ellipse whose foci are (±3,0) and length of semi-major axis is 4 is
View SolutionEquation of the ellipse whose foci are (2,2) and (4,2) and the major axis is of length 10, is
View SolutionProve that if any tangent to the ellipse is cut by the tangents at the endpoints of the major axis at TandT' , then the circle whose diameter is TT' will pass through the foci of the ellipse.
View SolutionFind the equation of the ellipse with centre at the origin, the length of the major axis 12 and one focus at (4, 0).
View SolutionIf the length of the major axis of an ellipse with the equation 5x2+24y2=40 is j and the length of the minor axis of the ellipse is n, then what is the value of j+n ?
View SolutionThe equation of tangent to the ellipse 2x2+3y2=6 which make an angle 30∘ with the major axis is
View SolutionFind the equation of the ellipse, whose length of the major axis is 20 and foci are (0,±5).
View SolutionThe equation of the ellipse having foci (1,0),(0,-1) and minor axis of length 1 is ……
View SolutionThe equation of the ellipse, the co-ordinates of whose foci a re (±√3,0) and length of the semi-major axis as 2 is
View Solution