A rectangular plate in the x-y plane is shown in the figure. A force ¯¯¯F=2ˆi−3ˆj N is applied at point B. Find the torque of ¯¯¯F about
(a) the origin O
(b) the point C
(c) X-axis, Y-axis and Z-axis.
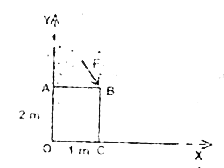
(a) Torque of →F about O : →r=O→B=ˆi+2ˆj
τ0=→r×→F=(ˆi+2ˆj)×(2ˆi−3ˆj)=−7ˆkN−m
(b) τC=¯¯¯¯¯¯CB×→F=2ˆj×(2ˆi−3ˆj)=−4ˆkN−m
(c) τXX.=→τ0.ˆi=(−7ˆk).ˆi=0
τYY=→τ0.ˆj=(−7ˆk).ˆj=0
τZ=→τ0.ˆk=(−7ˆk).ˆk=−7N−m
Similar Questions
The torque of a force F=2ˆi−3ˆj+5ˆk acting at a point whose position vector r=3ˆi−3ˆj+5ˆk about the origin is
View SolutionThe torque of a force F=−2ˆi+2ˆj+3ˆk acting on a point r=ˆi−2ˆj+ˆk about origin will be
View SolutionFind the length of the foot of perpendicular drawn from the point P(a, b, c) on x-axis, y-axis and
z-axis respectively.
View SolutionA force F=2ˆi+ˆj−ˆk acts at point A whose position vector is 2ˆi−ˆj. Find the moment of force F about the origin.
View SolutionThe torque of force F=−3ˆi+ˆj+5ˆk acting on a point r=7ˆi+3ˆj+ˆk about origin will be
View SolutionFind the torque of the force →F=(2ˆi−3ˆj+4ˆk) N acting at the point →r=(3ˆi=2ˆj+3ˆk)m about the origion.
View SolutionThe torque of a force F=−6ˆi acting at a point r=4ˆj about origin will be
View SolutionFind the angle of vector →a = 6ˆi + 2ˆj - 3ˆk with x -axis.
View SolutionThe line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
the co-ordinates of A and B.
View SolutionFind the torque of a force F=(2ˆi+ˆj−3ˆk)N about a point O. The position vector of point of application of force about O is r=(2ˆi+3ˆj−ˆk)m.
View Solution
