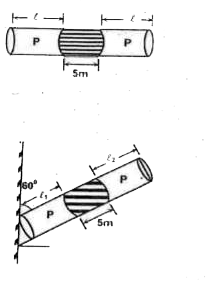
A thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal ends containing air at the same pressure P. When the tube is held at an angle of $60^{\circ}$ with the vertical direction, the length of the air column above and below the mercury column are $46 c m$ and $44.5 c m$ respectively. Calculate the pressure P in centimetre of mercury. (The temperature of the system is kept at $30^{\circ} C$

Video Solution

Text Solution

Verified by Experts

Length of pipe = $(46 + 44.5 + 5) c m = 95.5 c m$
Suppose the pressure in both the columns of air in the horizontal the pressure in both the columns of air in the horizontal position be P .
$∴ l = (46 + 44.5) / 2 = 45.25 c m$
When the tube is inclined , let $P_{1} and P_{2}$ be the pressure and $l_{1} and l_{2}$ be the lengths of lower and upper air columns respectively .
Using Boyle.s law ,
$P A l_{1} = P_{1} A l_{2} = P_{2} A l_{2}$ (where A is area of cross - section of the tube )
Using Boyle.s law
$P A l = P_{2} A l_{2}$
$P_{1} = P_{2} + + ρ H_{g} g . l_{g} {cos 60}^{\circ}$
$(P_{2} + ρ H_{g} g l_{H g} {cos 60}^{\circ}) l_{1} = P_{2} l_{2}$ [ From equaton (1)]
$P_{2} = \frac{l_{H g} \times ρ_{H g} g \times l_{1}}{2 (l_{2} - l_{1})}$
$P = \frac{l_{H g} \times ρ_{H g} \times g \times l_{1} l_{2}}{2 (l_{2} - l_{1}) \times l} P a = \frac{l_{H g} \times l_{1} \times l_{2}}{2 (l_{2} - l_{1}) \times l}$ cm of Hg column
$= 75.4$ cm of Hg column

Updated on:21/07/2023

A thin tube of uniform cross-section is sealed at both ends. It lies horizontally, the middle 5 cm containing mercury and the two equal end containing air at the same pressure P. When the tube is held at an angle of $60^{\circ}$ with the vetical direction, the length of the air column above and below the mercury column are 46 cm and 44.5 cm respectively. Calculate the pressure P in centimeters of mercury. (The temperature of the system is kept at $30^{\circ} C$ ).
A $75.4$
B $45.8$
C $67.5$
D $89.3$