A water jet directed by firemen must reach a window 40 m above the ground , from a nozzle of diameter 3 cm discharging 30 kg/s .Determine the largest distance from the building where a fireman can stand and still reach the jet into the window .
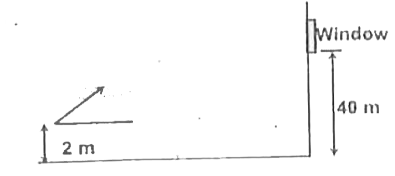
Taking O as the origin of coordinate system . The equation of the water jet is
y=xtanα−gx22u2sec2α
Here, y = 38 (constant )
⇒38=xtanα−gx22u2sec2α
Differentiating with respect to α , we get
0=dxdαtanα+xsec2α
Differentiating with respect to α , we get
0dxdαtanα+xsec2α−g2u2{2xdxdαsec2α+x22sec2α.tanα}
Now , for x to be maximum , dxdα=0
or tanα=u2gx
It can be shown that his value of α gives the maximum for x
y=xu2gx−gx22u2(1+u4g2x2)
⇒x2max=2u2g(u22g−y)
Now , π4(3×10−2)2×1×103×u=30
⇒u=12009πms=42.44 m/s
Putting this value of u and y = 38 m ew get Xmax=137m

Similar Questions
A ladder 25 m long reaches a window of a building 20 m above the ground. Determine the distance of the foot of the ladder from the building.
View SolutionA ladder 25m long reaches a window of a building 20m above the ground. Determine the distance of the foot of the ladder from the building.
View SolutionA ladder 10m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from base of the wall.
View SolutionA ladder, 25 m long reaches a window of building 20 m, above the ground. The distance of the foot of the ladder from the building.
View SolutionA ladder 17m long reaches a window of a building 15m above the ground. Find the distance of the foot of the ladder from the building.
View SolutionA 15 m long ladder reached a window 12 m highfrom the ground on placing it against a wall at a distance a. Find the distance of the foot of theladder from the wall.
View SolutionThe foot of a ladder is 6m away from a wall and its top reaches a window 8m above the ground. If the ladder is shifted in such a way that its foot is 8m away from the wall, to what height does its tip reach?
View SolutionA hill is 500m high. Supplies are to be sent across the hill using a canon that can hurl packets at a speed of 125m/s over the hill . The canon is located at a distance of 800m from the foot to hill and can be moved on the ground at a speed of 2m/s , so that its distance from the hill can be adjusted. What is the shortest time in which a packet can reach on the ground across the hill ? Take g=10m/s2.
View SolutionThe foot of a ladder is 6 m away from a wall and its top reaches a window 8 m above the ground. If the ladder is shifted in such a way that its foot is 8 m away from the wall, to what height does its top reach?
View SolutionA hill is 500m high. Supplies are to be across the hill using a canon that can hurl packets at a speed of 125m/s over the hill . The canon is located at a distance of 800m from the foot to hill and can be veoved on the ground at a speed of 2 m//s , so that its distance from the hill can be adjusted. What is the shortest time inwhich a pachet can reach on the ground across the hill ? Taje g=10m/s2.
View Solution
