A body weighs W1 in a liquid of density ρ1 and W2 in a liquid of density ρ2. What is the weight of body in a liquid of density ρ3?
To solve the problem, we need to determine the weight of a body in a liquid of density ρ3, given its weights in two other liquids of densities ρ1 and ρ2.
1. Understand the Weight in a Liquid:
The weight of a body when submerged in a liquid is given by:
W′=W−FB
where W is the actual weight of the body and FB is the buoyant force acting on the body. The buoyant force can be expressed as:
FB=ρliquid⋅V⋅g
where V is the volume of the body and g is the acceleration due to gravity.
2. Relate Volume to Weight:
The volume V of the body can be expressed in terms of its weight and density:
V=Wρ0⋅g
where ρ0 is the density of the body.
3. Weight in Liquid of Density ρ1:
For the first liquid with density ρ1:
W1=W−ρ1⋅V⋅g
Substituting for V:
W1=W−ρ1⋅(Wρ0⋅g)⋅g
Simplifying gives:
W1=W−ρ1Wρ0
Rearranging yields:
W1=W(1−ρ1ρ0)
4. Weight in Liquid of Density ρ2:
Similarly, for the second liquid with density ρ2:
W2=W−ρ2⋅V⋅g
Following the same steps as above:
W2=W(1−ρ2ρ0)
5. Set Up the Equations:
We now have two equations:
W1=W(1−ρ1ρ0)(1)
W2=W(1−ρ2ρ0)(2)
6. Subtract the Two Equations:
Subtracting equation (2) from (1):
W1−W2=W(ρ2−ρ1ρ0)
Rearranging gives:
W=(W1−W2)⋅ρ0ρ2−ρ1(3)
7. Weight in Liquid of Density ρ3:
For the liquid of density ρ3:
W3=W(1−ρ3ρ0)
Substituting W from equation (3):
W3=(W1−W2)⋅ρ0ρ2−ρ1(1−ρ3ρ0)
8. Final Expression:
Simplifying gives:
W3=(W1−W2)⋅(ρ0−ρ3)ρ2−ρ1
Final Answer:
Thus, the weight of the body in a liquid of density ρ3 is given by:
W3=W2+(W1−W2)⋅(ρ3−ρ1)ρ2−ρ1
The weight of a body when submerged in a liquid is given by:
W′=W−FB
where W is the actual weight of the body and FB is the buoyant force acting on the body. The buoyant force can be expressed as:
FB=ρliquid⋅V⋅g
where V is the volume of the body and g is the acceleration due to gravity.
The volume V of the body can be expressed in terms of its weight and density:
V=Wρ0⋅g
where ρ0 is the density of the body.
For the first liquid with density ρ1:
W1=W−ρ1⋅V⋅g
Substituting for V:
W1=W−ρ1⋅(Wρ0⋅g)⋅g
Simplifying gives:
W1=W−ρ1Wρ0
Rearranging yields:
W1=W(1−ρ1ρ0)
Similarly, for the second liquid with density ρ2:
W2=W−ρ2⋅V⋅g
Following the same steps as above:
W2=W(1−ρ2ρ0)
We now have two equations:
W1=W(1−ρ1ρ0)(1)
W2=W(1−ρ2ρ0)(2)
Subtracting equation (2) from (1):
W1−W2=W(ρ2−ρ1ρ0)
Rearranging gives:
W=(W1−W2)⋅ρ0ρ2−ρ1(3)
For the liquid of density ρ3:
W3=W(1−ρ3ρ0)
Substituting W from equation (3):
W3=(W1−W2)⋅ρ0ρ2−ρ1(1−ρ3ρ0)
Simplifying gives:
W3=(W1−W2)⋅(ρ0−ρ3)ρ2−ρ1
Thus, the weight of the body in a liquid of density ρ3 is given by:
W3=W2+(W1−W2)⋅(ρ3−ρ1)ρ2−ρ1
Similar Questions
Knowledge Check
The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρA body floats in a liquid A of density ρ1 with a part of it submerged inside liquid while in liquid B of density ρ2 totally submerged inside liquid. The densities ρ1 and ρ2 are related as :
Aρ1=ρ2Bρ1<ρ2Cρ1>ρ2Dnothing can be said
A ball of density ρ is released from deep inside of a liquid of density 2ρ. It will move up
View SolutionA body of density ρ floats with volume V1 of its total volume V immersed in a liquid of density ρ1 and with the remainder of volume V2 immersed in another liquid of density ρ2 where ρ1>ρ2. Find the volume immersed in two liquids. (V1 and V2).
View SolutionA plank of area of cross-section A is half immersed in liquid 1 of density ρ and half in liquid 2 of density 2ρ. What is period of osciallation of the plank if it is slightly depressed downwards?

View SolutionA body of density ρ is immersed in a liquid of density ρL.State condition when the body will (i) float (ii) sink in liquid.
View SolutionA tube U-shaped has a uniform cross-sectionn with arm length l1andl2(l1>l2). Tube has a liquid of density ρ1 filled to a height h. another liquid of density ρ2=(ρ1)14 is poured in arm A. both liquids are immiscible. the length of second liquid that should be poured in A so that first overtone of A is in unisom with fundamental tone of B is:
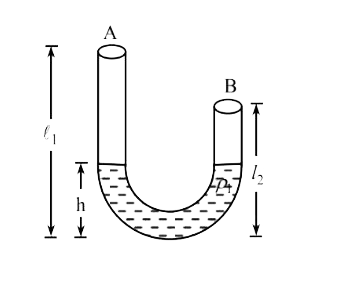
View SolutionAn object of w and density ρ is submerged in liquid of density σ, its apparent weight will be
View Solution
