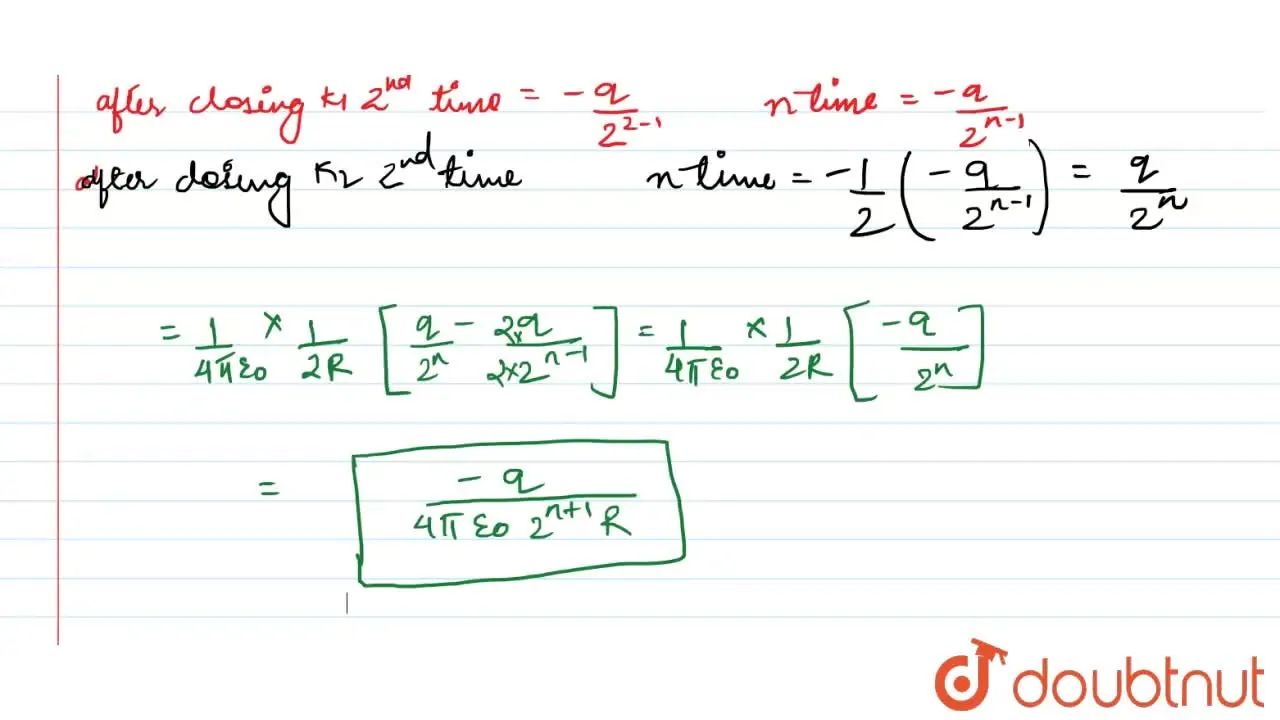
Two concentric shells of radii R and 2R are shown in the figure. Initially a charge q is imparted to the inner shell. After the keys K1&K2 are alternately closed n times each, find the potential difference between the shells.
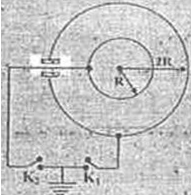
When K1 is closed first time, outer sphere is earthed and its potential becomes zero. Let the charge on it be q1.
V1'= Potential due to charge on inner sphere and that due to charge on outer sphere.
0=14πε0[q2R+q12R]
⇒q1'=q
When K2 is closed first time, the potential V2' on inner sphere becomes zero as it is earthed.
Let the new charge on inner sphere be q2'
0=14πε0q2R+14πε0(−q)(2R)
⇒q2'=q/2
Now when K1 will be closed second time charge on outer sphere will be −q2' i.e. −q/2
Similarly when K1 will be closed nth time, charge on outer sphere will be −q2n−1 as each time.
Charge will be reduced to half the previous value.
After closing K2 nth time charge on inner shell will be negative to half the charge on outer shell.
i.e. (−q2n) and potential on it will be zero.
For potential of outer shell
V0=14πε0(−q/2n)2R+14πε0(q2n−1)2R
V0=q[−1+2]4πε02n+1R=+q4πε02n+1R
Potential difference = V0−V1=q4πε02n+1R−0=q4πε02n+1R
Similar Questions
Two concentric shells of radii R and 2R are shown in (Fig. 3.115). Initially, a charge q is imparted to the inner shells. Now, key K1 is closed and opened and then key K2 is closed and opened. After the keys K1andK2 are alterbately closed n times each, find the potential difference between the shells. Note that finally key K2 remains closed.
 .View Solution
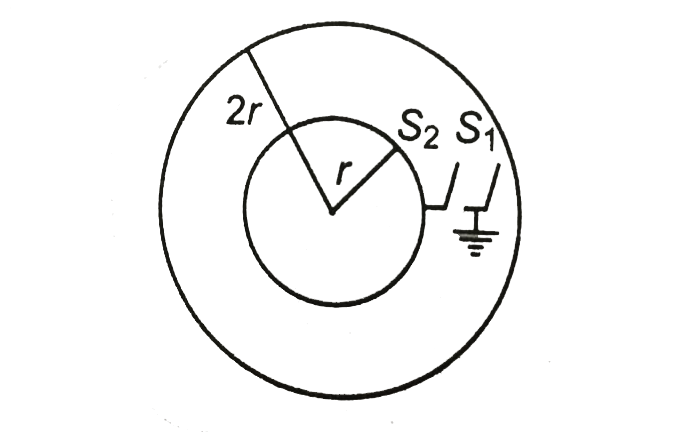
.View SolutionThere are two concentric spherical shell of radii r and 2r. Initially, a charge Q is given to the inner shell and both the switches are open.
The two steps of the above two problems are repeated b times, the potential differences between the shells will beView SolutionIn the given figure there are two concentric shells find potential difference between spheres.
 View Solution
View SolutionThere are two concentric spherical shell of radii r and 2r. Initially, a charge Q is given to the inner shell and both the switches are open.

If switch S1 is closed and then opened, charge on the outer shell will beView SolutionThere are two concentric spherical shells of ratii r and 2r. Intially a charge Q is given to the inner shell. Now switch S1is closed and S2 is opned and the process is repeated n times for both the keys altermatively. Find the final potential between the shells.
 View Solution
View SolutionTwo concentric conducting shells of radii R and 2R are having charges Q and -2Q respectively.In a region r
View SolutionTwo thin conducting shells of radii R and 3R are as shown in the fig. External shell carries a charge +Q and inner shell neutral. The inner shell is earthed with the help of a switch S. Then
 View Solution
View SolutionThere are two concentric spherical shell of radii r and 2r. Initially, a charge Q is given to the inner shell and both the switches are open.

Now, S2 is closed and opened. The charge flowing through the swithc S2 in the process isView Solutionthere are two concentric metallic shells of radii R and 2R, if outer shell is given charge q1 , then charge on surface of inner shell will be
View SolutionThere are two concentric conducting spherical shells of radius rA and rB respectively (rB=2rA). If the inner shell is given a charge Q and outer shell is given a charge 2Q, then the potential difference between the surfaces of the shells will be K=14πε0
View Solution