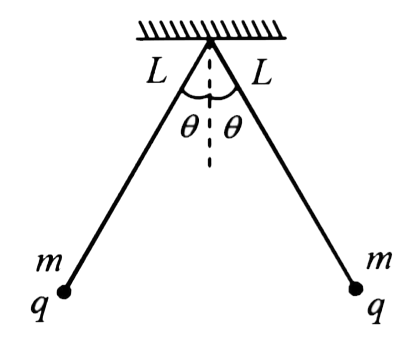
Two similar balls, each of mass m and charge q, are hung from a common point by two silk threads, each of length l. Prove that separation between the ball is x=[q2l2πε0mg]1/3, if θ is small
Find the rate dqdt with which the charge should leak off each sphere if the velocity of approach varies as v=a/√x, where a is a constant.
![Two similar balls, each of mass m and charge q, are hung from a common point by two silk threads, each of length l. Prove that separation between the ball is x=[(q^(2)l)/(2 pi epsilon(0)mg)]^(1//3), if theta is small Find the rate (dq)/(dt) with which the charge should leak off each sphere if the velocity of approach varies as v=a//sqrt(x), where a is a constant.](https://d10lpgp6xz60nq.cloudfront.net/physics_images/BMS_V03_C01_E01_082_Q01.png)
Force of electrostatic repulsion :
F=q24πε0x2
For equilibrium of each sphere :
Tcosθ=mg

Tsinθ=F=q24πε0x2
⇒Fmg=tanθ
⇒q24πε0x2(mg)=tanθ≈sinθ=x2l [∵θ→0]
⇒x=(q2l2πε0mg)1/3 ...(i)
From equation (i)
q2=2πε0mgx3l
Differentiating both sides with respect to time :
(ii) 2qdqdt=2πε0mgl3x2dxdt
Now, dxdt = velocity of approach = v = a√x
(2πε0mgl)1/2.x3/2dqdt=3πε0mglx2(a√x) using equation (i)
⇒dqdt=32a√2πε0mgl
Similar Questions
Two identical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View SolutionTwo small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionThe compound
 is used as
is used as
View SolutionTwo identical small bodies each of mass m and charge q are suspended from two strings each of length l from a fixed point. This whole system is taken into an orbiting artificial satellite, then find the tensio in strings
View SolutionTwo small spheres each of mass m and charge q are tied from the same rigid support with the help of silk threads of length L. They make angle θ with the vertical as shown in the fig. If length L is decreased, then angle θ with the vertical.
View SolutionThree identical small balls, each of mass 0.1 g, are suspended at one point on silk thread having a length of l=20cm . What charges should be imparted to the balls for each thread to form an angle of α=30∘ with the vertical?
View SolutionTwo identical balls , each having a charge q and mass m , are suspended from a common point by two insultating strings each of length L. The balls are held at a separation x and then released. Find
(a) the electric force on each ball
(b) the component of the resultant force on a ball along and perpendicular to string
(c ) the tension in the string
(d) the acceleration of one of the balls. Consider the situation only for the instant just after the release.
View SolutionTwo small balls having equal positive charge Q (coulumb) on each suspended by two insulating strings of equal length L (metre) from a hook fixed to a stand. The whole set-up is taken in a satellite into space where there is no gravity (state of weightlessness). Then tension (newtons) in each string is:
View SolutionTwo identical charged spheres suspended from a common point by two mass-less strings of length l are initially at a distance d ( d<<l) apart because of their mutual repulsion . The charge begins to leak from both the spheres at a constant rate. As a result the charge approach each other with a velocity v. Then as a function of distance x between them .
View Solution
