The space between the two coaxial cylindrical shells of radius R1andR2(R1<R2) is filled by a dielectric substance, whose dielectric constant varies with the distance from the axis as k=A/r3. If the length of the cylindrical shells is l then find the capacitance of the system
To find the capacitance of the coaxial cylindrical shells filled with a dielectric whose dielectric constant varies with distance, we follow these steps:
Step 1: Understand the Geometry and Variables
We have two coaxial cylindrical shells with inner radius R1 and outer radius R2. The dielectric constant k varies with distance r from the axis as k=Ar3, where A is a constant.
Step 2: Electric Field Calculation
The electric field E between the two cylindrical shells can be derived from Gauss's law. The electric field in the region between the cylinders is given by:
E=Q2πϵ0kr
Substituting the expression for k:
E=Q2πϵ0(Ar3)r=Qr22πϵ0A
Step 3: Calculate the Potential Difference V
The potential difference V between the two shells is obtained by integrating the electric field from R1 to R2:
V=−∫R2R1Edr
Substituting the expression for E:
V=−∫R2R1Qr22πϵ0Adr
Step 4: Evaluate the Integral
Calculating the integral:
V=−Q2πϵ0A∫R2R1r2dr
The integral of r2 is:
∫r2dr=r33
Thus,
V=−Q2πϵ0A(R323−R313)=−Q6πϵ0A(R32−R31)
Step 5: Calculate Capacitance C
The capacitance C is defined as:
C=QV
Substituting the expression for V:
C=Q−Q6πϵ0A(R32−R31)=−6πϵ0A1(R32−R31)
Final Result
Thus, the capacitance of the system is:
C=6πϵ0AR32−R31
We have two coaxial cylindrical shells with inner radius R1 and outer radius R2. The dielectric constant k varies with distance r from the axis as k=Ar3, where A is a constant.
The electric field E between the two cylindrical shells can be derived from Gauss's law. The electric field in the region between the cylinders is given by:
E=Q2πϵ0kr
Substituting the expression for k:
E=Q2πϵ0(Ar3)r=Qr22πϵ0A
The potential difference V between the two shells is obtained by integrating the electric field from R1 to R2:
V=−∫R2R1Edr
Substituting the expression for E:
V=−∫R2R1Qr22πϵ0Adr
Calculating the integral:
V=−Q2πϵ0A∫R2R1r2dr
The integral of r2 is:
∫r2dr=r33
Thus,
V=−Q2πϵ0A(R323−R313)=−Q6πϵ0A(R32−R31)
The capacitance C is defined as:
C=QV
Substituting the expression for V:
C=Q−Q6πϵ0A(R32−R31)=−6πϵ0A1(R32−R31)
Thus, the capacitance of the system is:
C=6πϵ0AR32−R31
Similar Questions
The capacitance of two concentric spherical shells of radii R1 and R2(R2>R1) is
View SolutionA cylinder of radius R and length l is made up of substance whose thermal conductivity K varies with the distance x from the axis as K=K1x+K2. Determine the the effective thermal conductivity between the flat faces of the cylinder.
View Solution
Knowledge Check
Space between tow concentric spheres of radii r1andr2 such that r1<r2 is filled with a material of resistivity ρ. Find the resistance between inner and outer surface of the material
 Ar1r2p2Br2−r1r1r2p4πCr1r2r2−r1p4πDnone of these.
Ar1r2p2Br2−r1r1r2p4πCr1r2r2−r1p4πDnone of these.
How does the electric field (E) between the plates of a charged cylindrical capacitor vary with the distance r from the axis of the cylinder ?
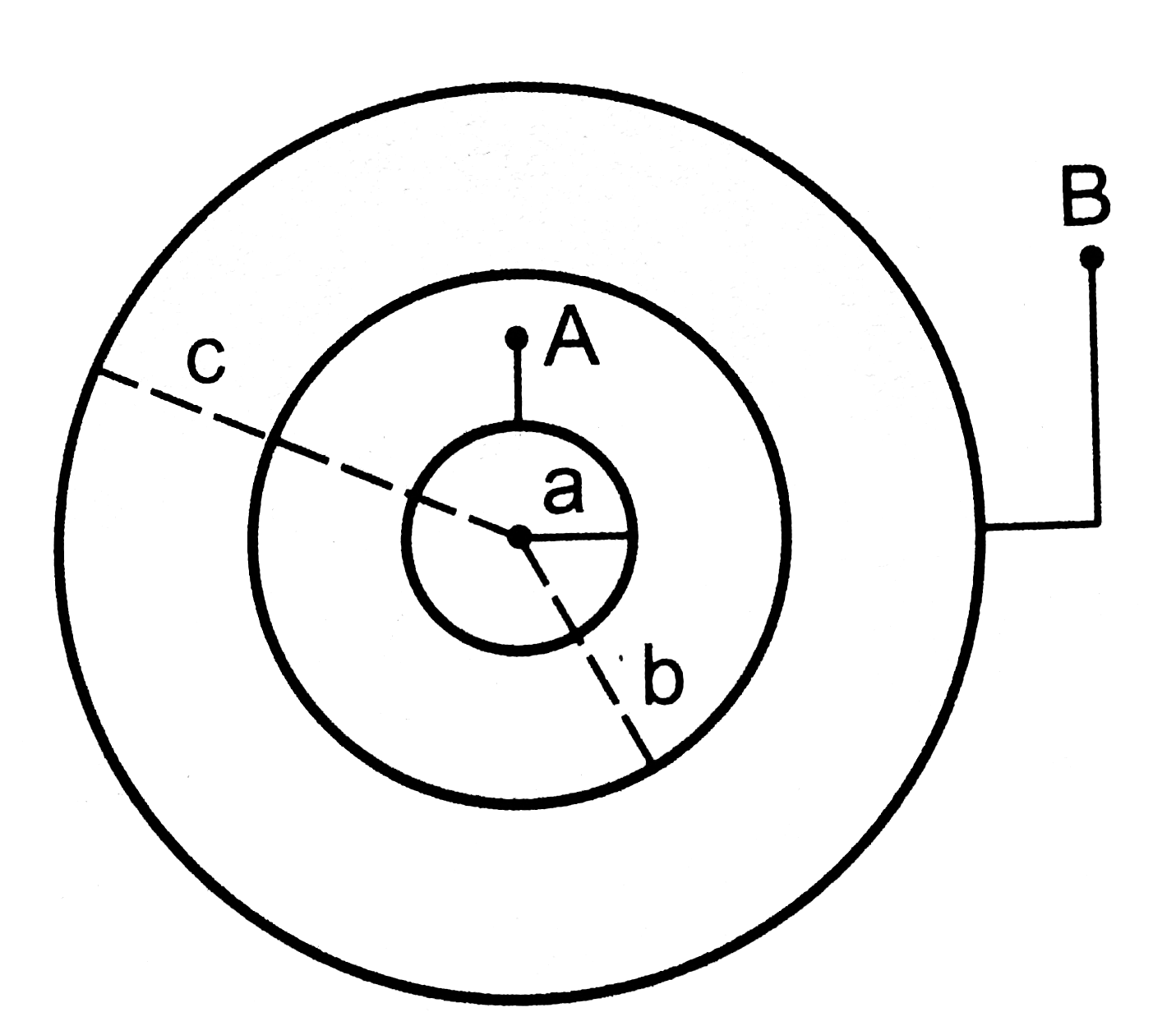
View SolutionSuppose the space between the two inner shells of the previous problum is filled with a dielectric constant .K.Find the capacitance of the system between AandB.
View SolutionTwo concentric spherical shells of radius R1 and R2(R2>R1) are having uniformly distributed charges Q1 and Q2 respectively. Find out total energy of the system.

View SolutionSpace between the plates of a parallel plate capacitor is filled with a dielectric whose dielectric constant varies with distance as per the relation: K(X)=K0+λX,
(λ= constant, K0= constant, X is perpendicular distance from one plate to a point inside dielectric). The capacitance C1 of this capacitor, would be related to its vacuum capacitance C0 per the relation (d = plate separation):
View SolutionMoment of inertia of a cylindrical shell of mass M, radius R and length L about its geometrical axis would be -
View SolutionThe figure represents two concentric shells of radii R1 and R2 and masses M1 and M2 respectively. The gravitational field intensity at the point A at distance a(R1<a<R2) is

View SolutionA cylindrical wire of radius R has current density varying with distance r form its axis as J(x)=J0(1−r2R2) . The total current through the wire is
View Solution
