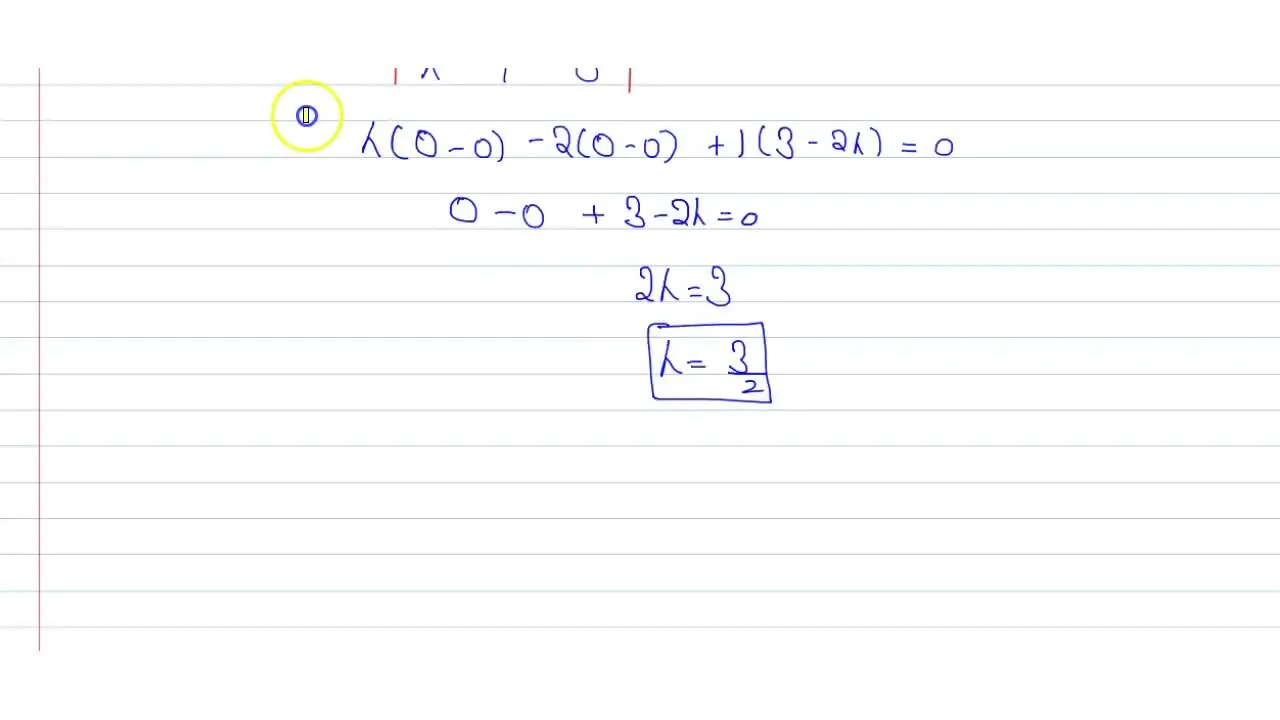For what values of λ are the vectors λˆi+2ˆj+ˆk,3ˆi+2ˆj,λi+j are coplanar.
Step by step video & image solution for For what values of lamda are the vectors lamdahati+2hatj+hatk,3hati+2hatj,lamda i+j are coplanar. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Find the value of λ so that the two vectors 2ˆi+3ˆj−ˆk and −4ˆi−6ˆj+λˆk are parallel
View SolutionShow that the vectors ˆi−ˆj−ˆk,2ˆi+3ˆj+ˆkand7ˆi+3ˆj−4ˆk are coplanar.
View SolutionThe value of λ for which the vectors 3ˆi−6ˆj+ˆkand2ˆi−4ˆj+λˆk parallel, is
View SolutionThe number of real values of a for which the vectors ˆi+2ˆj+ˆk,aˆi+ˆj+2ˆk and ˆi+2ˆj+aˆk are coplanar is
View SolutionThe number of distinct values of λ, for which the vectors −λ2ˆi+ˆj+ˆk,ˆi−λ2ˆj+ˆk and ˆi+ˆj−λ2ˆk are coplanar, is
View SolutionThe number of distinct real values of λ, for which the vectors −λ2ˆi+ˆj+ˆk,ˆi−λ2ˆj+ˆk and ˆi+ˆj−λ2ˆk are coplanar, is
View SolutionFind the value of λso that the two vectors 2ˆi+3ˆj−ˆkand−4ˆi−6ˆj+λˆk are Perpendicular to each other
View SolutionThe vectors λˆi+ˆj+2ˆk,ˆi+λˆj−ˆkand2ˆi−ˆj+λˆk are coplanar, if
View SolutionFind the value of λ such that the vectors →a=2ˆi+λˆj+ˆkand→b=ˆi+2ˆj+3ˆk are orthogonal.
View SolutionLet →a=2ˆi+3ˆj−ˆk and →b=ˆi−2ˆj+3ˆk. Then , the value of λ for which the vector →c=λˆi+ˆj+(2λ−1)ˆk is parallel to the plane containing →a and →b. Is
View Solution