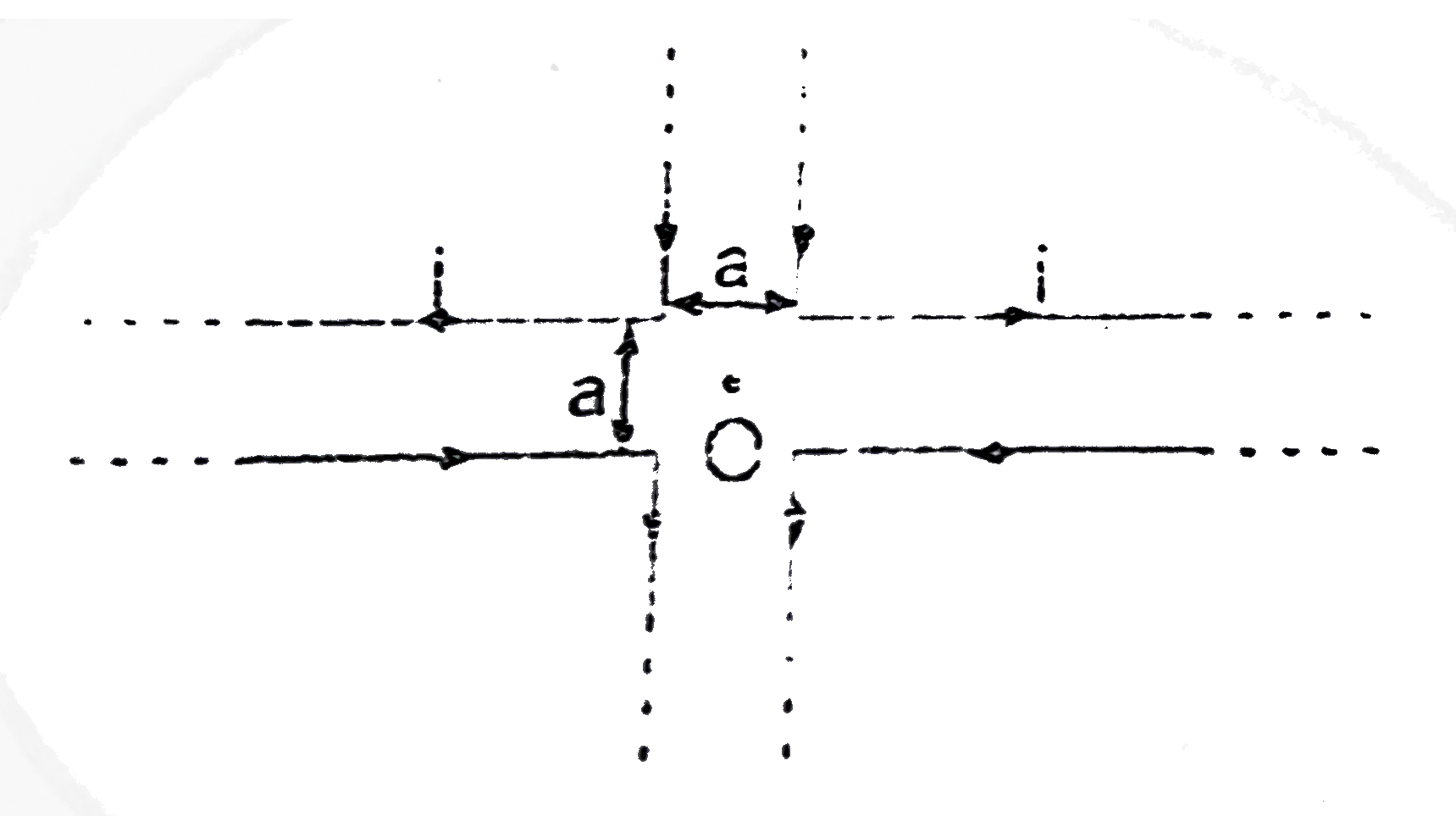
The location of three long wires carrying same current I are x=−a, x=0 and x=+ a respectively as shown in figure. The magnetic field at point (0, 0, a) is
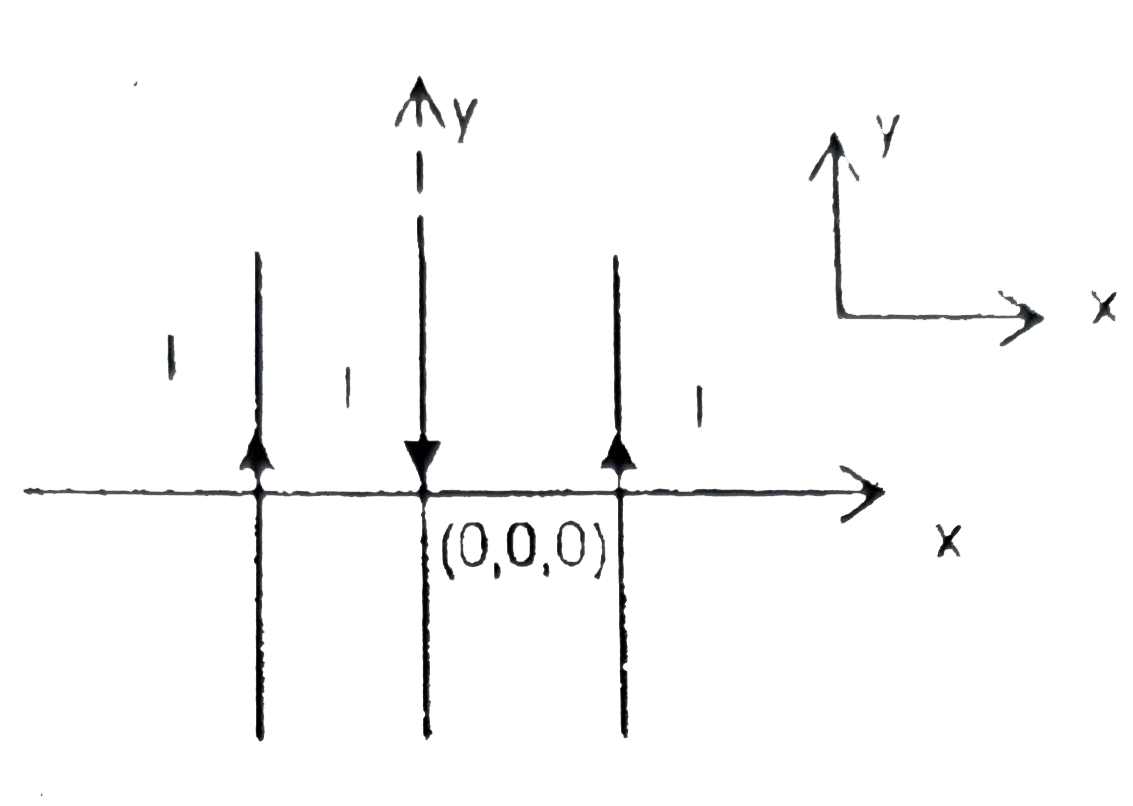
μ0I2πa
μ0I√2πa
μ0I2√2πa
zero
By symmetry, the net field is Zero
The net field at P(0,0,a)
→B=→BA+→B0+→Bc
=μ0I2πa√2{cos45∘ˆi+sin45∘(−ˆk)}+μ0I2πa(−ˆi)+μ0I2πa√2{cos45∘ˆi+sin45∘(ˆk)}]
=μ0I2πa√2√2ˆi+μ0I2πa(−ˆi)
=μ0I2πa[ˆi−ˆi]=0
Similar Questions
A long wire carrying current 'I' is bent into the shape as shown in the figure. The net magnetic field intensity at the centre 'O' is

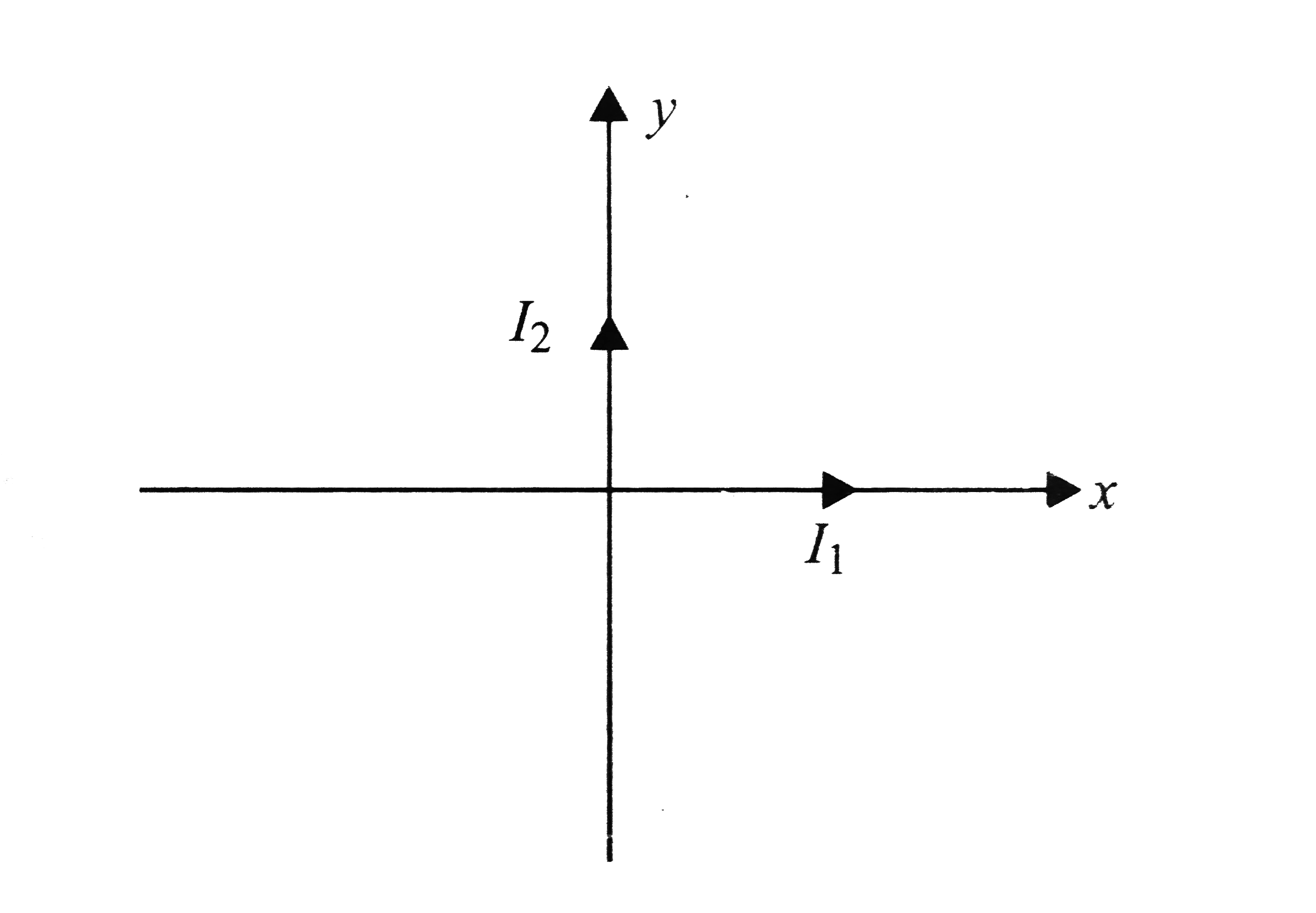
View SolutionTwo thin long wires carry currents I1andI2 along x- and y-axes, respectively, as shown in Fig. Consider the points only in x-y plane.
View SolutionTwo perpendicular infinite wires are carrying current i as shown in the figure. The magnetic field at point P is :
_2019_CBT2_E01_008_Q01.png)
View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is :

View SolutionA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is

View SolutionTwo long straight currents carrying wires having currents I and 2I lie along y-and z-axis, respectively, as shown in Fig. Find →B at a point P(x,0).

View SolutionA coil having N turns carry a current I as shown in the figure. The magnetic field intensity at point P is
View Solutiona straight wire current element is carrying current 100 A as shown in figure. the magnitude of magnetic field at a point P.

View SolutionFour infinitely long L shaped wires, each carrying a current i have been arranged as shown in the figure.Obtain the magnetic field strength at the point O equidistant from all the four corners.
View SolutionA conducting wire carrying current I is shown in the figure. The magnitude of magnetic field at the point P will be

View Solution
