A sperical object of mass 1kg and radius 1 m is falling vertically downward inside a viscous liquid in a gravity free space. At a certain instant the velocity of the sphere is 2 m/s. if the coefficient of viscosity of the liquid is 118πN−S/m2 then velocity of ball will become 0.5 m/s after a tie
In 4 s
2 In 4 s
3 In s
2 In 2 s
The correct Answer is:C
To solve the problem, we need to determine the time it takes for a spherical object to decrease its velocity from 2 m/s to 0.5 m/s while falling through a viscous liquid. We will use the equation for viscous drag and integrate to find the time.
1. Identify the Forces Acting on the Sphere:
The only force acting on the sphere in the viscous liquid is the viscous drag force, which can be expressed as:
Fdrag=−6πηrv
where:
- η is the coefficient of viscosity,
- r is the radius of the sphere,
- v is the velocity of the sphere.
2. Set Up the Equation of Motion:
According to Newton's second law, the net force acting on the sphere is equal to the mass times the acceleration:
mdvdt=−6πηrv
Given that the mass m=1kg, we can rewrite the equation as:
dvdt=−6πηrmv
3. Substitute the Given Values:
We know:
- η=118πN-s/m2
- r=1m
- m=1kg
Substituting these values into the equation gives:
dvdt=−6π(118π)(1)1v=−618v=−13v
4. Separate Variables and Integrate:
We can separate the variables:
dvv=−13dt
Integrating both sides from the initial velocity v0=2m/s to the final velocity v=0.5m/s and from t=0 to t=T:
∫0.521vdv=−13∫T0dt
5. Calculate the Integrals:
The left side becomes:
ln(v)∣∣∣0.52=ln(0.5)−ln(2)=ln(0.52)=ln(14)=−ln(4)
The right side becomes:
−13T
6. Set the Equations Equal:
Setting the two integrals equal gives:
−ln(4)=−13T
7. Solve for Time T:
Rearranging gives:
T=3ln(4)
Final Answer:
The time taken for the sphere to reduce its velocity from 2 m/s to 0.5 m/s is:
T=3ln(4)seconds
The only force acting on the sphere in the viscous liquid is the viscous drag force, which can be expressed as:
Fdrag=−6πηrv
where:
- η is the coefficient of viscosity,
- r is the radius of the sphere,
- v is the velocity of the sphere.
According to Newton's second law, the net force acting on the sphere is equal to the mass times the acceleration:
mdvdt=−6πηrv
Given that the mass m=1kg, we can rewrite the equation as:
dvdt=−6πηrmv
We know:
- η=118πN-s/m2
- r=1m
- m=1kg
dvdt=−6π(118π)(1)1v=−618v=−13v
We can separate the variables:
dvv=−13dt
Integrating both sides from the initial velocity v0=2m/s to the final velocity v=0.5m/s and from t=0 to t=T:
∫0.521vdv=−13∫T0dt
The left side becomes:
ln(v)∣∣∣0.52=ln(0.5)−ln(2)=ln(0.52)=ln(14)=−ln(4)
The right side becomes:
−13T
Setting the two integrals equal gives:
−ln(4)=−13T
Rearranging gives:
T=3ln(4)
The time taken for the sphere to reduce its velocity from 2 m/s to 0.5 m/s is:
T=3ln(4)seconds
Similar Questions
A sphere of mass m and radius r is projected in a gravity free space with speed v. If the coefficient of viscosity of the medium in which it moves is 1/6π Pa s, the distance travelled by the body before it stops is :
View SolutionAn object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
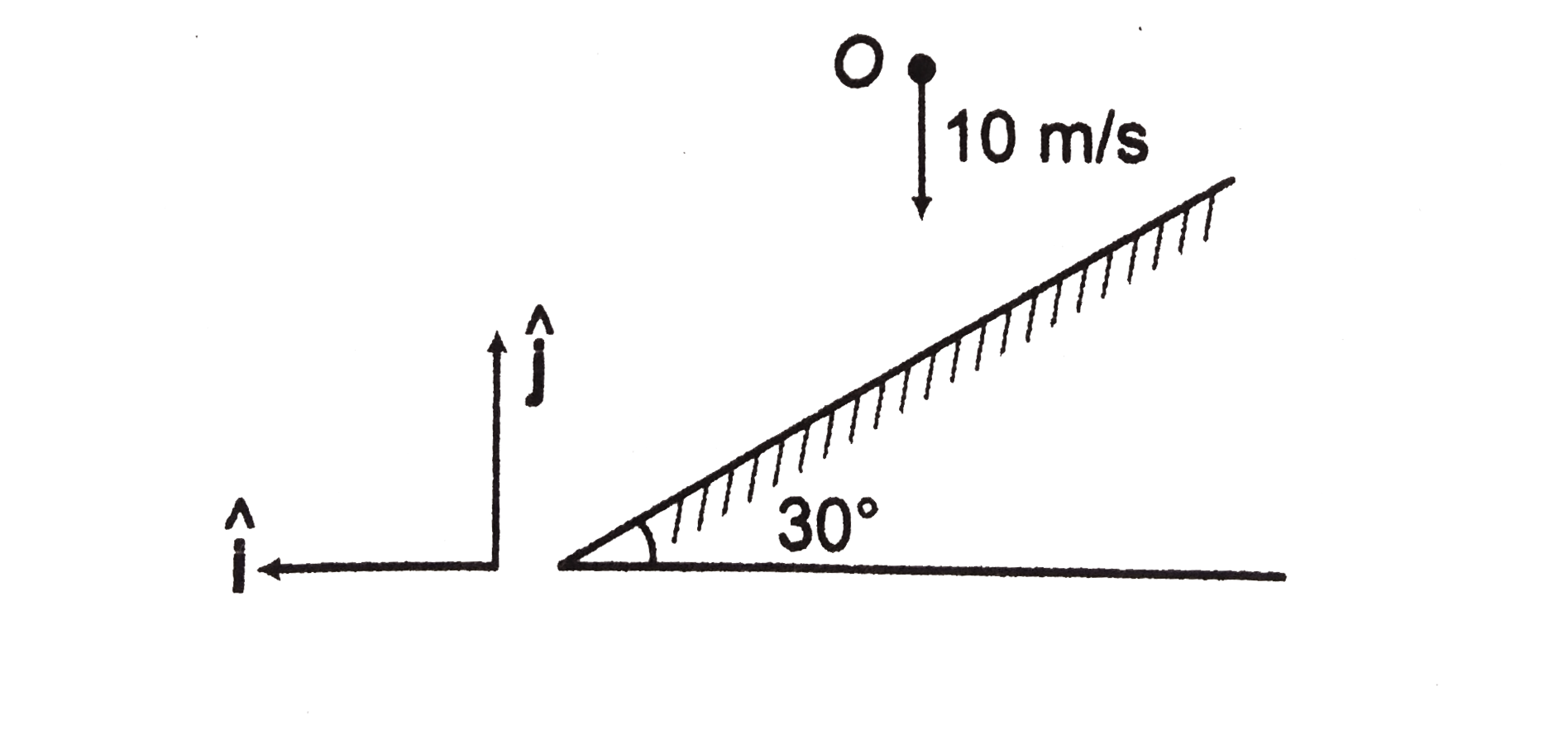
View SolutionRain is falling vertically downward with velocity 4m/s. A man is moving horizontally with velocity 3m/s, the velocity of rain with respect to man is
View SolutionRain is falling vertically downwards with a velocity of 2m//s. A person is runing to the North with a velocity of 2√3m/s. Find out the velocity and direction of rain as appeared to the person.
View SolutionA sphere of mass m and radius r is projected in a gravity free space with speed v. If coefficient of viscosity of the medium in which it moves is 16π , the distance travelled by the body before it stops is
View SolutionA sphere of mass m and radius r is projected in a gravity free space with speed v. If coefficient of viscosity of the medium in which it moves is 16π , the distance travelled by the body before it stops is
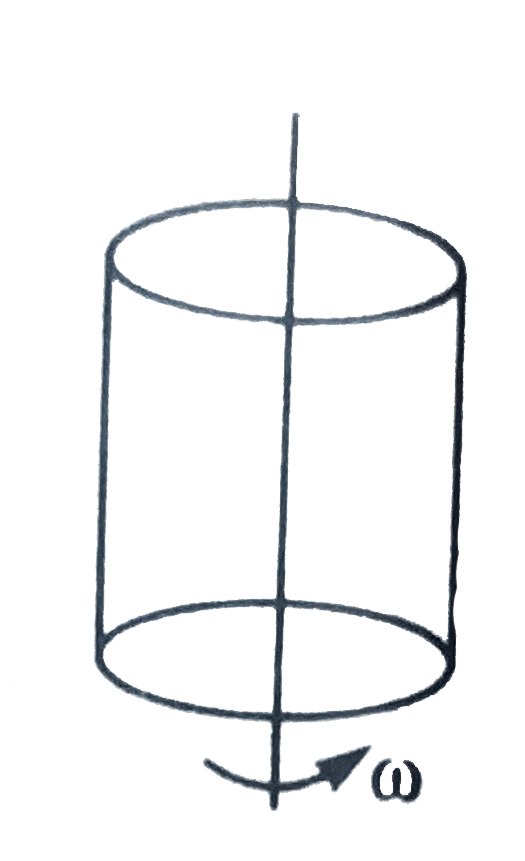
View SolutionAn insect of mass m=3kg is inside a vertical drum of radius 2m that is rotating with an angular velocity of 5rads−1 . The insect doesn't fall off. Then, the minimum coefficient of friction required is
View SolutionA glass of radius 10−3 and density 2000kgm−3 fall in a jar filled with oil of density 800kgm−3 .The terminal velocity is found to be 1 cm/s. Calculate the coefficient of viscosity of oil
View SolutionA disc of mass 1 kg and radius 0.1 m is rotating with angular velocity 20 rad/s. What is angular velocity (in rad/s) if a mass of 0.5 kg is put on the circumference of the disc ?
View SolutionA ring of mass 1kg and radius 1m is moving with a velocity of 1m/s by rolling on a frictionless inclined plane. The total kinetic energy of the ring is
View Solution
