The total No. of isomers possible for the complex [Co(en)2Cl2] is :-
2
3
4
5
The correct Answer is:B
To determine the total number of isomers for the complex (Co(en)2Cl2), we can follow these steps:
Step 1: Identify the Coordination Number and Geometry
The complex (Co(en)2Cl2) has a coordination number of 6 because it contains two bidentate ethylenediamine (en) ligands and two monodentate chloride (Cl) ligands. The geometry of such a complex is octahedral.
Hint: Remember that the coordination number indicates how many ligand atoms are bonded to the central metal atom.
Step 2: Determine Possible Geometrical Isomers
In an octahedral complex, we can have geometrical isomers, specifically cis and trans forms.
- Cis Isomer: In the cis configuration, the two ethylenediamine ligands are adjacent to each other.
- Trans Isomer: In the trans configuration, the two ethylenediamine ligands are opposite each other.
Hint: Visualize the octahedral structure to help differentiate between cis and trans configurations.
Step 3: Analyze Optical Isomerism
The cis isomer can exhibit optical isomerism because it lacks a plane of symmetry. This means that the cis isomer can exist in two forms: the D (dextrorotatory) form and the L (levorotatory) form.
- Optical Isomers of Cis: The cis isomer has two optical isomers (D and L).
- Optical Isomers of Trans: The trans isomer does not have optical isomers because it has a plane of symmetry.
Hint: Remember that optical isomers are non-superimposable mirror images and require the absence of symmetry.
Step 4: Count Total Isomers
Now, we can summarize the isomers:
- Cis Isomer: 2 (D and L forms)
- Trans Isomer: 1
Thus, the total number of isomers is:
Total Isomers=2 (cis)+1 (trans)=3
Hint: Keep track of both geometrical and optical isomers to ensure you count all possible variations.
Conclusion
The total number of isomers possible for the complex (Co(en)2Cl2) is 3.
Final Answer: 3
The complex (Co(en)2Cl2) has a coordination number of 6 because it contains two bidentate ethylenediamine (en) ligands and two monodentate chloride (Cl) ligands. The geometry of such a complex is octahedral.
In an octahedral complex, we can have geometrical isomers, specifically cis and trans forms.
- Trans Isomer: In the trans configuration, the two ethylenediamine ligands are opposite each other.
The cis isomer can exhibit optical isomerism because it lacks a plane of symmetry. This means that the cis isomer can exist in two forms: the D (dextrorotatory) form and the L (levorotatory) form.
- Optical Isomers of Trans: The trans isomer does not have optical isomers because it has a plane of symmetry.
Now, we can summarize the isomers:
- Cis Isomer: 2 (D and L forms)
- Trans Isomer: 1
Total Isomers=2 (cis)+1 (trans)=3
The total number of isomers possible for the complex (Co(en)2Cl2) is 3.
Topper's Solved these Questions
- INORGANIC CHEMISTRYBOOK - ALLENCHAPTER - INORGANIC CHEMISTRYEXERCISE - COORDINATION COMPOUNDS & d-BLOCK COMPOUNDS (MATCH THE COLUMN)
2 Videos
- INORGANIC CHEMISTRYBOOK - ALLENCHAPTER - INORGANIC CHEMISTRYEXERCISE - p-BLOCK ELEMENT
80 Videos
- INORGANIC CHEMISTRYBOOK - ALLENCHAPTER - INORGANIC CHEMISTRYEXERCISE - CHEMICAL BONDING
119 Videos
- HYDROGENBOOK - ALLENCHAPTER - HYDROGENEXERCISE - EXERCISE-5
17 Videos
- Ionic EquilibriumBOOK - ALLENCHAPTER - Ionic EquilibriumEXERCISE - All Questions
37 Videos
Similar Questions
The number of geometric isomers possible for the complex [CoL2Cl2]−(L=H2NCH2CH2O−) is
View SolutionHow many isomers are possible for the complex [Co(en)2Cl2](en=ethylene diamine )
View SolutionHow many isomers are possible for the complex ion [Cr(NH3)3Cl3] ?
View SolutionHow many isomers are possible for the complex [Ir(CO)Cl(PPh3)2]?
View Solution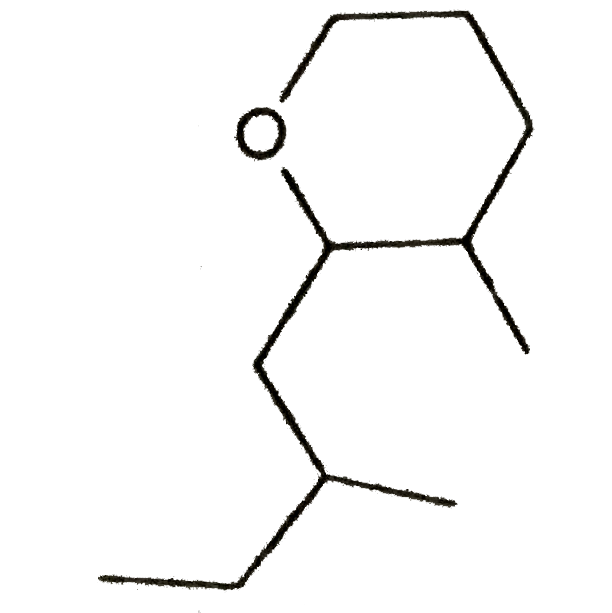
The total number of optical isomers possible for this compound are?
View SolutionHow many total ionization isomers of the complex [CoCl(en)2NO2]SCN are possible ?
View SolutionNumber of possible isomer for the complex [Co(en)2CI2]CI will be: (em = ethylenediamine)
View SolutionThe possible geometric isomers of the complex , [Co(en)Br2Cl2]− is :
View SolutionHow many isomers are possible for the complex ion [Cr(NH3)(OH)2Cl3]2− ?
View Solution(a) For the complex [Fe(H2O)6]3+ , write the hybridization, magnetic character and spin of the complex. (At. Number : Fe =26)
(b) Draw one of the geometrical isomers of the complex [Pt(en)2Cl2]2+ which is optically inactive.
View Solution
