A spherometer has 250 equal divisions marked along the periphery of its disc, and one full rotation of the disadvances by 0.0625 cm on the main scale. What is the least count of the spherometer ?
The correct Answer is:2.5×10−4 cm
To find the least count of the spherometer, we can follow these steps:
Step 1: Understand the given data
We are given:
- The number of equal divisions on the periphery of the disc = 250
- The distance advanced by one full rotation of the disc = 0.0625 cm
Step 2: Use the formula for least count
The least count (LC) of an instrument can be calculated using the formula:
Least Count=Value of one complete rotationNumber of divisions
Step 3: Substitute the values into the formula
Substituting the values we have:
Least Count=0.0625cm250
Step 4: Perform the division
Now, we perform the division:
Least Count=0.0625250
To simplify this, we can convert 0.0625 into a fraction:
0.0625=62510000
Now we can rewrite the least count:
Least Count=625/10000250=62510000×250
Calculating 10000×250:
10000×250=2500000
Thus, we have:
Least Count=6252500000
Step 5: Simplify the fraction
Now we simplify the fraction:
625÷625=1and2500000÷625=4000
So,
Least Count=14000cm
Step 6: Convert to scientific notation
Finally, we can express this in scientific notation:
Least Count=2.5×10−4cm
Final Answer
The least count of the spherometer is:
Least Count=2.5×10−4cm
---
We are given:
- The number of equal divisions on the periphery of the disc = 250
- The distance advanced by one full rotation of the disc = 0.0625 cm
The least count (LC) of an instrument can be calculated using the formula:
Least Count=Value of one complete rotationNumber of divisions
Substituting the values we have:
Least Count=0.0625cm250
Now, we perform the division:
Least Count=0.0625250
0.0625=62510000
Now we can rewrite the least count:
Least Count=625/10000250=62510000×250
10000×250=2500000
Thus, we have:
Least Count=6252500000
Now we simplify the fraction:
625÷625=1and2500000÷625=4000
So,
Least Count=14000cm
Finally, we can express this in scientific notation:
Least Count=2.5×10−4cm
The least count of the spherometer is:
Least Count=2.5×10−4cm
Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Book:ALLENChapter:PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Exercise:EXERCISE-I UNIT
Explore 12 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Book:ALLENChapter:PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Exercise:EXERCISE-I DIMENSIONS
Explore 19 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Book:ALLENChapter:PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Exercise:BEGINNER S BOX-6
Explore 6 Videos
- MISCELLANEOUSBook:ALLENChapter:MISCELLANEOUSExercise:Question
Explore 1 Video
- SEMICONDUCTORSBook:ALLENChapter:SEMICONDUCTORSExercise:Part-3(Exercise-4)
Explore 51 Videos
Similar Questions
A spherometer has 100 equal divisions marked along the periphery of its disc, and one full rotation of the disc advances on the main scale by 0.01cm. Find the least count of the system.
View SolutionIf the number of divisions on the circular scale is 100 and number of full rotations given to screw is 8 and distance moved by the screw is 4 mm, then what will be least count of the screw gauge ?
View SolutionThe figure shows the variation of energy with the orbit radius of a body in circular planetary motion.
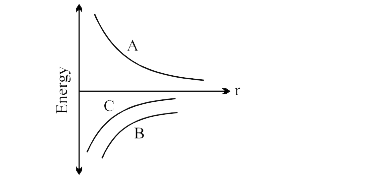
Find the correct statement about the curves A, B and C
View SolutionIn an instrument, there are 25 divisions on the vernier scale which have length of 24 divisions of the main scale. 1 cm on main scale is divided in 20 equal parts. Find the least count.
View SolutionA vernier callipers has 20 divisions on the vernier scale which coincide with 19 divisions on the main scale. The least count of the instrument is 0.1 mm. The main scale divisions are of
View SolutionOne cm on the main scale of vernier callipers is divided into ten equal parts. If 20 division of vernier scale coincide with 8 small divisions of the main scale. What will be the least count of callipers ?
View SolutionIn a vernier callipers, one main scale division is x cm and n divisions of the vernier scale coincide with (n-1) divisions of the main scale. The least count (in cm) of the callipers is :-
View SolutionA microsocpe is provided with a main scale graduated with 20 divisions in 1 cm and a vernier scale with 50 divisions on it of length same as of 49 divisions of main scale. Find the least count of the microscope.
View SolutionA stop watch has 10 divisions graduated between the 0 and 5 s marks. What is its least count?
View SolutionA vernier has 10 divisions and they are equal to 9 divisions of main scale in length. If the main scale is calibrated in mm, what is its least count?
View Solution