It is known that the time of revolution T of a satellite around the earth depends on the universal gravitational constant G, the mass of the earth M, and the radius of the circular orbit R. Obtain an expression for T using dimensional analysis.
To derive the expression for the time of revolution T of a satellite around the Earth using dimensional analysis, we will follow these steps:
Step 1: Identify the variables
We know that the time period T depends on:
- The universal gravitational constant G
- The mass of the Earth M
- The radius of the circular orbit R
Step 2: Write the relationship
We can express T as a function of G, M, and R:
T=k⋅Ga⋅Mb⋅Rc
where k is a dimensionless constant, and a, b, and c are the powers we need to determine.
Step 3: Write the dimensional formulas
The dimensional formulas for the quantities involved are:
- Time T: (T)=T1
- Gravitational constant G: (G)=M−1L3T−2
- Mass M: (M)=M1
- Radius R: (R)=L1
Step 4: Substitute the dimensional formulas
Substituting the dimensional formulas into our equation gives:
(T)=(G)a⋅(M)b⋅(R)c
This translates to:
(T)=(M−1L3T−2)a⋅(M1)b⋅(L1)c
Expanding this, we have:
(T)=M−a+b⋅L3a+c⋅T−2a
Step 5: Set up the equations
Now we equate the dimensions on both sides:
M0L0T1=M−a+b⋅L3a+c⋅T−2a
From this, we can set up the following equations:
1. For mass: −a+b=0
2. For length: 3a+c=0
3. For time: −2a=1
Step 6: Solve the equations
From the third equation:
−2a=1⟹a=−12
Substituting a into the first equation:
−a+b=0⟹−(−12)+b=0⟹b=−12
Substituting a into the second equation:
3a+c=0⟹3(−12)+c=0⟹c=32
Step 7: Write the final expression
Now substituting the values of a, b, and c back into the relationship for T:
T=k⋅G−12⋅M−12⋅R32
This can be simplified to:
T∝R32√G⋅M
Step 8: Conclusion
Thus, the expression for the time of revolution T of a satellite around the Earth is:
T∝R32√G⋅M
We know that the time period T depends on:
- The universal gravitational constant G
- The mass of the Earth M
- The radius of the circular orbit R
We can express T as a function of G, M, and R:
T=k⋅Ga⋅Mb⋅Rc
where k is a dimensionless constant, and a, b, and c are the powers we need to determine.
The dimensional formulas for the quantities involved are:
- Time T: (T)=T1
- Gravitational constant G: (G)=M−1L3T−2
- Mass M: (M)=M1
- Radius R: (R)=L1
Substituting the dimensional formulas into our equation gives:
(T)=(G)a⋅(M)b⋅(R)c
This translates to:
(T)=(M−1L3T−2)a⋅(M1)b⋅(L1)c
Expanding this, we have:
(T)=M−a+b⋅L3a+c⋅T−2a
Now we equate the dimensions on both sides:
M0L0T1=M−a+b⋅L3a+c⋅T−2a
From this, we can set up the following equations:
1. For mass: −a+b=0
2. For length: 3a+c=0
3. For time: −2a=1
From the third equation:
−2a=1⟹a=−12
Substituting a into the first equation:
−a+b=0⟹−(−12)+b=0⟹b=−12
Substituting a into the second equation:
3a+c=0⟹3(−12)+c=0⟹c=32
Now substituting the values of a, b, and c back into the relationship for T:
T=k⋅G−12⋅M−12⋅R32
This can be simplified to:
T∝R32√G⋅M
Thus, the expression for the time of revolution T of a satellite around the Earth is:
T∝R32√G⋅M
Topper's Solved these Questions
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLENCHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - BEGINNER S BOX-1
2 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLENCHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - BEGINNER S BOX-2
4 Videos
- MISCELLANEOUSBOOK - ALLENCHAPTER - MISCELLANEOUSEXERCISE - Question
1 Video
- SEMICONDUCTORSBOOK - ALLENCHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
51 Videos
Similar Questions
The escape velocity of a sphere of mass m is given by (G= univesal gravitational constant, Me= mass of the earth and Re= radius of the earth)
View SolutionThe acceleration due to gravity at a piece depends on the mass of the earth M, radius of the earth R and the gravitational constant G. Derive an expression for g?
View Solution
Knowledge Check
Match the Column I with Column II
For a satellite in circular orbit
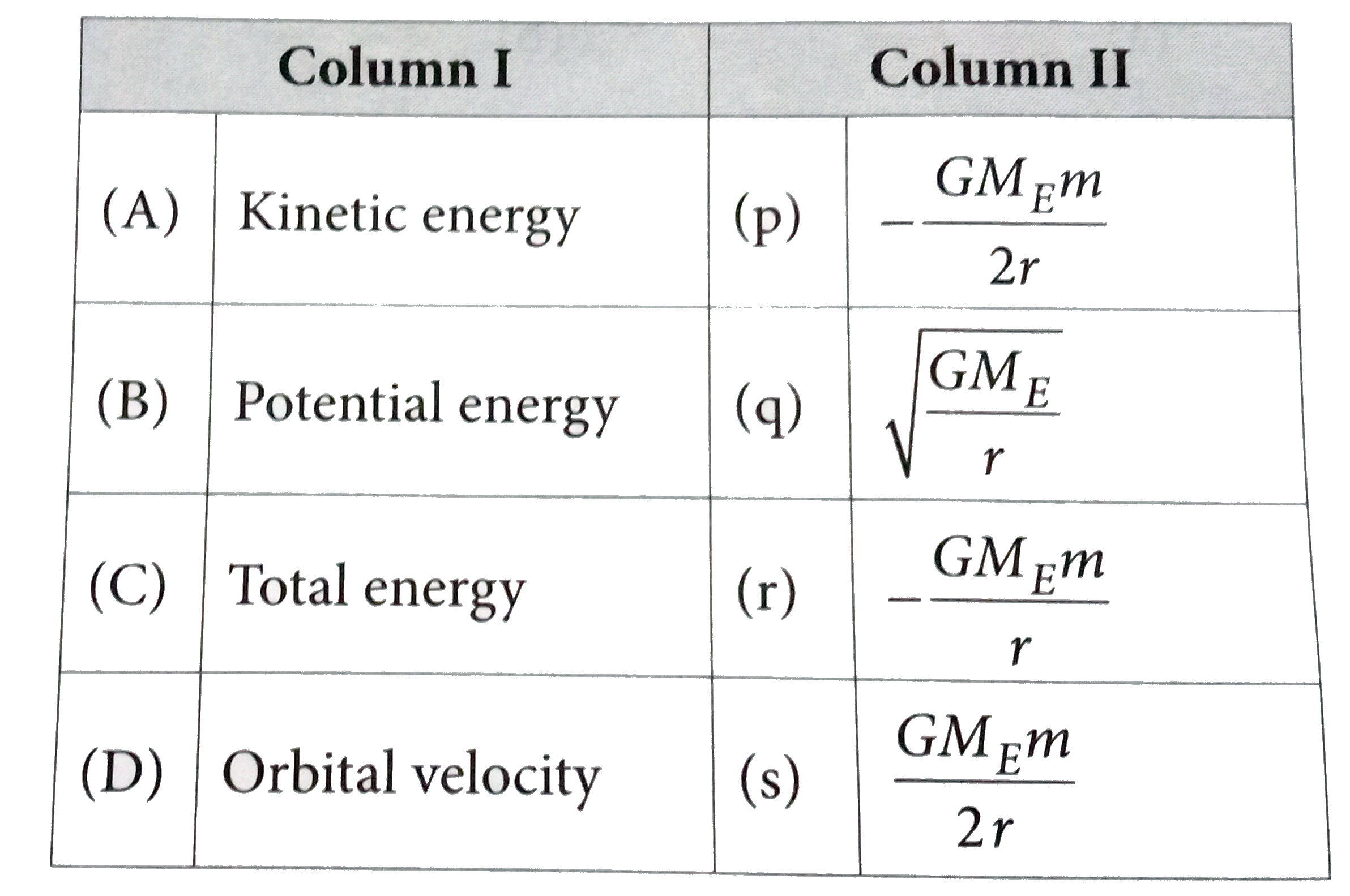
(where ME is the mass of the earth , m is the mass of the satellite and r is the radius of the orbit )AA-r, B-s,C-q,D-pBA-q,B-p,C-r,D-sCA-p,B-q,C-s,D-rDA-s,B-r,C-p,D-qA satellite is orbiting the earth in a circular orbit of radius r. Its
AKnetic enegy varies as rBangular momentum varies as 1√rClinear momentum varies as 1rDfrequency of revolution varies as 1r3/2
Length of a year on a planet is the duration in which it completes one revolution around the sun. Assume path of the planet known as orbit to be circular with sun at the centre. The length T of a year of a planet orbiting around the sun in circular orbit depends on universal gravitational constant G, mass ms of the sun and radius r of the orbit. if T∝Gambsrc find value of a+b+2c.
View SolutionThe escape velocity of a body from the surface of the earth depends upon (i) the mass of the earth M, (ii) The radius of the eath R, and (iii) the gravitational constnat G. Show that v=k√GMR, using the dimensional analysis.
View SolutionThe period of revolution of a satellite depends upon the radius of the orbit, the mass of the planet and the gravitational constant. Prove that the square of the period varies as the cube of the radius.
View SolutionA satellite moves around the earth in a circular orbit with speed v. If m is the mass of the satellite, its total energy is
View SolutionA satellite of mass m goes round the earth along a circular path of radius r. Let mE be the mass of the earth and RE its radius then the linear speed of the satellite depends on.
View SolutionTwo satellites are in the parking orbits around the earth. Mass of one is 5 times that of the other. The ratio of their periods of revolution is
View Solution
