A man walks for some time 't' with velocity(v) due east. Then he walks for same time 't' with velocity (v) due north. The average velocity of the man is:-
2v20g
v20g
v202g
v204g
The correct Answer is:D
To find the average velocity of a man who walks due east and then due north, we can follow these steps:
1. Identify the Directions and Velocities:
- The man walks due east with velocity v for time t.
- Then, he walks due north with the same velocity v for the same time t.
2. Calculate the Displacement for Each Segment:
- For the first segment (due east):
Displacement1=v⋅t(in the east direction)
- For the second segment (due north):
Displacement2=v⋅t(in the north direction)
3. Express the Displacements in Vector Form:
- The displacement vector for the eastward movement can be represented as:
→d1=vt^i
- The displacement vector for the northward movement can be represented as:
→d2=vt^j
4. Calculate the Total Displacement:
- The total displacement vector →D is the vector sum of the two displacements:
→D=→d1+→d2=vt^i+vt^j
5. Simplify the Total Displacement:
- Factor out vt:
→D=vt(^i+^j)
6. Calculate the Magnitude of the Total Displacement:
- The magnitude of the total displacement D can be calculated using the Pythagorean theorem:
D=√(vt)2+(vt)2=√2(vt)2=vt√2
7. Calculate the Total Time:
- The total time T taken for both segments is:
T=t+t=2t
8. Calculate the Average Velocity:
- The average velocity →Vavg is given by the formula:
→Vavg=Total DisplacementTotal Time=vt√22t
- Simplifying this gives:
→Vavg=v√22
9. Final Result:
- The average velocity of the man is:
→Vavg=v√2(in the direction of ^i+^j)
- The man walks due east with velocity v for time t.
- Then, he walks due north with the same velocity v for the same time t.
- For the first segment (due east):
Displacement1=v⋅t(in the east direction)
- For the second segment (due north):
Displacement2=v⋅t(in the north direction)
- The displacement vector for the eastward movement can be represented as:
→d1=vt^i
- The displacement vector for the northward movement can be represented as:
→d2=vt^j
- The total displacement vector →D is the vector sum of the two displacements:
→D=→d1+→d2=vt^i+vt^j
- Factor out vt:
→D=vt(^i+^j)
- The magnitude of the total displacement D can be calculated using the Pythagorean theorem:
D=√(vt)2+(vt)2=√2(vt)2=vt√2
- The total time T taken for both segments is:
T=t+t=2t
- The average velocity →Vavg is given by the formula:
→Vavg=Total DisplacementTotal Time=vt√22t
- Simplifying this gives:
→Vavg=v√22
- The average velocity of the man is:
→Vavg=v√2(in the direction of ^i+^j)
Topper's Solved these Questions
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:Comprehension#6
Explore 4 Videos
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:Comprehension#7
Explore 3 Videos
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:Comprehension#4
Explore 3 Videos
- KINEMATICS-2DBook:ALLENChapter:KINEMATICS-2DExercise:Exercise (O-2)
Explore 46 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBook:ALLENChapter:NEWTON'S LAWS OF MOTION & FRICTIONExercise:EXERCISE (JA)
Explore 4 Videos
Similar Questions
A person travels along a straight road for the half time with a velocity V1 and the next half time with a velocity V2. The mean velocity V of the man is
View SolutionA man traversed half the distance with a velocity v0. The remaining part of the distance was covered with velocity v1. For half the time and with velocity v2 for the other half of the time . Find the average speed of the man over the whole time of motion.
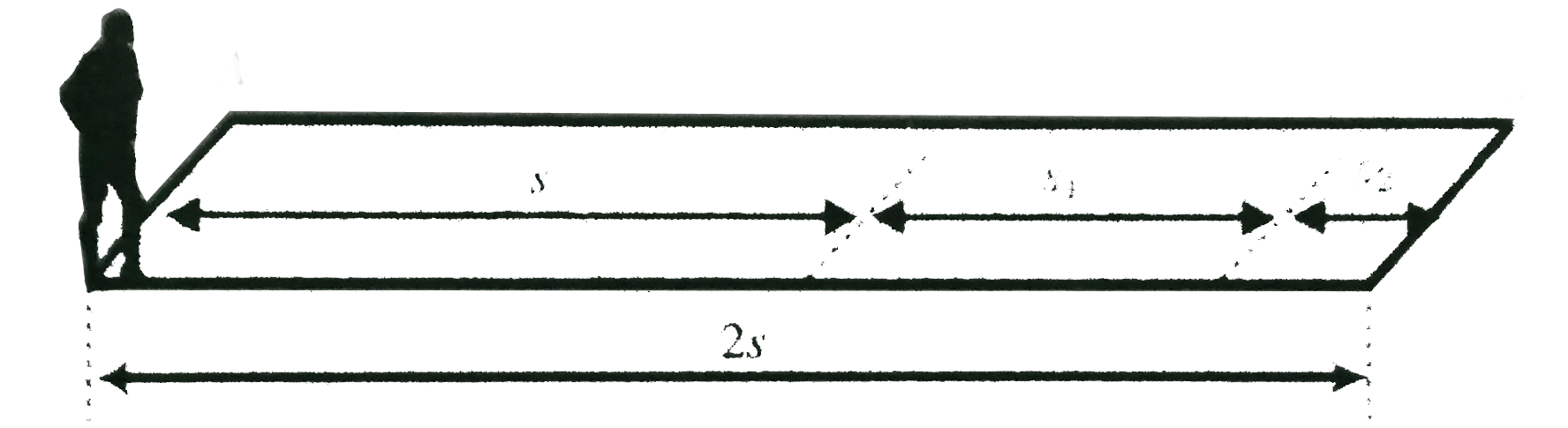 .
.
View Solution
Knowledge Check
A man runs along the straight road for half the distance with velocity v1 and the remaining half distance with velocity v2. Then the average velocity is given by
Av1v2Bv22/v21C(v1+v2)/2D2v1v2/(v1+v2)
A block moves in a straight line with velocity v for time t0. Then, its velocity becomes 2v for next t0 time. Finally, its velocity becomes 3v for time T. If average velocity during the complete journey was 2. 5 v, then find T in terms of t0.
View SolutionA person travelling on a straight line moves with a uniform velocity v1 for some time and with uniform velocity v2 for the next equal time. The average velocity v is given by
View SolutionA particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View SolutionA particle moves in a straight line with uniform acceleration. Its velocity at time t=0 is v1 and at time t=t is v2. The average velocity of the particle in this time interval is v1+v22 . Is this statement true or false?
View SolutionA body is projected vertically up with a velocity v and after some time it returns to the point from which it was projected. The average velocity and average speed of the body for the total time of flight are
View SolutionA body is projected vertically up with a velocity v and after some time it returns to the point from which it was projected. The average velocity and average speed of the body for the total time of flight are
View SolutionA man of mass 70 kg is standing at one end of a stationary, floating barge of mass 210 kg. he then walks to the other end of the barge, a distance of 90 meters. Ignore any frictional effect between the barge and the water.
Q. If the man walks at an average velocity of 8m/s what is the average velocity of the barge?
View Solution