Velocity-time curve for a body projected vertically upwards is with times (s)
v=v0e−S/R
v=v0eS/R
v=v0e−R/S
v=v0eR/S
The correct Answer is:A
Given dvdt=v2r⇒dvds=v2r,−v∫v01vdv=s∫odsr
⇒ln[v0v]=Sr⇒v0v=eS/r
⇒v0=veS/r⇒v=v0e−S/r
Related Playlists
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - EXERCISE-036videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - Assertion-Reason20videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - MOTION IN A PALNEEXERCISE - SOLVED EXAMPLE28videos
MOTION IN A PALNE
- BOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)46videos
KINEMATICS-2D
- BOOK - ALLENCHAPTER - NEWTON'S LAWS OF MOTION & FRICTIONEXERCISE - EXERCISE (JA)4videos
NEWTON'S LAWS OF MOTION & FRICTION
Similar Questions
The escape velocity for a body projected vertically upwards from the surface of the earth is 11.2kms−1. If the body is projected in a direction making an angle 45∘ with the vertical, the escape velocity will be
View SolutionThe escape velocity of a body projected vertically upward from the earth's surface is 11.2 kms−1 . If the body is projected in a direction making 30∘ angle to the vertical, its escape velocity in this case will be
View SolutionWith what velocity a body of mass m be projected vertically upwards so that it may be able to reach a height nR above earth's surface
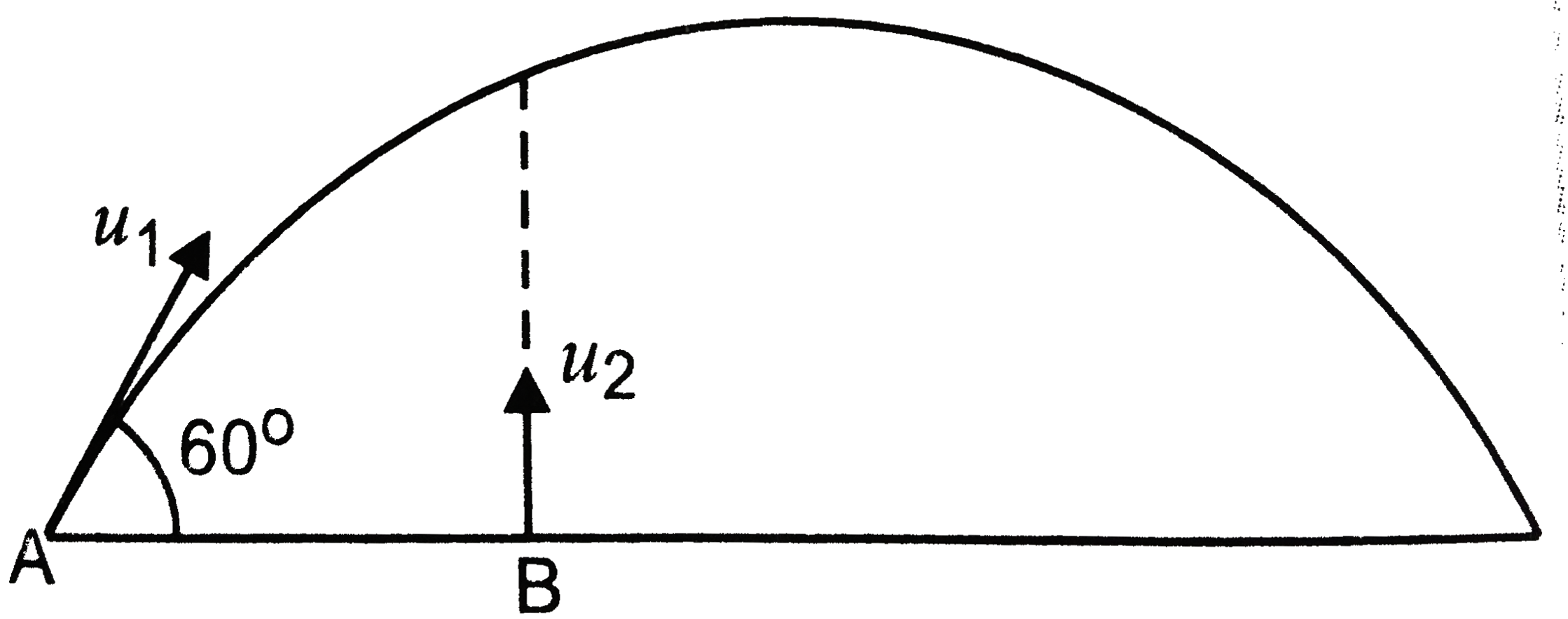
View SolutionA body is projected with the velocity u1 from the point (A) as shown in Fig. 2. (d). 37. At the same time another body is projected vertically upwards with the velocity u2 from the point (B) .What should be the value of u1/u1 for both the bodies to collide ?
 .View Solution
.View SolutionA body is projected with the velocity u1 from the point A as shown in Figure. At the same time another body is projectd vertically upwards with the velocity u2 from the point B. What shold be the value of u1u2 for both the bodies to collide.
 View Solution
View SolutionA particle is projected vertically upwards in vacuum with a speed u.
View SolutionA body is projected vertically upwards wth a velocity u=5m/s. After time t another body is projected vertically upward from the same point with a velocity v=3m/s. If they meet in minimum time duration measured from the projection of first body, then t=kg sec find k (where g is gravitatio acceleration).
View Solution(A):Body projected vertically up or down from the top of a tower with same velocity will reach the ground with same velocity.
(R ):Both the bodies projected vertically up and town will have same displacement and acceleration.View SolutionAir offers a retardation of 6m/s2 to a particle. If it is projected vertically upwards with a speed of 96m/s from ground then the time of flight is : (g=10m/s2)
View SolutionA stone is dropped from a height 300 m and at the same time another stone is projected vertically upwards with a velocity of 100m/sec. Find when and where the two stones meet.
View Solution